Mathematics > SOPHIA Milestone > COLLEGE ALGEBRA UNIT 2 LATEST QUESTIONS AND ANSWERS. GRADED A+. (All)
COLLEGE ALGEBRA UNIT 2 LATEST QUESTIONS AND ANSWERS. GRADED A+.
Document Content and Description Below
UNIT 2 — MILESTONE 2 Score 19/20 You passed this Milestone 19 questions were answered correctly. 1 question was answered incorrectly. 1 Consider the following subset of the real number line H... ow can this set be expressed using inequalities? -3 ≤ x < 1 -3 ≥ x > 1 -3 < x ≤ 1 -3 > x ≥ 1 RATIONALE 5/25/2021 Sophia :: Welcome https://snhu.sophia.org/spcc/college-algebra-3/milestone_take_feedbacks/9199062 2/13 CONCEPT Introduction to Inequalities 2 What is the solution set for the following inequality? 2x + 8x ≥ 15 + 14x + 9 x ≤ -6 x ≤ -0.85 x ≥ -6 x ≥ -0.85 RATIONALE Before solving for x, make sure that each side of the inequality is fully simplified. On the right side, we will add 15 and 9. On the right side, 15 plus 9 is 24. On the left side, we can combine 2x and 8x. 2x plus 8x is 10x. Now we can begin to solve for x by applying inverse operations to both sides of the inequality. First, subtract 14x from both sides. Subtracting 14x from both sides cancels the 14x term on the right side of the inequality, leaving x terms on only the left side. Finally, divide both sides of the inequality by -4. Remember that the sign of the inequality sign changes whenever you multiply or divide by a negative number! The solution to the inequality is x ≤ -6. CONCEPT Solve Linear Inequalities 3 John is driving at a constant speed of 40 miles per hour. How long does it take him to travel 50 miles? An hour and 48 minutes An hour and 15 minutes 5/25/2021 Sophia :: Welcome https://snhu.sophia.org/spcc/college-algebra-3/milestone_take_feedbacks/9199062 3/13 An hour and 8 minutes An hour and 30 minutes RATIONALE To find how long it will take John to travel 50 miles, we can use the distance, rate, time formula and solve for time. Plug in 50 miles for the distance, and 40 miles per hour for the rate, or speed. Once we have plugged in the values, we need to write miles per hour as a fraction: 40 miles over 1 hour. When dividing by a fraction, we can change this into a multiplication problem and multiply by the reciprocal of 40 miles per 1 hour, which would be 1 hour over 40 miles. Rewrite 50 miles as a fraction over 1, and multiply this by the reciprocal of 40 mph. Next, multiply the numerators and denominators of the fractions. Multiplying across the numerator and denominator, produces 50 over 40. The units of miles cancel, so we are left with hours. Finally, divide 50 by 40. It will take John 1.25 hours. However, because we must express our answer in hours and minutes, we must convert 0.25 hours to minutes. Using the conversion factor, 60 minutes to 1 hour, evaluate the fractions by multiplying the numerators and denominators. Multiplying across the numerator and denominator produces 0.25 times 60, or 15. Units of hours cancel, leaving only minutes. It will take John 1 hour and 15 minutes to travel 50 miles at a constant speed of 40 miles per hour. CONCEPT Distance, Rate, and Time 4 The number of employees who will be able to perform a specific task is given by the equation , where N is the number of people who are able to perform the task, and t is time (in days). How long will it take until 100 employees are able to perform the task? 16 days 20 days 4 days 8 days RATIONALE The number of employees who can perform the task is modeled by this equation. To find how long it will take until 100 employees can perform the task, we will substitute this value for N and solve for t. Once N is replaced, we can solve for t. First, divide both sides by 25 to undo 25 multiplied by the square root of t. On the left, 100 divided by 25 is 4. To undo the square root of t on the right, square both sides. On the left, 4 squared is 16. It will take 16 days for 100 employees to be able to perform the task. CONCEPT Solving Multi-step Equations 5 5/25/2021 Sophia :: Welcome https://snhu.sophia.org/spcc/college-algebra-3/milestone_take_feedbacks/9199062 4/13 The value V (in dollars) of an airplane depends on the flight hours x as given by the formula V = 1,800,000 – 250x. After one year, the value of the plane is between $1,200,000 and $1,300,000. Which range for the flight hours does this correspond to? 2000 ≤ x ≤ 2400 2200 ≤ x ≤ 2500 1800 ≤ x ≤ 2100 1500 ≤ x ≤ 1800 RATIONALE The value of the airplane is modeled by this equation. To find the range for the flight hours, construct an inequality with the expression for the plane's value in between the lower and upper values for the plane given in the problem. Solving these kinds of inequalities is very similar to solving equations. Use inverse operations to isolate the variable, but be sure to perform the same operations in all parts of the inequality. First, subtract 1,800,000 from all three parts of the inequality. Subtracting 1,800,000 from all three parts cancels out the 1,800,000 in the middle, leaving only the x term. Next, divide all parts of the inequality by -250 Remember that whenever you multiple or divide an inequality by a negative number, the sign of the inequality symbols change direction. We are now left with only a x term in the middle. However, the inequality symbols are not arranged in standard form, with the smaller number first. This is an equivalent statement from the previous inequality. If you re-write the inequalities in this way, you'll notice the symbols change directions, too. CONCEPT Compound Inequalities 6 Simplify the following expression. ‐4(x – 2) + 3(x + 2) -x – 2 7x - 6 7x + 4 -x + 14 RATIONALE Notice that there is a factor of -4 multiplied by (x – 2). Distribute the -4 by multiplying it by each individual term in (x – 2). -4 times x is -4x, and -4 times -2 is 8. We see that -4(x – 2) is equal to -4x + 8. Next, we must do the same with 3(x + 2). 3 times x is 3x, and 3 times 2 is 6. We see that 3(x + 2) is equal to 3x + 6. Now we can combine like terms to simplify. We can combine -4x and 3x to get -x. We can also combine 8 and 6 to get 14. The simplified expression is -x + 14. CONCEPT Properties in Algebraic Expressions 7 Dan paid $498.09 (including sales tax) for a laptop computer. The sales tax in his state is 7%. 5/25/2021 Sophia :: Welcome https://snhu.sophia.org/spcc/college-algebra-3/milestone_take_feedbacks/9199062 5/13 What was the price of the laptop before the sales tax was added? $472.00 $469.75 $465.50 $463.25 RATIONALE To calculate the price of an item with tax included, multiply the original price of the item by (1 + r), where r is the percent tax expressed as a decimal. The price of the laptop with tax included is $498.09 and the percent tax, r, is 7%, or 0.07 when written as a decimal. Substitute these values in the equation and solve for the original price. Once the known values are substituted into the formula, evaluate the parentheses by adding 1 and 0.07. 1 plus 0.07 equals 1.07. Next, undo the multiplication on the right side by dividing both sides by 1.07. $498.09 divided by 1.07 is $465.50. The price of the laptop without sales tax is $465.50 CONCEPT Solving Problems involving Percents 8 Simplify the following expression. ‐2 + 5(a – 2b + 1) – 3(8 – 2a + b) 3a – b – 25 11a – 3b – 21 11a – 5b – 25 3a – 9b – 21 RATIONALE To simplify this expression, we must use the Distributive Property to distribute the number outside parentheses by multiplying each term inside. There are two instances here. First, we will distribute 5 into (a – 2b + 1). 5(a – 2b + 1) is equivalent to 5a – 10b + 5. Next we have to distribute -3 into (8 – 2a + b). Notice the negative sign in front of 3. -3(8 – 2a + b) is equivalent to -24 + 6a – 3b. Now we have to combine like terms. The terms 5a and 6a combine to 11a, the terms -10b and-3b combine to -13b, and the constant terms -2, 5, and -24 combine to -21. The expression ‐2 + 5 (a – 2b + 1) – 3(8 – 2a + b) is equivalent to 11a – 3b – 21. CONCEPT Terms and Factors in Algebraic Expressions 9 Which of the following is equivalent to ? 5/25/2021 Sophia :: Welcome https://snhu.sophia.org/spcc/college-algebra-3/milestone_take_feedbacks/9199062 6/13 RATIONALE Radical expressions can easily be added and subtracted similar to combining like terms, if there is the same number underneath the radical. If they are different, use the Product Property of Radicals to simplify if possible. Let's consider the first term, . is already in its simplest form, since 2 is a prime number that cannot be broken down any further. Let's simplify the next term, . can be written as because 4 times 2 is 8. We can simplify the square root of 4 further. The square root of 4 is 2, so the expression becomes when fully evaluated. Let's consider the next radical, . can be written as because 9 times 2 is 18. We can simplify the square root of 9 further. The square root of 9 is 3, so the expression becomes when fully evaluated. Now we can rewrite the original expression with the newly evaluated terms. can be rewritten as . We have simplified the expression to radical expressions with the same number underneath the radical sign. Lastly, we can combine all of the coefficients in front of the radical sign. 7 minus 6 plus 3 equals 4. The expression is equivalent to . CONCEPT Adding and Subtracting Radical Expressions 10 Laura opened a deposit account. In the first month, she made an initial deposit of $2500, and pla [Show More]
Last updated: 1 year ago
Preview 1 out of 13 pages

Reviews( 0 )
Recommended For You
Mathematics> SOPHIA Milestone > LATEST SOFIA LEARNING. GRADED A,. MATHEMATICS QUESTIONS AND ANSWERS. 2021/2022. DOWNLOAD TO SCORE A+ (All)
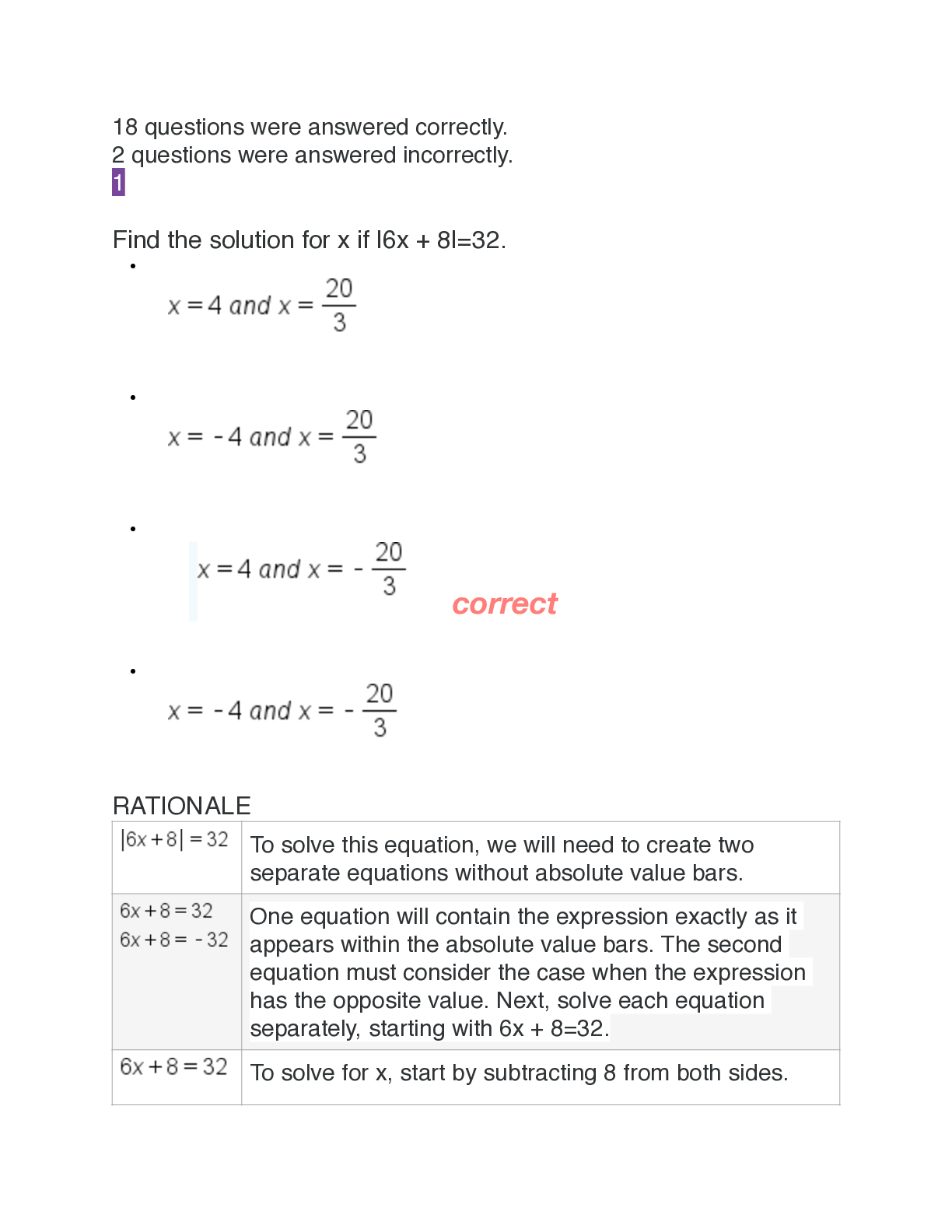
LATEST SOFIA LEARNING. GRADED A,. MATHEMATICS QUESTIONS AND ANSWERS. 2021/2022. DOWNLOAD TO SCORE A+
18 questions were answered correctly. 2 questions were answered incorrectly. 1 Find the solution for x if |6x + 8|=32. • • • correct • RATIONALE To solve this equation, we will need to crea...
By A grade master , Uploaded: Apr 07, 2022
$8
Mathematics> SOPHIA Milestone > College Algebra Milestone 5 - All Answers ACCURATE QUESTIONS AND ANSWERS. GRADED A. 100% PASS RATE (All)

College Algebra Milestone 5 - All Answers ACCURATE QUESTIONS AND ANSWERS. GRADED A. 100% PASS RATE
You passed this Milestone 21 questions were answered correctly. 1 question was answered incorrectly. 1 Consider the function . What are the domain and range of this function? • • correct •...
By A grade master , Uploaded: Apr 07, 2022
$8
Communication> SOPHIA Milestone > Visual Communication - Unit 1 Milestone 1.docx. GRADED A+. ALL QUESTIONS AND ANSWERS. (All)

Visual Communication - Unit 1 Milestone 1.docx. GRADED A+. ALL QUESTIONS AND ANSWERS.
Which of the following best defines space? Space consists of lines. Space surrounds form and any other positive elements. Space is made up of neutral colors. Which question reflects the c...
By A grade master , Uploaded: Mar 31, 2022
$8
Computer Science> SOPHIA Milestone > Unit 4 - Milestone 4.docx. Questions and answers. Rated A Masterpiece. 100% pass rate. (All)

Unit 4 - Milestone 4.docx. Questions and answers. Rated A Masterpiece. 100% pass rate.
Zehra works with a software development team. One of his tasks is to define the overall structure of an application and divide it into components to be developed. What is Zehra's role on the softwa...
By A grade master , Uploaded: Mar 29, 2022
$7
Philosophy> SOPHIA Milestone > Intro to Ethics Unit 3.docx. SOFIA MILESTONE QUESTIONS AND ANSWERS. GRADED A+ (All)

Intro to Ethics Unit 3.docx. SOFIA MILESTONE QUESTIONS AND ANSWERS. GRADED A+
Unit 3 Challenge 1 For a utilitarian, which consideration is most important? Answer: The consequences of an action Why is utilitarianism an objectivist or relativist theory? Answer: Utilitarianism...
By A grade master , Uploaded: Mar 29, 2022
$7
Philosophy> SOPHIA Milestone > sophia Ethics Milestone4.docx,. ALL QUESTIONS AND ANSWERS. GRADED A+. (All)

sophia Ethics Milestone4.docx,. ALL QUESTIONS AND ANSWERS. GRADED A+.
Renae has three brothers. Over the course of a weekend, she observed them in different situations with the new neighbors. Her oldest brother, Nick, stepped in front of her when the neighbors’ large...
By A grade master , Uploaded: Mar 29, 2022
$7
*NURSING> SOPHIA Milestone > Sophia Human Biology Milestones 6 (RATED A) Questions And Answers. (All)

Sophia Human Biology Milestones 6 (RATED A) Questions And Answers.
Sophia Human Biology Milestones 6 Sophia Human Biology Milestone 6 1 Being left-handed (represented as l) is a recessive trait. Jim and Suzie are both left-handed. If they have a child, which of the f...
By A+ Solutions , Uploaded: Nov 19, 2021
$10
History> SOPHIA Milestone > Sophia US History II Milestone 1 Final Exam. Questions And Answers. Latest 2020/21 (All)

Sophia US History II Milestone 1 Final Exam. Questions And Answers. Latest 2020/21
Sophia US History II Milestone 1 Final Exam. Questions And Answers. Latest 2020/21.Which statement about populism most closely aligns with historian Lawrence Goodwyn’s interpretation of the term? • I...
By Quality Suppliers , Uploaded: Feb 23, 2021
$10
*NURSING> SOPHIA Milestone > Sophia - US History II - Milestone 2 Final Questions And Answers. Latest 2020/21 (All)
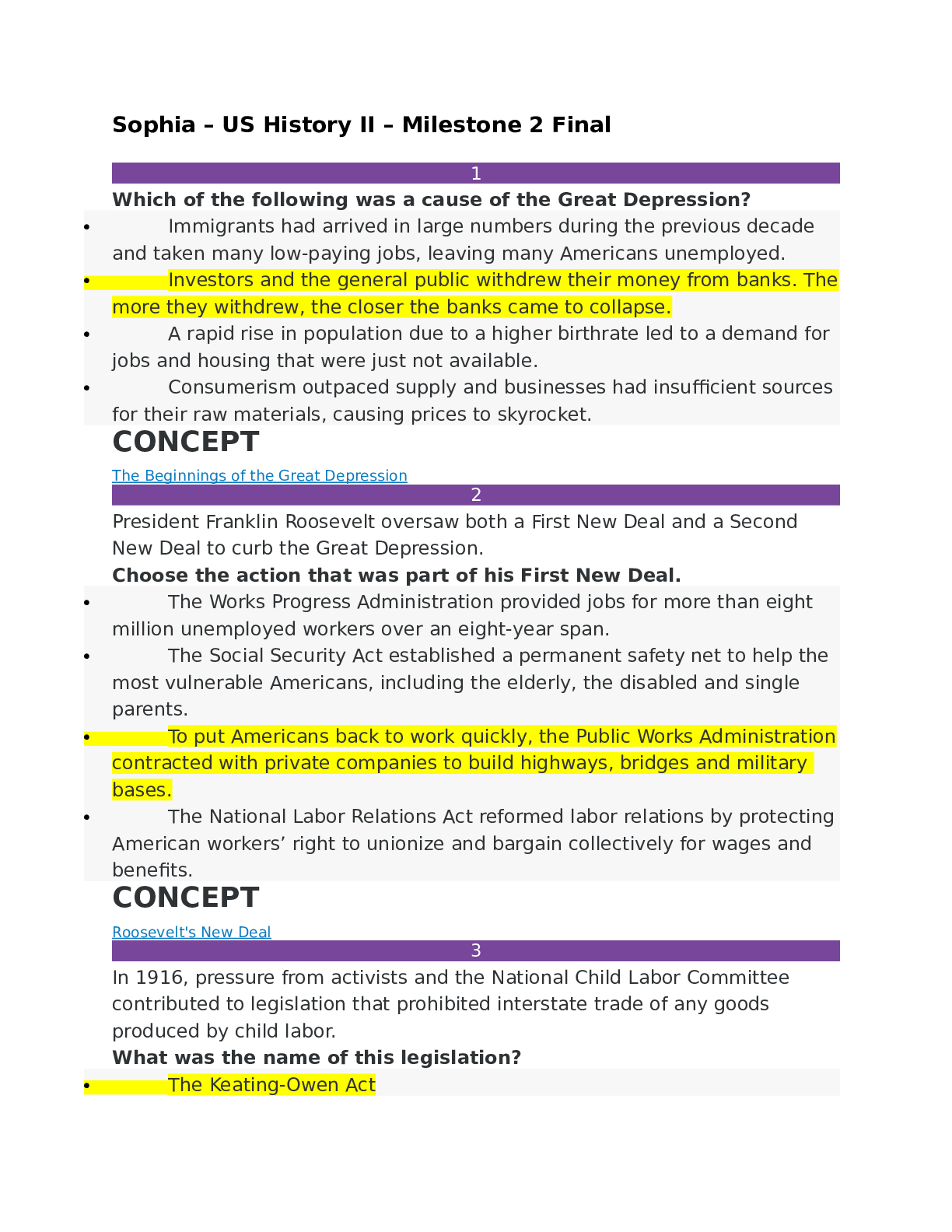
Sophia - US History II - Milestone 2 Final Questions And Answers. Latest 2020/21
Sophia - US History II - Milestone 2 Final Questions And Answers. Latest 2020/21.Which of the following was a cause of the Great Depression? • Immigrants had arrived in large numbers during the previ...
By Quality Suppliers , Uploaded: Feb 23, 2021
$10
History> SOPHIA Milestone > Sophia - US History II - Milestone 3 Final Exam. Questions And Answers. Complete Solution (All)

Sophia - US History II - Milestone 3 Final Exam. Questions And Answers. Complete Solution
Sophia - US History II - Milestone 3 Final Exam. Questions And Answers. Complete Solution.Which of the following was a significant effect of containment policy and the Cold War on the American governm...
By Quality Suppliers , Uploaded: Feb 23, 2021
$11
Document information
Connected school, study & course
About the document
Uploaded On
Apr 07, 2022
Number of pages
13
Written in
Additional information
This document has been written for:
Uploaded
Apr 07, 2022
Downloads
1
Views
256






