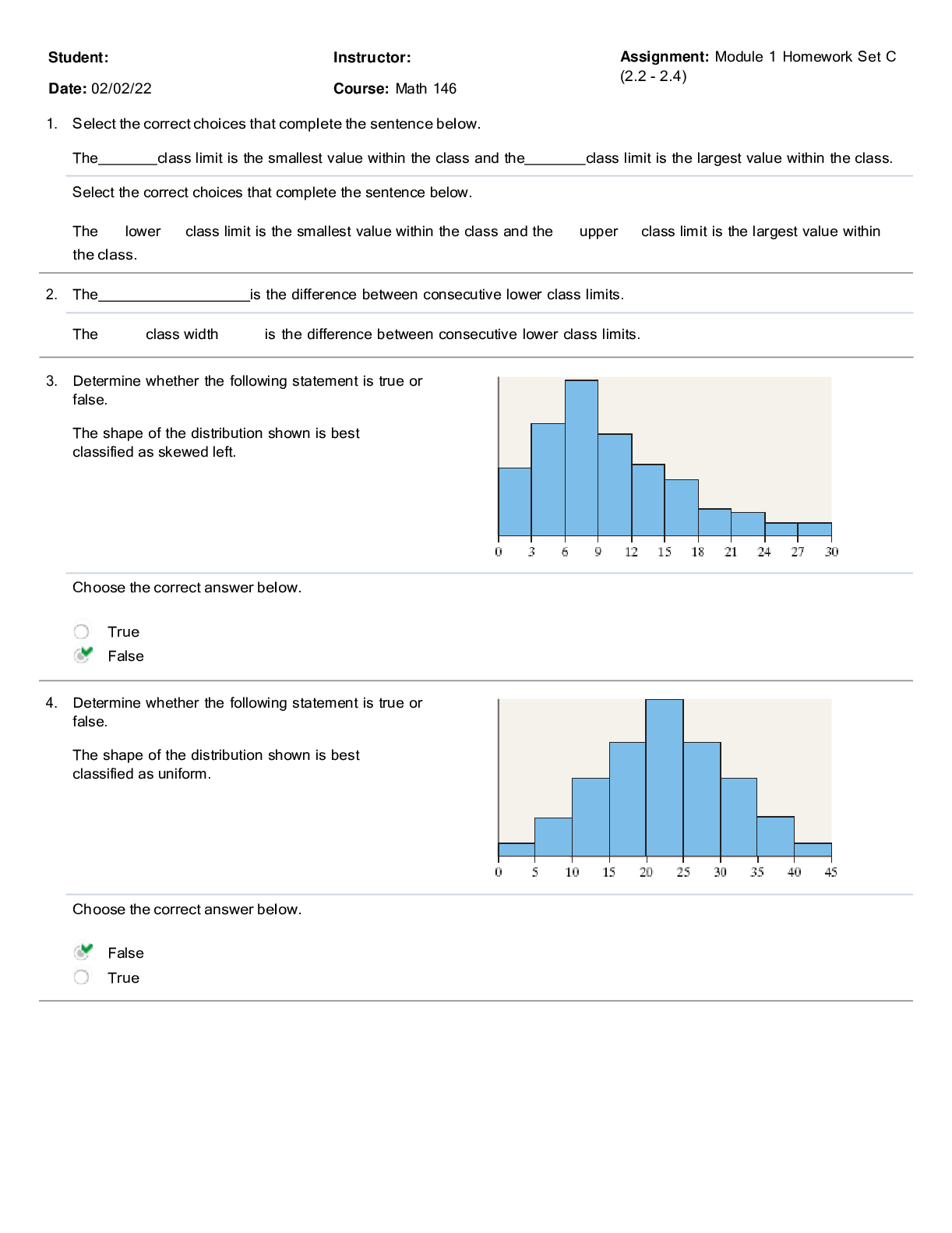
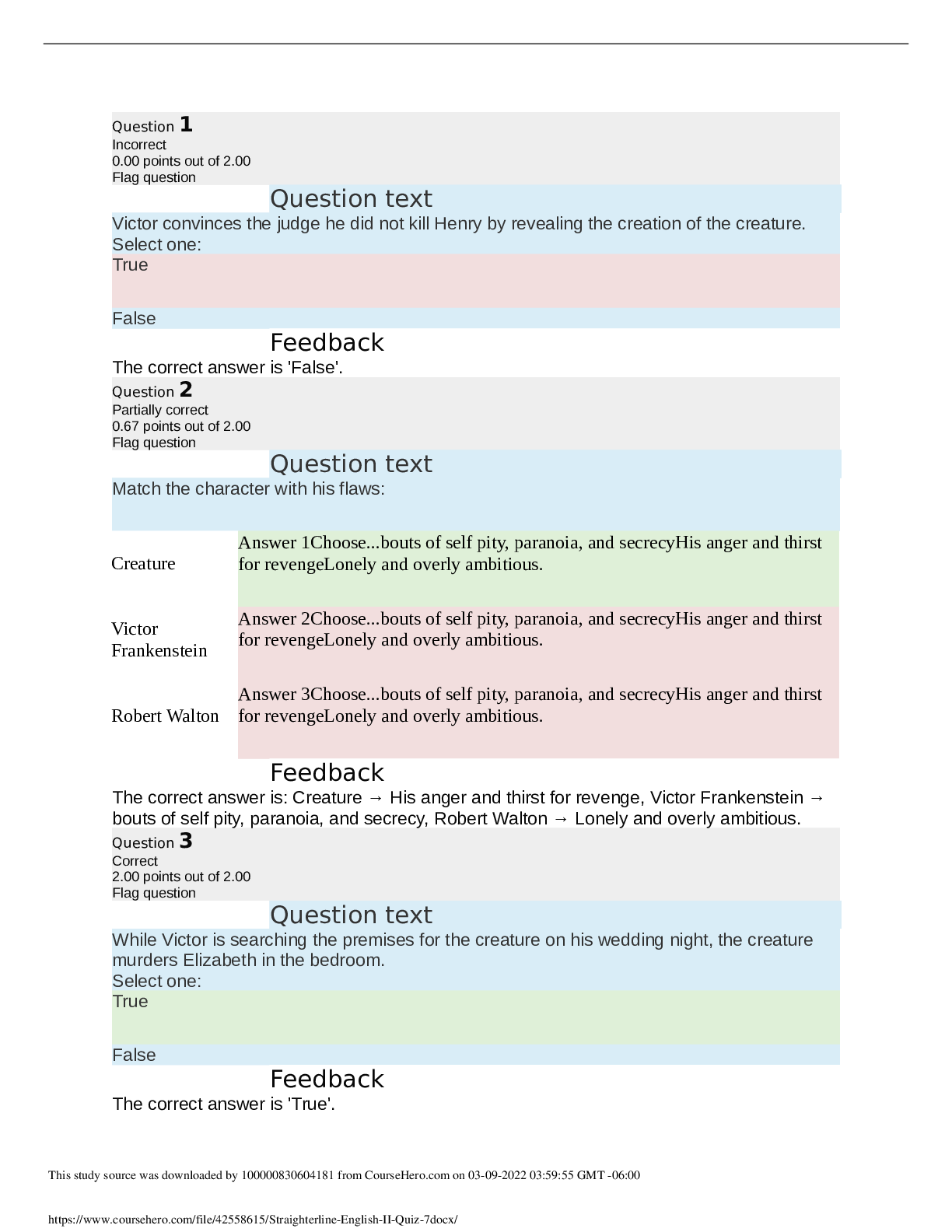
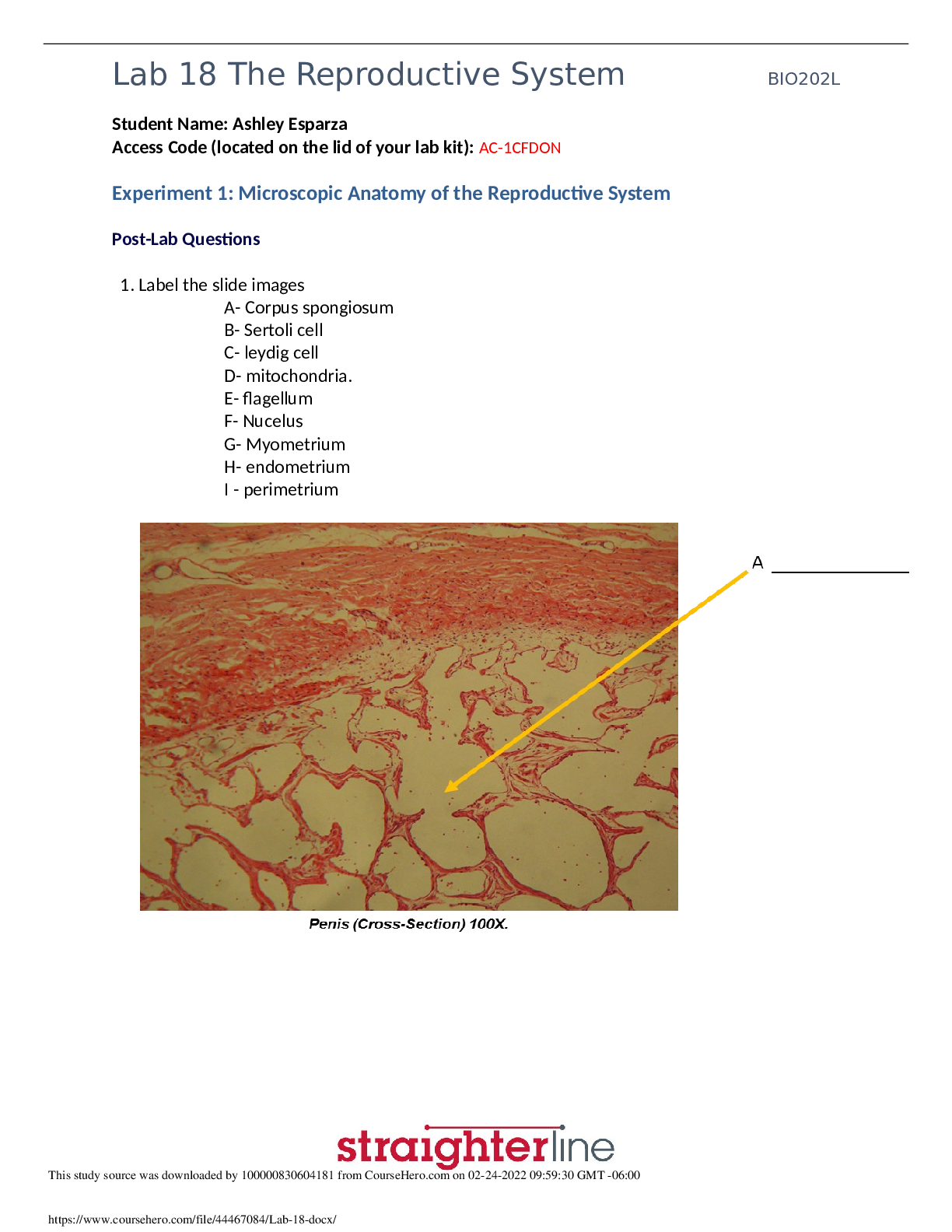
University of Waterloo MATH 207 Fall 2013 Solution

Document Content and Description Below

MATH 207 Fall 2013 Dr. Smith Assignment 4 Total is 50 1. Find an equation of the tangent plane to the given surface at the specified point. a) z = 3(x – 1)2 + 2(y + 3)2 + 7, at (2, – 2, 12) fx... (x,y) = 6(x – 1) and fy(x,y) = 4(y + 3) fx (2, –2) = 6(2 – 1) = 6 and fy(2, –2) = 4(–2 + 3) = 4 z = 6(x – 2) + 4(y + 2) + 12 = 6x + 4y + 8 b) z = y ln x at (1, 4, 0) c) 2 2 x y z e − = at (1, – 1, 1) Six points: 2 each for a, b, c. 2. a) Explain why the function f (x, y) = x + e4 y is differentiable at the point (3,0). Then find the linearization L(x,y) at (3,0), and use it to approximate (3.1, –0.1). y x x e f 2 4 1 + = ∂ ∂ and y y e e x fy 4 4 2 4 + = ∂ ∂ . Both are continuous on their domain, since they are an algebraic combination of continuous functions, and (3,0) is in their domain, since the denominators do not go to 0 there. By Thm. 8, f(x,y) is differentiable at (3,0). L(x,y) = fx(3,0)(x – 3) + fy(3,0)y + f(3,0) = ( 3) 2 14 x − + y + L(3.1, –0.1) = ¼ (3.1 – 3) + (–0.1) + 2 = 1.925 b) Explain why the function f(x,y) = sin(2x + 3y) is differentiable at the point (–3,2). Then find the linearization L(x,y) at (–3,2), and use it to approximate (–2.9, 2.1). fx (x,y) = 2 cos(2x + 3y) and fy(x,y) = 3 cos(2x + 3y). Both are continuous on their domain, since they are an algebraic combination of continuous functions, and (–3,2) is in their domain, since the cosine is continuous on the entire real number line. By Thm. 8, f(x,y) is differentiable at (–3,2).L(x,y) = fx(–3,2)(x + 3) + fy(–3,2) (y – 2) + f(–3,2) = 2(x + 3) + 3(y – 2) + 0 = 2x + 3y + 6 – 6 = 2x + 3y. L(–2.9, 2.1) = 2(–2.9) + 3(2.1) = 0.5 Six points: 3 each for a and b (1 for explanation, 1 for linearization, 1 for approximation) 3. Suppose you need to know an equation of the tangent plane to a surface S at the point p(2, 1, 3). You don’t have an equation for S but you know that the curves r1(t) = <2 + 3t, 1 – t2, 3 – 4t + t2> and r2(u) = <1 + u2, 2u3 – 1, 2u + 1> both lie on S. Find an equation of the tangent plane at P. The direction vectors of r′1(t) and r′2(u) will lie on the tangent plane, and if they are not parallel, their cross product will be the normal vector to the tangent plane. With the point P(2,1,3) we will have the equation for the plane. r1(t) gives point P if t = 0 and r2(u) gives P if u = 1. r′1(t) = <3, – 2t, – 4 + 2t> and r′1(0) = <3, 0, – 4> r2(u) = <2u, 6u2, 2> and r′2(1) = <2, 6, 2>, which is a scalar multiple of <1, 3, 1>. Note that r′1(0) = <3, 0, – 4> and r′1(0) = <3, 0, – 4> are not scalar multiples of each other, so they are not parallel. n = <3, 0, – 4> × <1, 3, 1> = < 12, –7, 9> The tangent plane equation is 12(x – 2) – 7(y – 1) + 9(z – 3) = 0 ⇒ 12x – 7y + 9z – 44 = 0. Four points: 1 for r′1(0), 1 for r′2(1), 1 for n, 1 for the tangent plane equation. 4. Use the Chain Rule to find a) dt dw for w = ln x2 + y2 + z 2 , x = sin t, y = cos t, z = tan t. t x y z z t x y z y t x y z x dt dz wz dt dy wy dt dx wx dt dw 2 2 2 2 2 2 2 2 2 2 sec 2 2 cos 2 sin + + + + + − + + = ∂ ∂ + ∂ ∂ + ∂ ∂ = t t t t t t t t t t t t t t 2 tan [Show More]

Last updated: 1 year ago

Preview 1 out of 5 pages