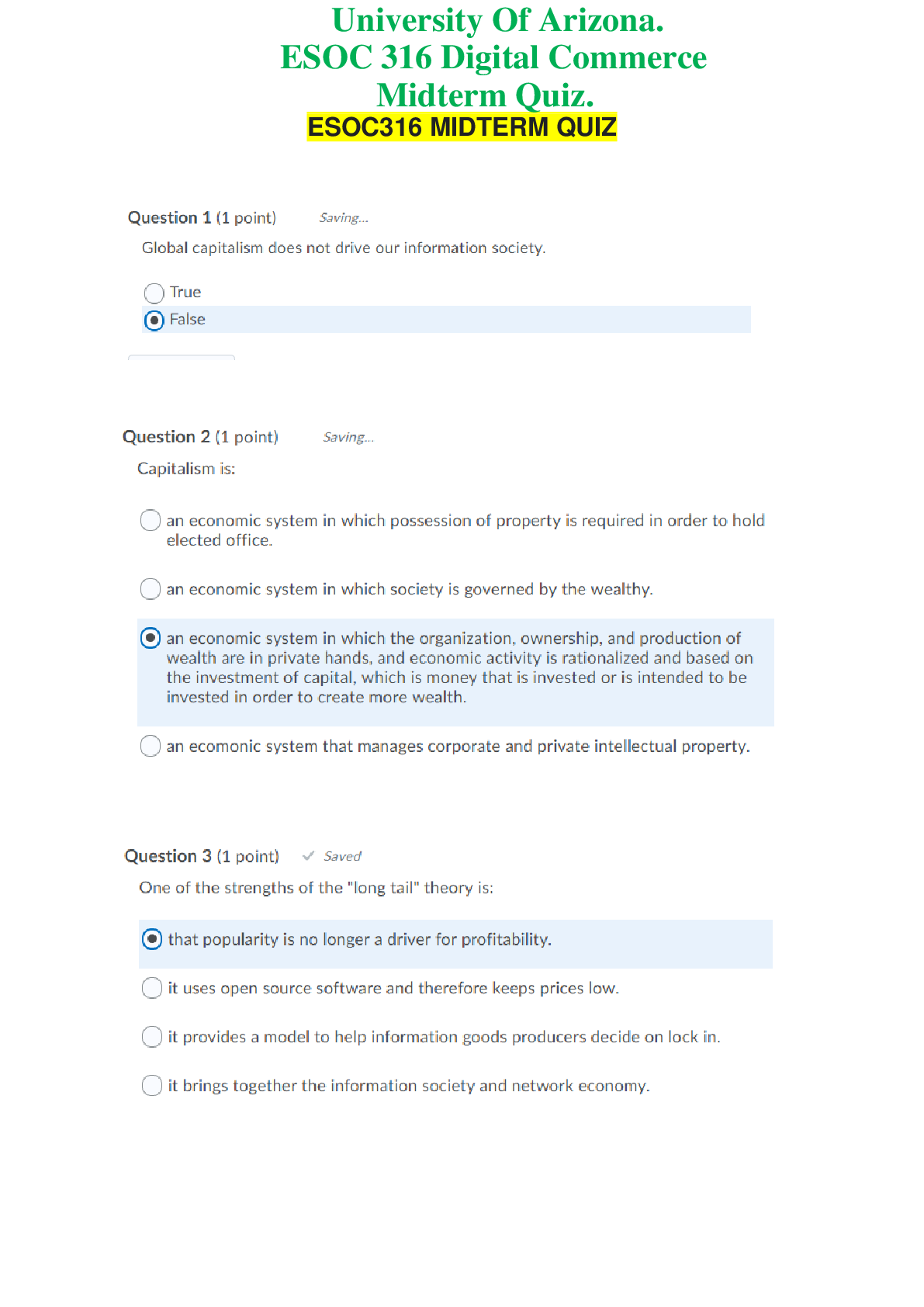
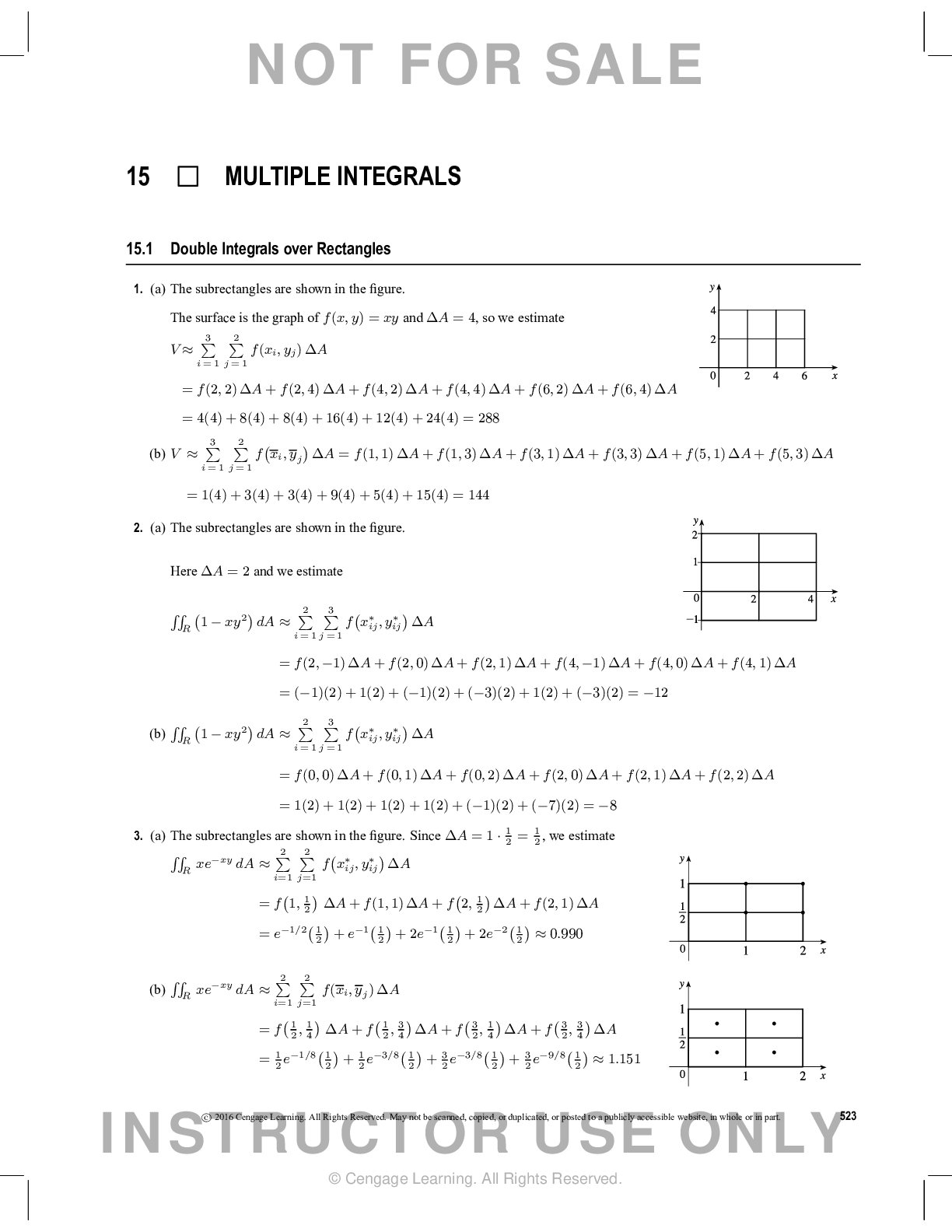
Calculus > QUESTIONS & ANSWERS > Chapter 5 INTEGRALS. Work and Answers (All)
Chapter 5 INTEGRALS. Work and Answers
Document Content and Description Below
5.1 Areas and Distances 1. (a) Since is decreasing , we can obtain a lower estimate by using right endpoints. We are instructed to use five rectangles, so = 5. 5 = 5 =1 (�... ��)∆ ∆ = − = 105− 0 = 2 = (1) · 2 + (2) · 2 + (3) · 2 + (4) · 2 + (5) · 2 = 2[(2) + (4) + (6) + (8) + (10)] ≈ 2(32 + 18 + 08 + 02 + 0) = 2(6) = 12 Since is decreasing , we can obtain an upper estimate by using left endpoints. 5 = 5 =1 (−1)∆ = (0) · 2 + (1) · 2 + (2) · 2 + (3) · 2 + (4) · 2 = 2[(0) + (2) + (4) + (6) + (8)] ≈ 2(5 + 32 + 18 + 08 + 02) = 2(11) = 22 (b) 10 = 10 =1 ()∆ ∆ = 1010 − 0 = 1 = 1[(1) + (2) + · · · + (10)] = (1) + (2) + · · · + (10) ≈ 4 + 32 + 25 + 18 + 13 + 08 + 05 + 02 + 01 + 0 = 144 10 = 10 =1 (−1)∆ = (0) + (1) + · · · + (9) = 10 + 1 · (0) − 1 · (10) subtract rightmost lower rectangle add leftmost upper rectangle, = 144 + 5 − 0 = 194 °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. 1 NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.2 ¤ CHAPTER 5 INTEGRALS 2. (a) (i) 6 = 6 =1 (−1)∆ [∆ = 126− 0 = 2] = 2[(0) + (1) + (2) + (3) + (4) + (5)] = 2[(0) + (2) + (4) + (6) + (8) + (10)] ≈ 2(9 + 88 + 82 + 73 + 59 + 41) = 2(433) = 866 (ii) 6 = 6 + 2 · (12) − 2 · (0) ≈ 866 + 2(1) − 2(9) = 706 [Add area of rightmost lower rectangle and subtract area of leftmost upper rectangle.] (iii) 6 = 6 =1 ()∆ = 2[(1) + (3) + (5) + (7) + (9) + (11)] ≈ 2(89 + 85 + 78 + 66 + 51 + 28) = 2(397) = 794 (b) Since is decreasing, we obtain an overestimate by using left endpoints; that is, 6. (c) Since is decreasing, we obtain an underestimate by using right endpoints; that is, 6. (d) 6 gives the best estimate, since the area of each rectangle appears to be closer to the true area than the overestimates and underestimates in 6 and 6. 3. (a) 4 = 4 =1 ()∆ ∆ = 2 −4 1 = 1 4 = =1 4 ()∆ = [(1) + (2) + (3) + (4)] ∆ = 514 + 614 + 714 + 81 4 14 = 4 5 + 2 3 + 4 7 + 1 2 1 4 ≈ 06345 Since is decreasing on [12], an underestimate is obtained by using the right endpoint approximation, 4. (b) 4 = 4 =1 (−1)∆ = =1 4 (−1) ∆ = [(0) + (1) + (2) + (3)] ∆ = 11 + 514 + 614 + 714 1 4 = 1 + 4 5 + 2 3 + 4 7 1 4 ≈ 07595 4 is an overestimate. Alternatively, we could just add the area of the leftmost upper rectangle and subtract the area of the rightmost lower rectangle; that is, 4 = 4 + (1) · 1 4 − (2) · 1 4. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.1 AREAS AND DISTANCES ¤ 3 4. (a) 4 = 4 =1 () ∆ ∆ = 24− 0 = 8 = =1 4 () ∆ = [(1) + (2) + (3) + (4)] ∆ = sin 8 + sin 28 + sin 38 + sin 48 8 ≈ 11835 Since is increasing on 0 2 , 4 is an overestimate. (b) 4 = 4 =1 (−1) ∆ = =1 4 (−1) ∆ = [(0) + (1) + (2) + (3)] ∆ = sin 0 + sin 8 + sin 28 + sin 38 8 ≈ 07908 Since is increasing on 0 2 , 4 is an underestimate. 5. (a) () = 1 + 2 and ∆ = 2 − (−1) 3 = 1 ⇒ 3 = 1 · (0) + 1 · (1) + 1 · (2) = 1 · 1 + 1 · 2 + 1 · 5 = 8. ∆ = 2 − (−1) 6 = 05 ⇒ 6 = 05[(−05) + (0) + (05) + (1) + (15) + (2)] = 05(125 + 1 + 125 + 2 + 325 + 5) = 05(1375) = 6875 (b) 3 = 1 · (−1) + 1 · (0) + 1 · (1) = 1 · 2 + 1 · 1 + 1 · 2 = 5 6 = 05[(−1) + (−05) + (0) + (05) + (1) + (15)] = 05(2 + 125 + 1 + 125 + 2 + 325) = 05(1075) = 5375 (c) 3 = 1 · (−05) + 1 · (05) + 1 · (15) = 1 · 125 + 1 · 125 + 1 · 325 = 575 6 = 05[(−075) + (−025) + (025) + (075) + (125) + (175)] = 05(15625 + 10625 + 10625 + 15625 + 25625 + 40625) = 05(11875) = 59375 (d) 6 appears to be the best estimate. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.4 ¤ CHAPTER 5 INTEGRALS 6. (a) (b) () = − 2ln and ∆ = 5 − 1 4 = 1 ⇒ (i) 4 = 1 · (2) + 1 · (3) + 1 · (4) + 1 · (5) = (2 − 2ln2) + (3 − 2ln3) + (4 − 2ln4) + (5 − 2ln5) ≈ 4425 (ii) 4 = 1 · (15) + 1 · (25) + 1 · (35) + 1 · (45) = (15 − 2ln 15) + (25 − 2ln25) + (35 − 2ln35) + (45 − 2ln45) ≈ 3843 (c) (i) 8 = 1 2[(15) + (2) + · · · + (5)] = 1 2 [(15 − 2ln15) + (2 − 2ln2) + · · · + (5 − 2ln5)] ≈ 4134 (ii) 8 = 1 2[(125) + (175) + · · · + (475)] = 1 2 [(125 − 2ln125) + (175 − 2ln175) + · · · + (475 − 2ln475)] ≈ 3889 7. () = 2 + sin, 0 ≤ ≤ , ∆ = . = 2: The maximum values of on both subintervals occur at = 2 , so upper sum = 2 · 2 + 2 · 2 = 3 · 2 + 3 · 2 = 3 ≈ 942.2 The minimum values of on the subintervals occur at = 0 and = , so lower sum = (0) · 2 + () · 2 = 2 · 2 + 2 · 2 = 2 ≈ 628. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.1 AREAS AND DISTANCES ¤ 5 = 4: upper sum = 4 + 2 + 2 + 34 4 = 2 + 1 2√2 + (2 + 1) + (2 + 1) + 2 + 1 2√2 4 = 10 + √2 4 ≈ 896 lower sum = (0) + 4 + 34 + () 4 = (2 + 0) + 2 + 1 2√2 + 2 + 1 2√2 + (2 + 0) 4 = 8 + √2 4 ≈ 739 = 8: upper sum = 8 + 4 + 38 + 2 + 2 + 58 + 34 + 78 8 ≈ 865 lower sum = (0) + 8 + 4 + 38 + 58 + 34 + 78 + () 8 ≈ 786 8. () = 1 + 2, −1 ≤ ≤ 1, ∆ = 2. = 3: upper sum = (−1) + 1 3 + (1) 2 3 = 2 + 10 9 + 2 2 3 = 92 27 ≈ 341 lower sum = − 1 3 + (0) + 1 3 2 3 = 10 9 + 1 + 10 9 2 3 = 58 27 ≈ 215 = 4: upper sum = (−1) + − 1 2 + 1 2 + (1) 2 4 = 2 + 5 4 + 5 4 + 2 1 2 = 13 4 = 325 lower sum = − 1 2 + (0) + (0) + 1 2 2 4 = 5 4 + 1 + 1 + 5 4 1 2 = 9 4 = 225 9. Here is one possible algorithm (ordered sequence of operations) for calculating the sums: 1 Let SUM = 0, X_MIN = 0, X_MAX = 1, N = 10 (depending on which sum we are calculating), DELTA_X = (X_MAX - X_MIN)/N, and RIGHT_ENDPOINT = X_MIN + DELTA_X. 2 Repeat steps 2a, 2b in sequence until RIGHT_ENDPOINT X_MAX. 2a Add (RIGHT_ENDPOINT)^4 to SUM. Add DELTA_X to RIGHT_ENDPOINT. At the end of this procedure, (DELTA_X)·(SUM) is equal to the answer we are looking for. We find that 10 = 1 10 10 =1 10 4 ≈ 02533, 30 = 30 1 30 =1 30 4 ≈ 02170, 50 = 50 1 50 =1 50 4 ≈ 02101 and °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.6 ¤ CHAPTER 5 INTEGRALS 100 = 1 100 100 =1 100 4 ≈ 02050. It appears that the exact area is 02. The following display shows the program SUMRIGHT and its output from a TI-83/4 Plus calculator. To generalize the program, we have input (rather than assign) values for Xmin, Xmax, and N. Also, the function, 4, is assigned to Y1, enabling us to evaluate any right sum merely by changing Y1 and running the program. 10. We can use the algorithm from Exercise 9 with X_MIN = 0, X_MAX = 2, and cos(RIGHT_ENDPOINT) instead of (RIGHT_ENDPOINT)^4 in step 2a. We find that 10 = 2 10 10 =1 cos20 ≈ 09194, 30 = 302 30 =1cos60 ≈ 09736, and 50 = 2 50 50 =1 cos100 ≈ 09842, and 100 = 1002 100 =1cos200 ≈ 09921. It appears that the exact area is 1. 11. In Maple, we have to perform a number of steps before getting a numerical answer. After loading the student package [command: with(student);] we use the command left_sum:=leftsum(1/(xˆ2+1),x=0..1,10 [or 30, or 50]); which gives us the expression in summation notation. To get a numerical approximation to the sum, we use evalf(left_sum);. Mathematica does not have a special command for these sums, so we must type them in manually. For example, the first left sum is given by (1/10)*Sum[1/(((i-1)/10)ˆ2+1)],{i,1,10}], and we use the N command on the resulting output to get a numerical approximation. In Derive, we use the LEFT_RIEMANN command to get the left sums, but must define the right sums ourselves. (We can define a new function using LEFT_RIEMANN with ranging from 1 to instead of from 0 to − 1.) (a) With () = 1 2 + 1, 0 ≤ ≤ 1, the left sums are of the form = 1 =1 −112 + 1. Specifically, 10 ≈ 08100, 30 ≈ 07937, and 50 ≈ 07904. The right sums are of the form = 1 =1 1 2 + 1. Specifically, 10 ≈ 07600, 30 ≈ 07770, and 50 ≈ 07804. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.1 AREAS AND DISTANCES ¤ 7 (b) In Maple, we use the leftbox (with the same arguments as left_sum) and rightbox commands to generate the graphs. left endpoints, = 10 left endpoints, = 30 left endpoints, = 50 right endpoints, = 10 right endpoints, = 30 right endpoints, = 50 (c) We know that since = 1(2 + 1) is a decreasing function on (01), all of the left sums are larger than the actual area, and all of the right sums are smaller than the actual area. Since the left sum with = 50 is about 07904 0791 and the right sum with = 50 is about 07804 0780, we conclude that 0780 50 exact area 50 0791, so the exact area is between 0780 and 0791. 12. See the solution to Exercise 11 for the CAS commands for evaluating the sums. (a) With () = ln, 1 ≤ ≤ 4, the left sums are of the form = 3 =1 ln1 + 3(− 1). In particular, 10 ≈ 23316, 30 ≈ 24752, and 50 ≈ 25034. The right sums are of the form = 3 =1 ln1 + 3. In particular, 10 ≈ 27475, 30 ≈ 26139, and 50 ≈ 25865. (b) In Maple, we use the leftbox (with the same arguments as left_sum) and rightbox commands to generate the graphs. left endpoints, = 10 left endpoints, = 30 left endpoints, = 50 right endpoints, = 10 right endpoints, = 30 right endpoints, = 50 °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.8 ¤ CHAPTER 5 INTEGRALS (c) We know that since = ln is an increasing function on (1 4), all of the left sums are smaller than the actual area, and all of the right sums are larger than the actual area. Since the left sum with = 50 is about 2503 250 and the right sum with = 50 is about 2587 259, we conclude that 250 50 exact area 50 259, so the exact area is between 250 and 259. 13. Since is an increasing function, 6 will give us a lower estimate and 6 will give us an upper estimate. 6 = (0 fts)(05 s) + (62)(05) + (108)(05) + (149)(05) + (181)(05) + (194)(05) = 05(694) = 347 ft 6 = 05(62 + 108 + 149 + 181 + 194 + 202) = 05(896) = 448 ft 14. (a) The velocities are given with units mih, so we must convert the 10-second intervals to hours: 10 seconds = 10 seconds 3600 secondsh = 1 360 h distance ≈ 6 = (1829 mih) 360 1 h + (1680) 360 1 + (1066) 360 1 + (998) 360 1 + (1245) 360 1 + (1761) 360 1 = 8579 360 ≈ 2383 miles (b) Distance ≈ 6 = 360 1 (1680 + 1066 + 998 + 1245 + 1761 + 1756) = 850 3606 ≈ 2363 miles (c) The velocity is neither increasing nor decreasing on the given interval, so the estimates in parts (a) and (b) are neither upper nor lower estimates. 15. Lower estimate for oil leakage: 5 = (76 + 68 + 62 + 57 + 53)(2) = (316)(2) = 632 L. Upper estimate for oil leakage: 5 = (87 + 76 + 68 + 62 + 57)(2) = (35)(2) = 70 L. 16. We can find an upper estimate by using the final velocity for each time interval. Thus, the distance traveled after 62 seconds can be approximated by = 6 =1 () ∆ = (185 fts)(10 s) + 319 · 5 + 447 · 5 + 742 · 12 + 1325 · 27 + 1445 · 3 = 54,694 ft 17. For a decreasing function, using left endpoints gives us an overestimate and using right endpoints results in an underestimate. We will use 6 to get an estimate. ∆ = 1, so 6 = 1[(05) + (15) + (25) + (35) + (45) + (55)] ≈ 55 + 40 + 28 + 18 + 10 + 4 = 155 ft For a very rough check on the above calculation, we can draw a line from (070) to (60) and calculate the area of the triangle: 1 2(70)(6) = 210. This is clearly an overestimate, so our midpoint estimate of 155 is reasonable. 18. For an increasing function, using left endpoints gives us an underestimate and using right endpoints results in an overestimate. We will use 6 to get an estimate. ∆ = 306− 0 = 5 s = 3600 5 h = 720 1 h. 6 = 720 1 [(25) + (75) + (125) + (175) + (225) + (275)] = 1 720(3125 + 66 + 88 + 1035 + 11375 + 11925) = 720 1 (52175) ≈ 0725 km For a very rough check on the above calculation, we can draw a line from (00) to (30 120) and calculate the area of the triangle: 1 2(30)(120) = 1800. Divide by 3600 to get 05, which is clearly an underestimate, making our midpoint estimate of 0725 seem reasonable. Of course, answers will vary due to different readings of the graph. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.1 AREAS AND DISTANCES ¤ 9 19. () = −( − 21)( + 1) and ∆ = 126− 0 = 2 6 = 2 · (1) + 2 · (3) + 2 · (5) + 2 · (7) + 2 · (9) + 2 · (11) = 2 · 40 + 2 · 216 + 2 · 480 + 2 · 784 + 2 · 1080 + 2 · 1320 = 7840 (infected cellsmL) · days Thus, the total amount of infection needed to develop symptoms of measles is about 7840 infected cells per mL of blood plasma. 20. (a) Use ∆ = 14 days. The number of people who died of SARS in Singapore between March 1 and May 24, 2003, using left endpoints is 6 = 14(00079 + 00638 + 01944 + 04435 + 05620 + 04630) = 14(17346) = 242844 ≈ 24 people Using right endpoints, 6 = 14(00638 + 01944 + 04435 + 05620 + 04630 + 02897) = 14(20164) = 282296 ≈ 28 people (b) Let be the number of days since March 1, 2003, () be the number of deaths per day on day , and the graph of = () be a reasonable continuous function on the interval [0 84]. Then the number of SARS deaths from = to = is approximately equal to the area under the curve = () from = to = . 21. () = 2 2 + 1, 1 ≤ ≤ 3. ∆ = (3 − 1) = 2 and = 1 + ∆ = 1 + 2. = lim →∞ = lim →∞ =1 ()∆ = lim →∞ =1 2(1 + 2) (1 + 2)2 + 1 · 2 . 22. () = 2 + √1 + 2, 4 ≤ ≤ 7. ∆ = (7 − 4) = 3 and = 4 + ∆ = 4 + 3. = lim →∞ = lim →∞ =1 ()∆ = lim →∞ =1 (4 + 3)2 + 1 + 2(4 + 3) · 3 . 23. () = √sin, 0 ≤ ≤ . ∆ = ( − 0) = and = 0 + ∆ = . = lim →∞ = lim →∞ =1 ()∆ = lim →∞ =1 sin() · . 24. lim →∞ =1 3 1 + 3 can be interpreted as the area of the region lying under the graph of = √1 + on the interval [03], since for = √1 + on [03] with ∆ = 3 − 0 = 3 , = 0 + ∆ = 3 , and ∗ = , the expression for the area is = lim →∞ =1 (∗ )∆ = lim →∞ =1 1 + 3 3 . Note that this answer is not unique. We could use = √ on [14] or, in general, = √ − on [ + 1 + 4], where is any real number. 25. lim →∞ =1 4 tan 4 can be interpreted as the area of the region lying under the graph of = tan on the interval 0 4 , since for = tan on 0 4 with ∆ = 4 − 0 = 4 , = 0 + ∆ = 4 , and ∗ = , the expression for the area is = lim →∞ =1 (∗ )∆ = lim →∞ =1 tan4 4 . Note that this answer is not unique, since the expression for the area is the same for the function = tan( − ) on the interval + 4 , where is any integer. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.10 ¤ CHAPTER 5 INTEGRALS 26. (a) ∆ = 1 − 0 = 1 and = 0 + ∆ = . = lim →∞ = lim →∞ =1 ()∆ = lim →∞ =1 3 · 1 . (b) lim →∞ =1 3 3 · 1 = lim →∞ 1 4 =1 3 = lim →∞ 1 4 (2+ 1)2 = lim →∞ (4+ 1) 2 2 = 14 lim →∞1 + 1 2 = 14 27. (a) Since is an increasing function, is an underestimate of [lower sum] and is an overestimate of [upper sum]. Thus, , , and are related by the inequality . (b) = (1)∆ + (2)∆ + · · · + ()∆ = (0)∆ + (1)∆ + · · · + (−1)∆ − = ()∆ − (0)∆ = ∆[() − (0)] = − [() − ()] In the diagram, − is the sum of the areas of the shaded rectangles. By sliding the shaded rectangles to the left so that they stack on top of the leftmost shaded rectangle, we form a rectangle of height () − () and width − . (c) , so − − ; that is, − − [() − ()]. 28. − − [() − ()] = 3 − 1 [(3) − (1)] = 2 (3 − ) Solving 2 (3 − ) 00001 for gives us 2(3 − ) 00001 ⇒ 2(3 − ) 00001 ⇒ 3473451. Thus, a value of that assures us that − 00001 is = 347346. [This is not the least value of .] 29. (a) = () = 5. ∆ = 2 − 0 = 2 and = 0 + ∆ = 2 . = lim →∞ = lim →∞ =1 ()∆ = lim →∞ =125 · 2 = lim →∞ =1 32 55 · 2 = lim →∞ 646 =1 5. (b) =1 5 CAS = 2( + 1)222 + 2 − 1 12 (c) lim →∞ 64 6 · 2( + 1)222 + 2 − 1 12 = 64 12 lim →∞ 2 + 2 + 122 + 2 − 1 2 · 2 = 16 3 lim →∞1 + 2 + 12 2 + 2 − 12 = 16 3 · 1 · 2 = 32 3 30. From Example 3(a), we have = lim →∞ 2 =1 −2. Using a CAS, =1 −2 = −22 − 1 2 − 1 and lim →∞ 2 · −22 − 1 2 − 1 = −22 − 1 ≈ 08647, whereas the estimate from Example 3(b) using 10 was 08632. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.2 THE DEFINITE INTEGRAL ¤ 11 31. = () = cos. ∆ = − 0 = and = 0 + ∆ = . = lim →∞ = lim →∞ =1 ()∆ = lim →∞ =1 cos · CAS = lim →∞ sin21 + 1 2sin2 − 2 CAS = sin If = 2 , then = sin 2 = 1. 32. (a) The diagram shows one of the congruent triangles, 4, with central angle 2. is the center of the circle and is one of the sides of the polygon. Radius is drawn so as to bisect ∠. It follows that intersects at right angles and bisects . Thus, 4 is divided into two right triangles with legs of length 1 2() = sin() and cos(). 4 has area 2 · 1 2[ sin()][ cos()] = 2 sin() cos() = 1 2 2 sin(2), so = · area(4) = 1 2 2 sin(2). (b) To use Equation 3.3.2, lim →0 sin = 1, we need to have the same expression in the denominator as we have in the argument of the sine function—in this case, 2. lim →∞ = lim →∞ 1 2 2 sin(2) = lim →∞ 1 2 2 sin(2) 2 · 2 = lim →∞ sin(2) 2 2. Let = 2 . Then as → ∞, → 0, so lim →∞ sin(2) 2 2 = lim →0 sin 2 = (1)2 = 2. 5.2 The Definite Integral 1. () = − 1, −6 ≤ ≤ 4. ∆ = − = 4 − (−6) 5 = 2. Since we are using right endpoints, ∗ = . 5 = 5 =1 () ∆ = (∆)[(1) + (2) + (3) + (4) + (5) + (6)] = 2[(−4) + (−2) + (0) + (2) + (4)] = 2[−5 + (−3) + (−1) + 1 + 3] = 2(−5) = −10 The Riemann sum represents the sum of the areas of the two rectangles above the -axis minus the sum of the areas of the three rectangles below the -axis; that is, the net area of the rectangles with respect to the -axis. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.12 ¤ CHAPTER 5 INTEGRALS 2. () = cos, 0 ≤ ≤ 34 . ∆ = − = 34 − 0 6 = 8 . Since we are using left endpoints, ∗ = −1. 6 = 6 =1 (−1)∆ = (∆)[(0) + (1) + (2) + (3) + (4) + (5)] = 8 [(0) + 8 + 28 + 38 + 48 + 58] ≈ 1033186 The Riemann sum represents the sum of the areas of the four rectangles above the -axis minus the area of the rectangle below the -axis; that is, the net area of the rectangles with respect to the -axis. A sixth rectangle is degenerate, with height 0, and has no area. 3. () = 2 − 4, 0 ≤ ≤ 3. ∆ = − = 3 − 0 6 = 1 2 . Since we are using midpoints, ∗ = = 1 2(−1 + ). 6 = 6 =1 () ∆ = (∆)[(1) + (2) + (3) + (4) + (5) + (6)] = 1 2 1 4 + 3 4 + 5 4 + 7 4 + 9 4 + 11 4 = 1 2 − 63 16 − 55 16 − 39 16 − 15 16 + 17 16 + 57 16 = 1 2− 98 16 = − 49 16 The Riemann sum represents the sum of the areas of the two rectangles above the -axis minus the sum of the areas of the four rectangles below the -axis; that is, the net area of the rectangles with respect to the -axis. 4. (a) () = 1 , 1 ≤ ≤ 2. ∆ = − = 2 − 1 4 = 1 4 . Since we are using right endpoints, ∗ = . 4 = 4 =1 () ∆ = (∆)[(1) + (2) + (3) + (4)] = 1 4 [ 5 4 + 6 4 + 7 4 + 8 4] = 1 4 4 5 + 2 3 + 4 7 + 1 2 ≈ 0634524 The Riemann sum represents the sum of the areas of the four rectangles. (b) Since we are using midpoints, ∗ = = 1 2(−1 + ). 4 = 4 =1 () ∆ = (∆)[(1) + (2) + (3) + (4)] = 1 4 9 8 + 11 8 + 13 8 + 15 8 = 1 4 8 9 + 11 8 + 13 8 + 15 8 ≈ 0691220 The Riemann sum represents the sum of the areas of the four rectangles. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.2 THE DEFINITE INTEGRAL ¤ 13 5. (a) 010 () ≈ 5 = [(2) + (4) + (6) + (8) + (10)]∆ = [−1 + 0 + (−2) + 2 + 4](2) = 3(2) = 6 (b) 010 () ≈ 5 = [(0) + (2) + (4) + (6) + (8)] ∆ = [3 + (−1) + 0 + (−2) + 2](2) = 2(2) = 4 (c) 010 () ≈ 5 = [(1) + (3) + (5) + (7) + (9)]∆ = [0 + (−1) + (−1) + 0 + 3](2) = 1(2) = 2 6. (a) −42 () ≈ 6 = [(−1) + (0) + (1) + (2) + (3) + (4)]∆ = − 3 2 + 0 + 3 2 + 1 2 + (−1) + 1 2(1) = 0 (b) −42 () ≈ 6 = [(−2) + (−1) + (0) + (1) + (2) + (3)]∆ = 0 + − 3 2 + 0 + 3 2 + 1 2 + (−1)(1) = − 1 2 (c) −42 () ≈ 6 = − 3 2 + − 1 2 + 1 2 + 3 2 + 5 2 + 7 2 ∆ = −1 + (−1) + 1 + 1 + 0 + − 1 2(1) = − 1 2 7. Since is increasing, 5 ≤ 10 30 () ≤ 5. Lower estimate = 5 = 5 =1 (−1)∆ = 4[(10) + (14) + (18) + (22) + (26)] = 4[−12 + (−6) + (−2) + 1 + 3] = 4(−16) = −64 Upper estimate = 5 = 5 =1 ()∆ = 4[(14) + (18) + (22) + (26) + (30)] = 4[−6 + (−2) + 1 + 3 + 8] = 4(4) = 16 8. (a) Using the right endpoints to approximate 39 (), we have 3 =1 ()∆ = 2[(5) + (7) + (9)] = 2 (−06 + 09 + 18) = 42. Since is increasing, using right endpoints gives an overestimate. (b) Using the left endpoints to approximate 39 (), we have 3 =1 (−1)∆ = 2[(3) + (5) + (7)] = 2(−34 − 06 + 09) = −62. Since is increasing, using left endpoints gives an underestimate. (c) Using the midpoint of each interval to approximate 39 (), we have 3 =1 ()∆ = 2[(4) + (6) + (8)] = 2(−21 + 03 + 14) = −08. We cannot say anything about the midpoint estimate compared to the exact value of the integral. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.14 ¤ CHAPTER 5 INTEGRALS 9. ∆ = (8 − 0)4 = 2, so the endpoints are 0, 2, 4, 6, and 8, and the midpoints are 1, 3, 5, and 7. The Midpoint Rule gives 08 sin√ ≈ 4 =1 (¯ )∆ = 2sin√1 + sin√3 + sin√5 + sin√7 ≈ 2(30910) = 61820. 10. ∆ = (1 − 0)5 = 1 5 , so the endpoints are 0, 1 5 , 2 5 , 3 5 , 4 5 , and 1, and the midpoints are 10 1 , 10 3 , 10 5 , 10 7 , and 10 9 . The Midpoint Rule gives 01 3 + 1 ≈ =1 5 (¯ )∆ = 1 5 10 1 3 + 1 + 10 3 3 + 1 + 10 5 3 + 1 + 10 7 3 + 1 + 10 9 3 + 1 ≈ 11097 11. ∆ = (2 − 0)5 = 2 5 , so the endpoints are 0, 2 5 , 4 5 , 6 5 , 8 5 , and 2, and the midpoints are 1 5 , 3 5 , 5 5 , 7 5 and 9 5 . The Midpoint Rule gives 02 + 1 ≈ =1 5 (¯ )∆ = 25 1 5 + 1 1 5 + 3 5 + 1 3 5 + 5 5 + 1 5 5 + 7 5 + 1 7 5 + 9 5 + 1 9 5 = 25127 56 = 127 140 ≈ 09071. 12. ∆ = ( − 0)4 = 4 , so the endpoints are 4 , 24 , 34 , and 44 , and the midpoints are 8 , 38 , 58 , and 78 . The Midpoint Rule gives 0 sin2 ≈ =1 5 (¯ )∆ = 4 8 sin2 8 + 38 sin2 38 + 58 sin2 58 + 78 sin2 78 ≈ 24674 13. In Maple 14, use the commands with(Student[Calculus1]) and ReimannSum(x/(x+1),0..2,partition=5,method=midpoint,output=plot). In some older versions of Maple, use with(student) to load the sum and box commands, then m:=middlesum(x/(x+1),x=0..2), which gives us the sum in summation notation, then M:=evalf(m) to get the numerical approximation, and finally middlebox(x/(x+1),x=0..2) to generate the graph. The values obtained for = 5, 10, and 20 are 09071, 09029, and 09018, respectively. 14. For () = ( + 1) on [02], we calculate 100 ≈ 089469 and 100 ≈ 090802. Since is increasing on [02], 100 is an underestimate of 02 + 1 and 100 is an overestimate. Thus, 08946 02 + 1 09081 15. We’ll create the table of values to approximate 0 sin by using the program in the solution to Exercise 5.1.9 with Y1 = sin, Xmin = 0, Xmax = , and = 5, 10, 50, and 100. The values of appear to be approaching 2. 5 1933766 10 1983524 50 1999342 100 1999836 °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.2 THE DEFINITE INTEGRAL ¤ 15 16. 02 −2 with = 5, 10, 50, and 100. 5 1077467 0684794 10 0980007 0783670 50 0901705 0862438 100 0891896 0872262 The value of the integral lies between 0872 and 0892. Note that () = −2 is decreasing on (0 2). We cannot make a similar statement for −21 −2 since is increasing on (−10). 17. On [0 1], lim →∞ =1 1 + ∆ = 01 1 + . 18. On [2 5], lim →∞ =1 1 + 3 ∆ = 25 √1 + 3 . 19. On [2 7], lim →∞ =1 [5(∗ )3 − 4∗ ]∆ = 27(53 − 4). 20. On [1 3], lim →∞ =1 ∗ (∗ )2 + 4 ∆ = 13 2+ 4 . 21. Note that ∆ = 5 − 2 = 3 and = 2 + ∆ = 2 + 3 . 25(4 − 2) = lim →∞ =1 ()∆ = lim →∞ =1 2 + 3 3 = lim →∞ 3 =1 4 − 22 + 3 = lim →∞ 3 =1 −6 = lim →∞ 3 −6 =1 = lim →∞ −182 (2+ 1) = lim →∞ −18 2 + 1 = −9 lim →∞ 1 + 1 = −9(1) = −9 22. Note that ∆ = 4 − 1 = 3 and = 1 + ∆ = 1 + 3 . 14(2 − 4 + 2) = lim →∞ =1 ()∆ = lim →∞ =1 1 + 3 3 = lim →∞ 3 =1 1 + 3 2 − 41 + 3 + 2 = lim →∞ 3 =1 1 + 6 + 922 − 4 − 12 + 2 = lim →∞ 3 =1 922 − 6 − 1 = lim →∞ 3 92 =1 2 − 6 =1 − =1 1 = lim →∞ 273 ( + 1)(2 6 + 1) − 182 (2+ 1) − 3 · (1) = lim →∞ 9 2 ( + 1)(2 2 + 1) − 9 + 1 − 3 = lim →∞ 9 2 + 1 2+ 1 − 91 + 1 − 3 = lim →∞ 9 21 + 1 2 + 1 − 91 + 1 − 3 = 9 2(1)(2) − 9(1) − 3 = −3 °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.16 ¤ CHAPTER 5 INTEGRALS 23. Note that ∆ = 0 − (−2) = 2 and = −2 + ∆ = −2 + 2 . −02(2 + ) = lim →∞ =1 ()∆ = lim →∞ =1 −2 + 2 2 = lim →∞ 2 =1 −2 + 22 + −2 + 2 = lim →∞ 2 =1 4 − 8 + 422 − 2 + 2 = lim →∞ 2 =1 422 − 6 + 2 = lim →∞ 2 42 =1 2 − 6 =1 + =1 2 = lim →∞ 83 ( + 1)(2 6 + 1) − 122 (2+ 1) + 2 · (2) = lim →∞ 4 3 ( + 1)(2 2 + 1) − 6 + 1 + 4 = lim →∞ 4 3 + 1 2+ 1 − 61 + 1 + 4 = lim →∞ 4 31 + 1 2 + 1 − 61 + 1 + 4 = 43(1)(2) − 6(1) + 4 = 23 24. Note that ∆ = 2 − 0 = 2 and = 0 + ∆ = 2 . 02(2 − 3) = lim →∞ =1 ()∆ = lim →∞ =1 2 2 = lim →∞ 2 =1 22 − 23 = lim →∞ 2 =1 4 − 833 = lim →∞ 2 4 =1 − 83 =1 3 = lim →∞ 82 (2+ 1) − 164 (2+ 1)2 = lim →∞ 4 + 1 − 4 ( + 1) 2 2 = lim →∞ 41 + 1 − 4 + 1 + 1 = lim →∞ 41 + 1 − 41 + 1 1 + 1 = 4(1) − 4(1)(1) = 0 25. Note that ∆ = 1 − 0 = 1 and = 0 + ∆ = . 01(3 − 32) = lim →∞ =1 ()∆ = lim →∞ =1 ∆ = lim →∞ =1 3 − 3 2 1 = lim →∞ 1 =1 33 − 322 = lim →∞ 1 13 =1 3 − 32 =1 2 = lim →∞ 14 (2+ 1)2 − 33 ( + 1)(2 6 + 1) = lim →∞ 1 4 + 1 + 1 − 1 2 + 1 2+ 1 = lim →∞ 1 41 + 1 1 + 1 − 1 21 + 1 2 + 1 = 14(1)(1) − 1 2(1)(2) = −34 26. (a) ∆ = (4 − 0)8 = 05 and ∗ = = 05. 04(2 − 3) ≈ 8 =1 (∗ )∆ = 05052 − 3(05) + 102 − 3(10) + · · · + 352 − 3(35) + 402 − 3(40) = 1 2 − 5 4 − 2 − 9 4 − 2 − 5 4 + 0 + 7 4 + 4 = −15 (b) °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.2 THE DEFINITE INTEGRAL ¤ 17 (c) 04(2 − 3) = lim →∞ =1 42 − 344 = lim →∞ 4 162 =1 2 − 12 =1 = lim →∞643 · ( + 1)(2 6 + 1) − 482 · (2+ 1) = lim →∞32 3 1 + 1 2 + 1 − 241 + 1 = 32 3 · 2 − 24 = − 8 3 (d) 04(2 − 3) = 1 − 2, where 1 is the area marked + and 2 is the area marked −. 27. = lim →∞ − =1 + − = lim →∞(− ) =1 1 + ( −2)2 =1 = lim →∞(− ) + ( −2)2 · (2+ 1) = ( − ) + lim →∞ ( −2)2 1 + 1 = ( − ) + 1 2( − )2 = ( − ) + 1 2 − 1 2 = ( − ) 1 2( + ) = 1 22 − 2 28. 2 = lim →∞ − =1 + − 2 = lim →∞ − =1 2 + 2 − + ( −2)2 2 = lim →∞( −3)3 =1 2 + 2(−2 )2 =1 + 2 (− ) =1 1 = lim →∞( −3)3 ( + 1) (2 6 + 1) + 2(−2 )2 (2+ 1) + 2 (− ) = lim →∞( −6)3 · 1 · 1 + 1 2 + 1 + ( − )2 · 1 · 1 + 1 + 2 ( − ) = ( − )3 3 + ( − )2 + 2 ( − ) = 3 − 32 + 32 − 3 3 + 2 − 22 + 3 + 2 − 3 = 3 3 − 3 3 − 2 + 2 + 2 − 2 = 3 − 3 3 29. () = √4 + 2, = 1, = 3, and ∆ = 3 − 1 = 2 . Using Theorem 4, we get ∗ = = 1 + ∆ = 1 + 2 , so 13 4 + 2 = lim →∞ = lim →∞ =1 4 + 1 + 22 · 2 . 30. () = 2 + 1 , = 2, = 5, and ∆ = 5 − 2 = 3 . Using Theorem 4, we get ∗ = = 2 + ∆ = 2 + 3 , so 25 2 + 1 = lim →∞ = lim →∞ =1 2 + 32 + 2 +1 3 · 3 . 31. ∆ = ( − 0) = and ∗ = = . 0 sin 5 = lim →∞ =1 (sin 5) = lim →∞ =1 sin 5 CAS = lim →∞ 1 cot5 2 CAS = 52 = 25 °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.18 ¤ CHAPTER 5 INTEGRALS 32. ∆ = (10 − 2) = 8 and ∗ = = 2 + 8. 210 6 = lim →∞ =1 2 + 868 = 8 lim →∞ 1 =1 2 + 86 CAS = 8 lim →∞ 1 · 6458,5936 + 164,0525 + 131,2084 − 27,7762 + 2048 215 CAS = 81,2497,984 = 9,9997,872 ≈ 1,428,5531 33. (a) Think of 02 () as the area of a trapezoid with bases 1 and 3 and height 2. The area of a trapezoid is = 1 2( + ), so 02 () = 1 2(1 + 3)2 = 4. (b) 05 () = 02 () trapezoid + 23 () rectangle + 35 () triangle = 1 2 (1 + 3)2 + 3 · 1 + 1 2 · 2 · 3 = 4 + 3 + 3 = 10 (c) 57 () is the negative of the area of the triangle with base 2 and height 3. 57 () = − 1 2 · 2 · 3 = −3. (d) 79 () is the negative of the area of a trapezoid with bases 3 and 2 and height 2, so it equals − 1 2 ( + ) = − 1 2(3 + 2)2 = −5. Thus, 09 () = 05 () + 57 () + 79 () = 10 + (−3) + (−5) = 2. 34. (a) 02 () = 1 2 · 4 · 2 = 4 [area of a triangle] (b) 26 () = − 1 2(2)2 = −2 [negative of the area of a semicircle] (c) 67 () = 1 2 · 1 · 1 = 1 2 [area of a triangle] 07 () = 02 () + 26 () + 67 () = 4 − 2 + 1 2 = 45 − 2 35. −21(1 − ) can be interpreted as the difference of the areas of the two shaded triangles; that is, 1 2(2)(2) − 1 2(1)(1) = 2 − 1 2 = 3 2 . 36. 09 1 3 − 2 can be interpreted as the difference of the areas of the two shaded triangles; that is, − 1 2(6)(2) + 1 2(3)(1) = −6 + 3 2 = − 9 2 . °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.2 THE DEFINITE INTEGRAL ¤ 19 37. −031 + √9 − 2 can be interpreted as the area under the graph of () = 1 + √9 − 2 between = −3 and = 0. This is equal to one-quarter the area of the circle with radius 3, plus the area of the rectangle, so −031 + √9 − 2 = 1 4 · 32 + 1 · 3 = 3 + 9 4. 38. −55 − √25 − 2 = −55 − −55 √25 − 2 . By symmetry, the value of the first integral is 0 since the shaded area above the -axis equals the shaded area below the -axis. The second integral can be interpreted as one half the area of a circle with radius 5; that is, 1 2(5)2 = 25 2 . Thus, the value of the original integral is 0 − 25 2 = − 25 2 . 39. −34 1 2 can be interpreted as the sum of the areas of the two shaded triangles; that is, 1 2(4)(2) + 1 2(3) 3 2 = 4 + 9 4 = 25 4 . 40. 01 |2 − 1| can be interpreted as the sum of the areas of the two shaded triangles; that is, 2 1 2 1 2 (1) = 1 2 . 41. 11 √1 + 4 = 0 since the limits of integration are equal. 42. 0 sin4 = − 0 sin4 [because we reversed the limits of integration] = − 0 sin4 [we can use any letter without changing the value of the integral] = − 3 8 [given value] 43. 01(5 − 62) = 01 5 − 601 2 = 5(1 − 0) − 6 1 3 = 5 − 2 = 3 44. 13 (2 − 1) = 213 − 13 1 = 2(3 − ) − 1(3 − 1) = 23 − 2 − 2 45. 13 + 2 = 13 · 2 = 2 13 = 2(3 − ) = 5 − 3 46. 02(2 cos − 5) = 02 2cos − 02 5 = 202 cos − 502 = 2(1) − 5(2)2 − 02 2 = 2 − 52 8 47. −22 () + 25 () − −−21 () = −52 () + −−12 () [by Property 5 and reversing limits] = −51 () [Property 5] °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.20 ¤ CHAPTER 5 INTEGRALS 48. 24 () + 48 () = 28 (), so 48 () = 28 () − 24 () = 73 − 59 = 14. 49. 09[2() + 3()] = 209 () + 309 () = 2(37) + 3(16) = 122 50. If () = 3 for for ≥ 3 3 , then 05 () can be interpreted as the area of the shaded region, which consists of a 5-by-3 rectangle surmounted by an isosceles right triangle whose legs have length 2. Thus, 05 () = 5(3) + 1 2(2)(2) = 17. 51. 03 () is clearly less than −1 and has the smallest value. The slope of the tangent line of at = 1, 0(1), has a value between −1 and 0, so it has the next smallest value. The largest value is 38 (), followed by 48 (), which has a value about 1 unit less than 38 (). Still positive, but with a smaller value than 48 (), is 08 (). Ordering these quantities from smallest to largest gives us 03 () 0(1) 08 () 48 () 38 () or B E A D C 52. (0) = 20 () = − 02 (), so (0) is negative, and similarly, so is (1). (3) and (4) are negative since they represent negatives of areas below the -axis. Since (2) = 22 () = 0 is the only non-negative value, choice C is the largest. 53. = −24[() + 2 + 5] = −24 () + 2−24 + −24 5 = 1 + 22 + 3 1 = −3 [area below -axis] + 3 − 3 = −3 2 = − 1 2(4)(4) [area of triangle, see figure] + 1 2(2)(2) = −8 + 2 = −6 3 = 5[2 − (−4)] = 5(6) = 30 Thus, = −3 + 2(−6) + 30 = 15. 54. Using Integral Comparison Property 8, ≤ () ≤ ⇒ (2 − 0) ≤ 02 () ≤ (2 − 0) ⇒ 2 ≤ 02 () ≤ 2. 55. 2 − 4 + 4 = ( − 2)2 ≥ 0 on [04], so 04(2 − 4 + 4) ≥ 0 [Property 6]. 56. 2 ≤ on [01] so √1 + 2 ≤ √1 + on [01]. Hence, 01 √1 + 2 ≤ 01 √1 + [Property 7]. 57. If −1 ≤ ≤ 1, then 0 ≤ 2 ≤ 1 and 1 ≤ 1 + 2 ≤ 2, so 1 ≤ √1 + 2 ≤ √2 and 1[1 − (−1)] ≤ −11 √1 + 2 ≤ √2[1 − (−1)] [Property 8]; that is, 2 ≤ −11 √1 + 2 ≤ 2√2. 58. If 6 ≤ ≤ 3 , then 1 2 ≤ sin ≤ √3 2 sin is increasing on 6 3 , so 1 2 3 − 6 ≤ 63 sin ≤ √23 3 − 6 [Property 8]; that is, 12 ≤ 63 sin ≤ √12 3 . °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.2 THE DEFINITE INTEGRAL ¤ 21 59. If 0 ≤ ≤ 1, then 0 ≤ 3 ≤ 1, so 0(1 − 0) ≤ 01 3 ≤ 1(1 − 0) [Property 8]; that is, 0 ≤ 01 3 ≤ 1. 60. If 0 ≤ ≤ 3, then 4 ≤ + 4 ≤ 7 and 1 7 ≤ + 4 1 ≤ 14, so 17(3 − 0) ≤ 03 + 4 1 ≤ 1 4(3 − 0) [Property 8]; that is, 3 7 ≤ 03 + 4 1 ≤ 34. 61. If 4 ≤ ≤ 3 , then 1 ≤ tan ≤ √3, so 1 3 − 4 ≤ 43 tan ≤ √3 3 − 4 or 12 ≤ 43 tan ≤ 12 √3. 62. Let () = 3 − 3 + 3 for 0 ≤ ≤ 2. Then 0() = 32 − 3 = 3( + 1)( − 1), so is decreasing on (01) and increasing on (12). has the absolute minimum value (1) = 1. Since (0) = 3 and (2) = 5, the absolute maximum value of is (2) = 5. Thus, 1 ≤ 3 − 3 + 3 ≤ 5 for in [0 2]. It follows from Property 8 that 1 · (2 − 0) ≤ 02 3 − 3 + 3 ≤ 5 · (2 − 0); that is, 2 ≤ 02 3 − 3 + 3 ≤ 10. 63. The only critical number of () = − on [02] is = 1. Since (0) = 0, (1) = −1 ≈ 0368, and (2) = 2−2 ≈ 0271, we know that the absolute minimum value of on [02] is 0, and the absolute maximum is −1. By Property 8 0 ≤ − ≤ −1 for 0 ≤ ≤ 2 ⇒ 0(2 − 0) ≤ 02 − ≤ −1(2 − 0) ⇒ 0 ≤ 02 − ≤ 2. 64. Let () = − 2sin for ≤ ≤ 2. Then 0() = 1 − 2cos and 0() = 0 ⇒ cos = 1 2 ⇒ = 53 . has the absolute maximum value 53 = 53 − 2sin 53 = 53 + √3 ≈ 697 since () = and (2) = 2 are both smaller than 697. Thus, ≤ () ≤ 53 + √3 ⇒ (2 − ) ≤ 2 () ≤ 53 + √3(2 − ); that is, 2 ≤ 2( − 2sin) ≤ 5 32 + √3. 65. √4 + 1 ≥ √4 = 2, so 13 √4 + 1 ≥ 13 2 = 1 3 33 − 13 = 26 3 . 66. 0 ≤ sin ≤ 1 for 0 ≤ ≤ 2 , so sin ≤ ⇒ 02 sin ≤ 02 = 1 2 2 2 − 02 = 82 . 67. sin √ for 1 ≤ ≤ 2 and arctan is an increasing function, so arctan(sin) arctan√ arctan, and hence, 12 arctan(sin) 12 arctan√ 12 arctan . Thus, 12 arctan has the largest value. 68. 2 √ for 0 ≤ 05 and cosine is a decreasing function on [005], so cos(2) cos√, and hence, 005 cos(2) 005 cos√ . Thus, 005 cos(2) is larger. 69. Using right endpoints as in the proof of Property 2, we calculate () = lim →∞ =1 ()∆ = lim →∞ =1 ()∆ = lim →∞ =1 ()∆ = (). 70. (a) Since − |()| ≤ () ≤ |()|, it follows from Property 7 that − |()| ≤ () ≤ |()| ⇒ () ≤ |()| Note that the definite integral is a real number, and so the following property applies: − ≤ ≤ ⇒ || ≤ for all real numbers and nonnegative numbers . °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.22 ¤ CHAPTER 5 INTEGRALS (b) 02 ()sin2 ≤ 02 |()sin2| [by part (a)] = 02 |()| |sin 2| ≤ 02 |()| by Property 7 since |sin 2| ≤ 1 ⇒ |()| |sin 2| ≤ |()|. 71. Suppose that is integrable on [01] that is, lim →∞ =1 (∗ )∆ exists for any choice of ∗ in [−1 ]. Let n denote a positive integer and divide the interval [01] into n equal subintervals 0 1 , 1 2 , , − 1 1. If we choose ∗ to be a rational number in the ith subinterval, then we obtain the Riemann sum =1 (∗ ) · 1 = 0, so lim →∞ =1 (∗ ) · 1 = lim →∞ 0 = 0. Now suppose we choose ∗ to be an irrational number. Then we get =1 (∗ ) · 1 = =1 1 · 1 = · 1 = 1 for each , so lim →∞ =1 (∗ ) · 1 = lim →∞ 1 = 1. Since the value of lim →∞ =1 (∗ )∆ depends on the choice of the sample points ∗ , the limit does not exist, and is not integrable on [0 1]. 72. Partition the interval [01] into n equal subintervals and choose ∗ 1 = 1 2 . Then with () = 1, =1 (∗ )∆ ≥ (∗ 1)∆ = 1 12 · 1 = Thus, =1 (∗ )∆ can be made arbitrarily large and hence, is not integrable on [0 1]. 73. lim →∞ =1 4 5 = lim →∞ =1 4 4 · 1 = lim →∞ =1 4 1 . At this point, we need to recognize the limit as being of the form lim →∞ =1 ()∆, where ∆ = (1 − 0) = 1, = 0 + ∆ = , and () = 4. Thus, the definite integral is 01 4 . 74. lim →∞ 1 =1 1 1 + ()2 = lim →∞ =1 1 1 + ()2 · 1 = lim →∞ =1 ()∆, where ∆ = (1 − 0) = 1, = 0 + ∆ = , and () = 1 1 + 2 . Thus, the definite integral is 01 1 +2 . 75. Choose = 1 + and ∗ = √−1 = 1 + − 11 + . Then 12 −2 = lim →∞ 1 =1 1 1 + − 11 + = lim →∞ =1 1 ( + − 1)( + ) = lim →∞ =1 +1 − 1 − +1 [by the hint] = lim →∞=0 −1 +1 − =1 +1 = lim →∞ 1 + + 1 1 + · · · + 21− 1 − + 1 1 + · · · + 21− 1 + 21 = lim →∞ 1 − 21 = lim →∞1 − 1 2 = 1 2 °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.DISCOVERY PROJECT AREA FUNCTIONS ¤ 23 DISCOVERY PROJECT Area Functions 1. (a) Area of trapezoid = 1 2(1 + 2) = 1 2(3 + 7)2 = 10 square units Or: Area of rectangle + area of triangle = + 1 2 = (2)(3) + 1 2(2)(4) = 10 square units (b) As in part (a), () = 1 2[3 + (2 + 1)]( − 1) = 1 2(2 + 4)( − 1) = ( + 2)( − 1) = 2 + − 2 square units (c) 0() = 2 + 1. This is the -coordinate of the point (2 + 1) on the given line. 2. (a) (b) () = −1 1 + 2 = −1 1 + −1 2 [Property 2] = 1[ − (−1)] + 3 − (−1)3 3 Exercise 5.2.28 Property 1 and = + 1 + 1 3 3 + 1 3 = 1 3 3 + + 4 3 (c) 0() = 2 + 1. This is the -coordinate of the point 1 + 2 on the given curve. (d) ( + ) − () is the area under the curve = 1 + 2 from = to = + . (e) An approximating rectangle is shown in the figure. It has height 1 + 2, width , and area (1 + 2), so ( + ) − () ≈ (1 + 2) ⇒ ( + ) − () ≈ 1 + 2. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.24 ¤ CHAPTER 5 INTEGRALS (f ) Part (e) says that the average rate of change of is approximately 1 + 2. As approaches 0, the quotient approaches the instantaneous rate of change—namely, 0(). So the result of part (c), 0() = 2 + 1, is geometrically plausible. 3. (a) () = cos(2) (b) () starts to decrease at that value of where cos2 changes from positive to negative; that is, at about = 125. (c) () = 0 cos(2). Using an integration command, we find that (0) = 0, (02) ≈ 0200, (04) ≈ 0399, (06) ≈ 0592, (08) ≈ 0768, (10) ≈ 0905, (12) ≈ 0974, (14) ≈ 0950, (16) ≈ 0826, (18) ≈ 0635, and (20) ≈ 0461. (d) We sketch the graph of 0 using the method of Example 1 in Section 2.8. The graphs of 0() and () look alike, so we guess that 0() = (). 4. In Problems 1 and 2, we showed that if () = (), then 0() = (), for the functions () = 2 + 1 and () = 1 + 2. In Problem 3 we guessed that the same is true for () = cos(2), based on visual evidence. So we conjecture that 0() = () for any continuous function . This turns out to be true and is proved in Section 5.3 (the Fundamental Theorem of Calculus). 5.3 The Fundamental Theorem of Calculus 1. One process undoes what the other one does. The precise version of this statement is given by the Fundamental Theorem of Calculus. See the statement of this theorem and the paragraph that follows it on page 398. 2. (a) () = 0 (), so (0) = 00 () = 0. (1) = 01 () = 1 2 · 1 · 1 [area of triangle] = 1 2. (2) = 02 () = 01 () + 12 () [below the t-axis] = 1 2 − 1 2 · 1 · 1 = 0. (3) = (2) + 23 () = 0 − 1 2 · 1 · 1 = − 1 2. (4) = (3) + 34 () = − 1 2 + 1 2 · 1 · 1 = 0. (5) = (4) + 45 () = 0 + 15 = 15. (6) = (5) + 56 () = 15 + 25 = 4. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.3 THE FUNDAMENTAL THEOREM OF CALCULUS ¤ 25 (b) (7) = (6) + 67 () ≈ 4 + 22 [estimate from the graph] = 62. (d) (c) The answers from part (a) and part (b) indicate that has a minimum at = 3 and a maximum at = 7. This makes sense from the graph of since we are subtracting area on 1 3 and adding area on 3 7. 3. (a) () = 0 (). (0) = 00 () = 0 (1) = 01 () = 1 · 2 = 2 [rectangle], (2) = 02 () = 01 () + 12 () = (1) + 12 () = 2 + 1 · 2 + 1 2 · 1 · 2 = 5 [rectangle plus triangle], (3) = 03 () = (2) + 23 () = 5 + 1 2 · 1 · 4 = 7, (6) = (3) + 36 () [the integral is negative since lies under the -axis] = 7 + − 1 2 · 2 · 2 + 1 · 2 = 7 − 4 = 3 (b) is increasing on (03) because as increases from 0 to 3, we keep adding more area. (d) (c) has a maximum value when we start subtracting area; that is, at = 3. 4. (a) () = 0 (), so (0) = 0 since the limits of integration are equal and (6) = 0 since the areas above and below the -axis are equal. (b) (1) is the area under the curve from 0 to 1, which includes two unit squares and about 80% to 90% of a third unit square, so (1) ≈ 28. Similarly, (2) ≈ 49 and (3) ≈ 57. Now (3) − (2) ≈ 08, so (4) ≈ (3) − 08 ≈ 49 by the symmetry of about = 3. Likewise, (5) ≈ 28. (c) As we go from = 0 to = 3, we are adding area, so increases on the interval (03). (d) increases on (03) and decreases on (36) [where we are subtracting area], so has a maximum value at = 3. (e) A graph of must have a maximum at = 3, be symmetric about = 3, and have zeros at = 0 and = 6. (f) If we sketch the graph of 0 by estimating slopes on the graph of (as in Section 2.8), we get a graph that looks like (as indicated by FTC1). °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.26 ¤ CHAPTER 5 INTEGRALS 5. (a) By FTC1 with () = 2 and = 1, () = 1 2 ⇒ 0() = () = 2. (b) Using FTC2, () = 1 2 = 1 3 3 1 = 1 3 3 − 1 3 ⇒ 0() = 2. 6. (a) By FTC1 with () = 2 + sin and = 0, () = 0(2 + sin) ⇒ 0() = () = 2 + sin. (b) Using FTC2, () = 0(2 + sin) = [2 − cos] 0 = (2 − cos) − (0 − 1) = 2 − cos + 1 ⇒ 0() = 2 − (−sin) + 0 = 2 + sin 7. () = √ + 3 and () = 0 √ + 3 , so by FTC1, 0() = () = √ + 3. 8. () = ln(1 + 2) and () = 1 ln(1 + 2) , so by FTC1, 0() = () = ln(1 + 2). 9. () = ( − 2)8 and () = 5( − 2)8 , so by FTC1, 0() = () = ( − 2)8. 10. () = √ + 1 and () = 0 √+ 1 , so by FTC1, 0() = () = √+ 1 . 11. () = 0 √1 + sec = − 0 √1 + sec ⇒ 0() = − 0 √1 + sec = −√1 + sec 12. () = 2 3 sin = − 2 3 sin ⇒ 0() = − 2 3 sin = −3 sin 13. Let = . Then = . Also, = , so 0() = 1 ln = 1 ln · = ln = (ln) · = . 14. Let = √. Then = 1 2√. Also, = , so 0() = 1√ 4+ 1 2 = 1 4+ 1 2 · = 4+ 1 2 = 2 + 1 2√1 = 2(√2 + 1) . 15. Let = 3 + 2. Then = 3. Also, = , so 0 = 13+2 1 + 3 = 1 1 + 3 · = 1 +3 = 1 + (3 3+ 2 + 2)3 · 3 = 1 + (3 3(3+ 2) + 2)3 16. Let = 4. Then = 43. Also, = , so 0 = 04 cos2 = 0 cos2 · = cos2 = cos2(4) · 43. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.3 THE FUNDAMENTAL THEOREM OF CALCULUS ¤ 27 17. Let = √. Then = 1 2√. Also, = , so 0 = √ 4 tan = − √4 tan · = −tan = −√tan√ · 2√1 = −12 tan√ 18. Let = sin. Then = cos. Also, = , so 0 = sin 1 1 + 2 = 1 1 + 2 · = − 1 1 + 2 · = −√1 + 2 cos = −1 + sin2 cos 19. 13(2 + 2 − 4) = 1 33 + 2 − 43 1 = (9 + 9 − 12) − 1 3 + 1 − 4 = 6 + 8 3 = 26 3 20. −11 100 = 101 1 1011 −1 = 101 1 − − 101 1 = 101 2 21. 02( 4 53 − 3 42 + 2 5) = 1 54 − 1 43 + 1 522 0 = 16 5 − 2 + 4 5 − 0 = 2 22. 01(1 − 83 + 167) = − 24 + 281 0 = (1 − 2 + 2) − 0 = 1 23. 19 √ = 19 12 = 3322 9 1 = 2 3329 1 = 2 3(932 − 132) = 2 3(27 − 1) = 52 3 24. 18 −23 = 1133 8 1 = 3138 1 = 3(813 − 113) = 3(2 − 1) = 3 25. 6 sin = −cos 6 = −cos − −cos 6 = −(−1) − −√32 = 1 + √32 26. −55 = 5 −5 = 5 − (−5) = 10 27. 01( + 2)( − 3) = 01(2 − − 6) = 1 33 − 1 22 − 61 0 = 1 3 − 1 2 − 6 − 0 = − 37 6 28. 04(4 − )√ = 04(4 − )12 = 04(412 − 32) = 8 332 − 2 5524 0 = 8 3(8) − 2 5(32) = 32015 −192 = 128 15 29. 14 2 + √2 = 14 √2 + √2 = 14(2−12 + 32) = 412 + 2 5524 1 = 4(2) + 2 5(32) − 4 + 2 5 = 8 + 64 5 − 4 − 2 5 = 82 5 30. −21(3 − 2)( + 1) = −21(32 + − 2) = 3 + 1 22 − 22 −1 = (8 + 2 − 4) − −1 + 1 2 + 2 = 6 − 3 2 = 9 2 31. 62 csc cot = −csc 2 6 = −csc 2 − −csc 6 = −1 − (−2) = 1 32. 43 csc2 = −cot 3 4 = −cot 3 − −cot 4 = −√13 − (−1) = 1 − √13 °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.28 ¤ CHAPTER 5 INTEGRALS 33. 01(1 + )3 = 01(1 + 3 + 32 + 3) = + 3 2 2 + 3 + 1 4 41 0 = 1 + 3 2 + 1 + 1 4 − 0 = 15 4 34. 03(2 sin − ) = −2cos − 3 0 = (−2cos 3 − 3) − (−2 − 1) = 3 − 2cos 3 − 3 35. 12 3 + 3 4 6 = 12 1 + 32 = ln|| + 32 1 = (ln 2 + 8) − (ln 1 + 1) = ln 2 + 7 36. 118 3 = 118 √3−12 = √321218 1 = 2√3(1812 − 112) = 2√3(3√2 − 1) 37. 01( + ) = + 1 +1 + 1 0 = + 1 1 + − (0 + 1) = + 1 1 + − 1 38. 01 cosh = sinh1 0 = sinh 1 − sinh 0 = sinh 1 or 1 2( − −1) 39. 1√√33 1 +82 = 8arctan√ 13√3 = 8 3 − 6 = 8 6 = 43 40. 13 3 − 22 2 − = 13 − 2 − 1 = 1 2 2 − 2 − ln||3 1 = 9 2 − 6 − ln 3 − 1 2 − 2 − 0 = −ln 3 41. 04 2 = ln 2 1 24 0 = ln 2 16 − ln 2 1 = ln 2 15 42. 112√2 √1 4− 2 = 4arcsin1 1 √ 2 2 = 4 4 − 6 = 412 = 3 43. If () = sin cos if if 0≤2 ≤ ≤ 2 then 0 () = 02 sin + 2 cos = −cos 0 2 + sin 2 = −cos 2 + cos 0 + sin − sin 2 = −0 + 1 + 0 − 1 = 0 Note that is integrable by Theorem 3 in Section 5.2. 44. If () = 24 − 2 if if 0− 2 ≤≤2≤ 0 then −22 () = −02 2 + 02(4 − 2) = 20 −2 + 4 − 1 3 32 0 = [0 − (−4)] + 16 3 − 0 = 28 3 Note that is integrable by Theorem 3 in Section 5.2. 45. Area = 04 √ = 04 12 = 2 3 324 0 = 2 3(8) − 0 = 16 3 °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.3 THE FUNDAMENTAL THEOREM OF CALCULUS ¤ 29 46. Area = 01 3 = 1 441 0 = 1 4 − 0 = 1 4 47. Area = −22(4 − 2) = 4 − 1 332 −2 = 8 − 8 3 − −8 + 8 3 = 32 3 48. Area = 02(2 − 2) = 2 − 1 332 0 = 4 − 8 3 − 0 = 4 3 49. From the graph, it appears that the area is about 60. The actual area is 027 13 = 3 44327 0 = 3 4 · 81 − 0 = 243 4 = 6075. This is 3 4 of the area of the viewing rectangle. 50. From the graph, it appears that the area is about 1 3. The actual area is 16 −4 = −−33 6 1 = 3−13 6 1 = −3 ·1216 + 13 = 215 648 ≈ 03318. 51. It appears that the area under the graph is about 2 3 of the area of the viewing rectangle, or about 2 3 ≈ 21. The actual area is 0 sin = [−cos ] 0 = (−cos) − (−cos 0) = −(−1) + 1 = 2. 52. Splitting up the region as shown, we estimate that the area under the graph is 3 + 1 43 · 3 ≈ 18. The actual area is 03 sec2 = [tan] 0 3 = √3 − 0 = √3 ≈ 173. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.30 ¤ CHAPTER 5 INTEGRALS 53. −21 3 = 1 442 −1 = 4 − 1 4 = 15 4 = 375 54. 26 cos = sin2 6 = 0 − 1 2 = − 1 2 55. () = −4 is not continuous on the interval [−21], so FTC2 cannot be applied. In fact, has an infinite discontinuity at = 0, so −12 −4 does not exist. 56. () = 4 3 is not continuous on the interval [−1 2], so FTC2 cannot be applied. In fact, has an infinite discontinuity at = 0, so −21 43 does not exist. 57. () = sec tan is not continuous on the interval [3 ], so FTC2 cannot be applied. In fact, has an infinite discontinuity at = 2, so 3 sec tan does not exist. 58. () = sec2 is not continuous on the interval [0 ], so FTC2 cannot be applied. In fact, has an infinite discontinuity at = 2, so 0 sec2 does not exist. 59. () = 23 2 2 − + 1 1 = 20 2 2 − + 1 1 + 03 2 2 − + 1 1 = − 02 2 2 − + 1 1 + 03 2 2 − + 1 1 ⇒ 0() = −(2)2 − 1 (2)2 + 1 · (2) + (3 (3))2 2 − + 1 1 · (3) = −2 · 442 2 − + 1 1 + 3 · 992 2 − + 1 1 60. () = 1−1+2 2 sin = 1−0 2 sin + 01+2 sin = − 01−2 sin + 01+2 sin ⇒ 0() = −(1 − 2)sin(1 − 2) · (1 − 2) + (1 + 2)sin(1 + 2) · (1 + 2) = 2(1 − 2)sin(1 − 2) + 2(1 + 2)sin(1 + 2) 61. () = 2 2 = 0 2 + 02 2 = − 0 2 + 02 2 ⇒ 0() = −2 + (2)2 · (2) = −2 + 24 °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.3 THE FUNDAMENTAL THEOREM OF CALCULUS ¤ 31 62. () = √2 arctan = √0 arctan + 02 arctan = − 0√ arctan + 02 arctan ⇒ 0() = −arctan√ · (√) + arctan 2 · (2) = −2√1 arctan√ + 2 arctan 2 63. = cos sin ln(1 + 2) = cos 0 ln(1 + 2) + 0sin ln(1 + 2) = − 0cos ln(1 + 2) + 0sin ln(1 + 2) ⇒ 0 = −ln(1 + 2 cos ) · cos + ln(1 + 2 sin) · sin = sin ln(1 + 2 cos) + cos ln(1 + 2 sin) 64. () = 0(1 − 2)2 is increasing when 0() = (1 − 2)2 is positive. Since 2 0, 0() 0 ⇔ 1 − 2 0 ⇔ || 1, so is increasing on (−11). 65. = 0 2 +2 + 2 ⇒ 0 = 2 +2 + 2 ⇒ 00 = (2 + + 2)(2) − 2(2 + 1) (2 + + 2)2 = 23 + 22 + 4 − 23 − 2 (2 + + 2)2 = 2 + 4 (2 + + 2)2 = ( + 4) (2 + + 2)2 . The curve is concave downward when 00 0; that is, on the interval (−4 0). 66. If () = 1 () , then by FTC1, 0() = (), and also, 00() = 0(). is concave downward where 00 is negative; that is, where 0 is negative. The given graph shows that is decreasing ( 0 0) on the interval (−11). 67. () = 2 2 ⇒ 0() = 2, so the slope at = 2 is 22 = 4. The -coordinate of the point on at = 2 is (2) = 22 2 = 0 since the limits are equal. An equation of the tangent line is − 0 = 4( − 2), or = 4 − 24. 68. () = 3 () ⇒ 0() = (). Since () = 0sin √1 + 2 , 00() = 0() = 1 + sin2 · cos, so 00( 6 ) = 1 + sin2( 6 ) · cos 6 = 1 + ( 1 2)2 · √23 = √25 · √23 = √415. 69. By FTC2, 14 0() = (4) − (1), so 17 = (4) − 12 ⇒ (4) = 17 + 12 = 29. 70. (a) erf() = √2 0 −2 ⇒ 0 −2 = √2 erf() By Property 5 of definite integrals in Section 5.2, 0 −2 = 0 −2 + −2 , so −2 = 0 −2 − 0 −2 = √2 erf() − √2 erf() = 1 2 √ [erf() − erf()]. (b) = 2 erf() ⇒ 0 = 22 erf() + 2 erf0() = 2 + 2 · √2 −2 [by FTC1] = 2 + √2 . 71. (a) The Fresnel function () = 0 sin 2 2 has local maximum values where 0 = 0() = sin 2 2 and 0 changes from positive to negative. For 0, this happens when 2 2 = (2 − 1) [odd multiples of ] ⇔ °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.32 ¤ CHAPTER 5 INTEGRALS 2 = 2(2 − 1) ⇔ = √4 − 2, any positive integer. For 0, 0 changes from positive to negative where 2 2 = 2 [even multiples of ] ⇔ 2 = 4 ⇔ = −2√. 0 does not change sign at = 0. (b) is concave upward on those intervals where 00() 0. Differentiating our expression for 0(), we get 00() = cos 2 22 2 = cos 2 2. For 0, 00() 0 where cos( 2 2) 0 ⇔ 0 2 2 2 or 2 − 1 2 2 2 2 + 1 2 , any integer ⇔ 0 1 or √4 − 1 √4 + 1, any positive integer. For 0, 00() 0 where cos( 2 2) 0 ⇔ 2 − 3 2 2 2 2 − 1 2 , any integer ⇔ 4 − 3 2 4 − 1 ⇔ √4 − 3 || √4 − 1 ⇒ √4 − 3 − √4 − 1 ⇒ −√4 − 3 −√4 − 1, so the intervals of upward concavity for 0 are −√4 − 1 −√4 − 3, any positive integer. To summarize: is concave upward on the intervals (01), −√3 −1, √3 √5, −√7 −√5, √7 3, . (c) In Maple, we use plot({int(sin(Pi*tˆ2/2),t=0..x),0.2},x=0..2);. Note that Maple recognizes the Fresnel function, calling it FresnelS(x). In Mathematica, we use Plot[{Integrate[Sin[Pi*tˆ2/2],{t,0,x}],0.2},{x,0,2}]. In Derive, we load the utility file FRESNEL and plot FRESNEL_SIN(x). From the graphs, we see that 0 sin 2 2 = 02 at ≈ 074. 72. (a) In Maple, we should start by setting si:=int(sin(t)/t,t=0..x);. In Mathematica, the command is si=Integrate[Sin[t]/t,{t,0,x}]. Note that both systems recognize this function; Maple calls it Si(x) and Mathematica calls it SinIntegral[x]. In Maple, the command to generate the graph is plot(si,x=-4*Pi..4*Pi);. In Mathematica, it is Plot[si,{x,-4*Pi,4*Pi}]. In Derive, we load the utility file EXP_INT and plot SI(x). (b) Si() has local maximum values where Si0() changes from positive to negative, passing through 0. From the Fundamental Theorem we know that Si0() = 0 sin = sin, so we must have sin = 0 for a maximum, and for 0 we must have = (2 − 1), any positive integer, for Si0 to be changing from positive to negative at . For 0, we must have = 2, any positive integer, for a maximum, since the denominator of Si0() is negative for 0. Thus, the local maxima occur at = , −2, 3, −4, 5, −6, . °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.3 THE FUNDAMENTAL THEOREM OF CALCULUS ¤ 33 (c) To find the first inflection point, we solve Si00() = cos − sin 2 = 0. We can see from the graph that the first inflection point lies somewhere between = 3 and = 5. Using a rootfinder gives the value ≈ 44934. To find the -coordinate of the inflection point, we evaluate Si(44934) ≈ 16556. So the coordinates of the first inflection point to the right of the origin are about (4493416556). Alternatively, we could graph 00() and estimate the first positive -value at which it changes sign. (d) It seems from the graph that the function has horizontal asymptotes at ≈ 15, with lim →±∞ Si() ≈ ±15 respectively. Using the limit command, we get lim →∞ Si() = 2 . Since Si() is an odd function, lim →−∞ Si() = − 2 . So Si() has the horizontal asymptotes = ± 2 . (e) We use the fsolve command in Maple (or FindRoot in Mathematica) to find that the solution is ≈ 11. Or, as in Exercise 65(c), we graph = Si() and = 1 on the same screen to see where they intersect. 73. (a) By FTC1, 0() = (). So 0() = () = 0 at = 1 357, and 9. has local maxima at = 1 and 5 (since = 0 changes from positive to negative there) and local minima at = 3 and 7. There is no local maximum or minimum at = 9, since is not defined for 9. (b) We can see from the graph that 01 13 35 57 79 . So (1) = 01 , (5) = 05 = (1) − 13 + 35 , and (9) = 09 = (5) − 57 + 79 . Thus, (1) (5) (9), and so the absolute maximum of () occurs at = 9. (c) is concave downward on those intervals where 00 0. But 0() = (), so 00() = 0(), which is negative on (approximately) 1 22, (4 6) and (89). So is concave downward on these intervals. (d) 74. (a) By FTC1, 0() = (). So 0() = () = 0 at = 2, 4, 6, 8, and 10. has local maxima at = 2 and 6 (since = 0 changes from positive to negative there) and local minima at = 4 and 8. There is no local maximum or minimum at = 10, since is not defined for 10. (b) We can see from the graph that 02 24 46 68 810 . So (2) = 02 , (6) = 06 = (2) − 24 + 46 , and (10) = 010 = (6) − 68 + 810 . Thus, (2) (6) (10), and so the absolute maximum of () occurs at = 2. (c) is concave downward on those intervals where 00 0. But 0() = (), so 00() = 0(), which is negative on (13), (57) and (910). So is concave downward on these intervals. (d) °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.34 ¤ CHAPTER 5 INTEGRALS 75. lim →∞ =1 45 + 2 = lim →∞ =1 44 + 1 = lim →∞ 1 − 0 =1 4 + = 01(4 + ) = 1 55 + 1 221 0 = 1 5 + 1 2 − 0 = 10 7 76. lim →∞ 1 1 + 2 + · · · + = lim →∞ 1 − 0 =1 = 01 √ = 2332 1 0 = 2 3 − 0 = 23 77. Suppose 0. Since is continuous on [ + ], the Extreme Value Theorem says that there are numbers and in [ + ] such that () = and () = , where and are the absolute minimum and maximum values of on [ + ]. By Property 8 of integrals, (−) ≤ + () ≤ (−); that is, ()(−) ≤ − + () ≤ ()(−). Since − 0, we can divide this inequality by −: () ≤ 1 + () ≤ (). By Equation 2, ( + ) − () = 1 + () for 6= 0, and hence () ≤ ( + ) − () ≤ (), which is Equation 3 in the case where 0. 78. (()) () = () () + () () [where is in the domain of ] = −() () + () () = −(()) 0() + (()) 0() = (())0 () − (())0() 79. (a) Let () = √ ⇒ 0() = 1(2√) 0 for 0 ⇒ is increasing on (0 ∞). If ≥ 0, then 3 ≥ 0, so 1 + 3 ≥ 1 and since is increasing, this means that 1 + 3 ≥ (1) ⇒ √1 + 3 ≥ 1 for ≥ 0. Next let () = 2 − ⇒ 0() = 2 − 1 ⇒ 0() 0 when ≥ 1. Thus, is increasing on (1 ∞). And since (1) = 0, () ≥ 0 when ≥ 1. Now let = √1 + 3, where ≥ 0. √1 + 3 ≥ 1 (from above) ⇒ ≥ 1 ⇒ () ≥ 0 ⇒ 1 + 3 − √1 + 3 ≥ 0 for ≥ 0. Therefore, 1 ≤ √1 + 3 ≤ 1 + 3 for ≥ 0. (b) From part (a) and Property 7: 01 1 ≤ 01 √1 + 3 ≤ 01(1 + 3) ⇔ 1 0 ≤ 01 √1 + 3 ≤ + 1 441 0 ⇔ 1 ≤ 01 √1 + 3 ≤ 1 + 1 4 = 125. 80. (a) For 0 ≤ ≤ 1 we have 2 ≤ Since () = cos is a decreasing function on [01],cos(2) ≥ cos (b) 6 1 so by part (a), cos(2) ≥ cos on [0 6]. Thus, 06 cos(2) ≥ 06 cos = sin 0 6 = sin(6) − sin 0 = 1 2 − 0 = 1 2. 81. 0 2 4 + 2 + 1 2 4 = 1 2 on [5 10], so 0 ≤ 510 4 +22 + 1 510 12 = −110 5 = −10 1 − −15 = 10 1 = 01 °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.3 THE FUNDAMENTAL THEOREM OF CALCULUS ¤ 35 82. (a) If 0, then () = 0 () = 0 0 = 0. If 0 ≤ ≤ 1, then () = 0 () = 0 = 1 22 0 = 1 22. If 1 ≤ 2, then () = 0 () = 01 () + 1 () = (1) + 1(2 − ) = 1 2 (1)2 + 2 − 1 22 1 = 1 2 + 2 − 1 22 − 2 − 1 2 = 2 − 1 22 − 1. If 2, then () = 0 () = (2) + 2 0 = 1 + 0 = 1. So () = 0 if 0 1 2 2 if 0 ≤ ≤ 1 2 − 1 22 − 1 if 1 ≤ 2 1 if 2 (b) (c) is not differentiable at its corners at = 0, 1, and 2. is differentiable on (−∞0), (01), (12) and (2 ∞). is differentiable on (−∞ ∞). 83. Using FTC1, we differentiate both sides of 6 + (2) = 2√ to get (2) = 2 2√1 ⇒ () = 32. To find , we substitute = in the original equation to obtain 6 + (2) = 2√ ⇒ 6 + 0 = 2√ ⇒ 3 = √ ⇒ = 9. 84. = 3 ⇒ 0 = 30 ⇒ [] 0 = 3 [] 0 ⇒ − 1 = 3( − 1) ⇒ = 3 − 2 ⇒ = ln(3 − 2) 85. (a) Let () = 0 (). Then, by FTC1, 0() = () = rate of depreciation, so () represents the loss in value over the interval [0 ]. (b) () = 1 + 0 () = +() represents the average expenditure per unit of during the interval [0 ], assuming that there has been only one overhaul during that time period. The company wants to minimize average expenditure. (c) () = 1 + 0 (). Using FTC1, we have 0() = −12 + 0 () + 1 (). 0() = 0 ⇒ () = + 0 () ⇒ () = 1 + 0 () = (). 86. (a) () = 1 0 [() + ()]. Using FTC1 and the Product Rule, we have 0() = 1 [() + ()] − 1 2 0 [() + ()]. Set 0() = 0: 1 [() + ()] − 12 0 [() + ()] = 0 ⇒ [() + ()] − 1 0 [() + ()] = 0 ⇒ [() + ()] − () = 0 ⇒ () = () + (). °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.36 ¤ CHAPTER 5 INTEGRALS (b) For 0 ≤ ≤ 30, we have () = 015 − 450 = 15 − 900 2 0 = 15 − 900 2. So () = ⇒ 15 − 900 2 = ⇒ 60 − 2 = 900 ⇒ 2 − 60 + 900 = 0 ⇒ ( − 30)2 = 0 ⇒ = 30. So the length of time is 30 months. (c) () = 1 015 − 450 + 12,9002 = 1 15 − 900 2 + 38,7003 0 = 1 15 − 900 2 + 38,7003 = 15 − 900 + 38,7002 ⇒ 0() = − 900 + 19,350 = 0 when 1 19,350 = 1 900 ⇒ = 215. (215) = 15 − 900 (215) + 38,700(215)2 ≈ 005472 , (0) = 15 ≈ 006667 , and (30) = 15 − 900 (30) + 38,700(30)2 ≈ 005659 , so the absolute minimum is (215) ≈ 005472 . (d) As in part (c), we have () = 15 − 900 + 38,7002, so () = () + () ⇔ 15 − 900 + 38,7002 = 15 − 450 + 12,9002 ⇔ 212,1900 − 38,1700 = 450 1 − 900 1 ⇔ = 2138 900 ,700 = 43 2 = 215. This is the value of that we obtained as the critical number of in part (c), so we have verified the result of (a) in this case. 5.4 Indefinite Integrals and the Net Change Theorem 1. −√1 + 2 + = −(1 + 2)12 + = − · 1 2(1 + 2)−12((2 )2) − (1 + 2)12 · 1 + 0 = − (1 + 2)−12 2 − (1 + 2) 2 = − −1 (1 + 2)122 = 1 2√1 + 2 2. 21 + 41 sin 2 + = 1 2 + 14 cos 2 · 2 + 0 = 12 + 12 cos 2 = 1 2 + 1 22cos2 − 1 = 1 2 + cos2 − 1 2 = cos2 3. (tan − + ) = sec2 − 1 + 0 = tan2 4. 1522 (3 − 2)( + )32 + = 1522 (3 − 2) 3 2( + )12() + ( + )32(3) + 0 = 2 152 (3)( + )12 (3 − 2) 1 2 + ( + ) = 2 5 ( + )125 2 = √ + °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.4 INDEFINITE INTEGRALS AND THE NET CHANGE THEOREM ¤ 37 5. (13 + 725) = 21323 + 37535 + = 21323 + 235 + 6. √4 5 = 54 = 4 994 + 7. 5 + 2 32 + 3 43 = 5 + 2 3 · 1 33 + 3 4 · 1 44 + = 5 + 2 93 + 16 3 4 + 8. (6 − 25 − 3 + 2 7) = 1 77 − 2 · 1 66 − 1 44 + 2 7 + = 1 77 − 1 36 − 1 44 + 2 7 + 9. ( + 4)(2 + 1) = (22 + 9 + 4) = 2 33 + 9 22 + 4 + = 2 33 + 922 + 4 + 10. √(2 + 3 + 2) = 12(2 + 3 + 2) = (52 + 332 + 212) = 2 7 72 + 3 · 2 552 + 2 · 2 332 + = 2 772 + 6 552 + 4 332 + 11. 1 + √ + = 1 + √ + = 1 + −12 + 1 = ln|| + 212 + + = ln|| + 2√ + + 12. 2 + 1 + 21+ 1 = 33 + + tan−1 + 13. (sin + sinh) = −cos + cosh + 14. 1 + 2 = 1 + 22+ 2 = (−2 + 2−1 + 1) = −−1 + 2 ln|| + + = −1 + 2 ln|| + + 15. (2 + tan2 ) = [2 + (sec2 − 1)] = (1 + sec2 ) = + tan + 16. sec(sec + tan) = (sec2 + sec tan) = tan + sec + 17. 2(1 + 5) = (2 + 2 · 5) = (2 + 10) = ln 2 2 + ln 10 10 + 18. sin 2 sin = 2sinsin cos = 2cos = 2 sin + 19. cos + 1 2 = sin + 1 42 + . The members of the family in the figure correspond to = −5, 0, 5, and 10. 20. ( − 22) = − 2 33 + The members of the family in the figure correspond to = −5, 0, 2, and 5. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.38 ¤ CHAPTER 5 INTEGRALS 21. −32(2 − 3) = 1 33 − 33 −2 = (9 − 9) − − 8 3 + 6 = 8 3 − 18 3 = − 10 3 22. 12(43 − 32 + 2) = 4 − 3 + 22 1 = (16 − 8 + 4) − (1 − 1 + 1) = 12 − 1 = 11 23. −02 1 24 + 1 43 − = 10 1 5 + 16 1 4 − 1 220 −2 = 0 − 10 1 (−32) + 16 1 (16) − 1 2(4) = − − 16 5 + 1 − 2 = 21 5 24. 03(1 + 62 − 104) = + 23 − 253 0 = (3 + 54 − 486) − 0 = −429 25. 02(2 − 3)(42 + 1) = 02(83 − 122 + 2 − 3) = 24 − 43 + 2 − 32 0 = (32 − 32 + 4 − 6) − 0 = −2 26. −11 (1 − )2 = −11 (1 − 2 + 2) = −11( − 22 + 3) = 1 22 − 2 33 + 1 441 −1 = 1 2 − 2 3 + 1 4 − 1 2 + 2 3 + 1 4 = − 4 3 27. 0(5 + 3 sin) = [5 − 3cos ] 0 = [5 − 3(−1)] − [5(1) − 3(1)] = 5 + 1 28. 12 12 − 43 = 12(−2 − 4−3) = −−11 − 4−−22 2 1 = −1 + 22 2 1 = −12 + 12 − (−1 + 2) = −1 29. 14 4 + 6 √ = 14 √4 + √6 = 14(4−12 + 612) = 812 + 4324 1 = (16 + 32) − (8 + 4) = 36 30. 01 1 +42 = 4arctan1 0 = 4 arctan 1 − 4arctan0 = 44 − 4(0) = 31. 01 √3 + √4 = 01(43 + 54) = 3 773 + 4 9941 0 = 3 7 + 4 9 − 0 = 55 63 32. 14 √−2 = 14 √2 − 2 = 14(−32 − −1) = −2−12 − ln|| 4 1 = −√2 − ln||4 1 = (−1 − ln 4) − (−2 − ln 1) = 1 − ln 4 33. 12 2 − 2 = 1 42 − 2ln||2 1 = (1 − 2ln2) − 14 − 2ln1 = 34 − 2ln2 34. 01(5 − 5) = 522 − ln 5 5 1 0 = 5 2 − ln 5 5 − 0 − ln 5 1 = 52 − ln 5 4 35. 01(10 + 10) = 11 11 + ln 10 10 1 0 = 11 1 + ln 10 10 − 0 + ln 10 1 = 11 1 + ln 10 9 36. 04 sec tan = sec 0 4 = sec 4 − sec 0 = √2 − 1 37. 04 1 + cos cos2 2 = 04cos12 + cos cos2 2 = 04(sec2 + 1) = tan + 0 4 = tan 4 + 4 − (0 + 0) = 1 + 4 °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.4 INDEFINITE INTEGRALS AND THE NET CHANGE THEOREM ¤ 39 38. 03 sin + sin sec2 tan2 = 03 sin (1 + tan sec2 2 ) = 03 sinsec sec 2 2 = 03 sin = −cos 0 3 = − 1 2 − (−1) = 1 2 39. 18 2 + √3 2 = 18 223 + 23 = 18(2−23 + 13) = 2 · 313 + 3 4 438 1 = (12 + 12) − 6 + 3 4 = 69 4 40. −10 10 sinh2+ cosh = −10 10 − −2 2 + + − 2 = −10 10 2 = −10 10 2 = 210 −10 = 20 − (−20) = 40 41. 0√32 √1 − 2 = arcsin√ 0 32 = arcsin√32 − arcsin 0 = 3 − 0 = 3 42. 12 ( −21)3 = 12 3 − 322+ 3 − 1 = 12 − 3 + 3 − 12 = 1 2 2 − 3 + 3 ln|| + 12 1 = 2 − 6 + 3 ln 2 + 1 2 − 1 2 − 3 + 0 + 1 = 3 ln 2 − 2 43. 01√3 2 4 − − 1 1 = 01√3 (2 + 1)( 2 −21− 1) = 01√3 2 1+ 1 = arctan1 0√3 = arctan1√3 − arctan 0 = 6 − 0 = 6 44. |2 − 1| = 2−(2−1− 1) if if 2 2 − − 1 1 ≥ 0 0 = 2 1−−21 if if ≥ 1 2 1 2 Thus, 02 |2 − 1| = 012(1 − 2) + 122(2 − 1) = − 21 02 + 2 − 2 12 = ( 1 2 − 1 4) − 0 + (4 − 2) − ( 1 4 − 1 2) = 1 4 + 2 − (− 1 4) = 5 2 45. −21 ( − 2||) = −01[ − 2(−)] + 02[ − 2()] = −01 3 + 02(−) = 3 1 2 20 −1 − 1 2 22 0 = 30 − 1 2 − (2 − 0) = − 7 2 = −35 46. 032 |sin| = 0 sin + 32(−sin) = − cos 0 + cos 3 2 = [1 − (−1)] + [0 − (−1)] = 2 + 1 = 3 47. The graph shows that = 1 − 2 − 54 has -intercepts at = ≈ −086 and at = ≈ 042. So the area of the region that lies under the curve and above the -axis is (1 − 2 − 54) = − 2 − 5 = ( − 2 − 5) − ( − 2 − 5) ≈ 136 48. The graph shows that = (2 + 1)−1 − 4 has -intercepts at = ≈ −087 and at = ≈ 087. So the area of the region that lies under the curve and above the -axis is (2 + 1)−1 − 4 = tan−1 − 1 5 5 = tan−1 − 1 5 5 − tan−1 − 1 5 5 ≈ 123 °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.40 ¤ CHAPTER 5 INTEGRALS 49. = 022 − 2 = 2 − 1 332 0 = 4 − 8 3 − 0 = 4 3 50. = √4 ⇒ = 4, so = 01 4 = 1 551 0 = 1 5 . 51. If 0() is the rate of change of weight in pounds per year, then () represents the weight in pounds of the child at age . We know from the Net Change Theorem that 510 0() = (10) − (5), so the integral represents the increase in the child’s weight (in pounds) between the ages of 5 and 10. 52. () = 0() = () − () by the Net Change Theorem, so it represents the change in the charge from time = to = . 53. Since () is the rate at which oil leaks, we can write () = − 0(), where () is the volume of oil at time . [Note that the minus sign is needed because is decreasing, so 0() is negative, but () is positive.] Thus, by the Net Change Theorem, 0120 () = − 0120 0() = −[ (120) − (0)] = (0) − (120), which is the number of gallons of oil that leaked from the tank in the first two hours (120 minutes). 54. By the Net Change Theorem, 015 0() = (15) − (0) = (15) − 100 represents the increase in the bee population in 15 weeks. So 100 + 015 0() = (15) represents the total bee population after 15 weeks. 55. By the Net Change Theorem, 1000 5000 0() = (5000) − (1000), so it represents the increase in revenue when production is increased from 1000 units to 5000 units. 56. The slope of the trail is the rate of change of the elevation , so () = 0(). By the Net Change Theorem, 35 () = 35 0() = (5) − (3) is the change in the elevation between = 3 miles and = 5 miles from the start of the trail. 57. In general, the unit of measurement for () is the product of the unit for () and the unit for . Since () is measured in newtons and is measured in meters, the units for 0100 () are newton-meters (or joules). (A newton-meter is abbreviated N·m.) 58. The units for () are pounds per foot and the units for are feet, so the units for are pounds per foot per foot, denoted (lbft)ft. The unit of measurement for 28 () is the product of pounds per foot and feet; that is, pounds. 59. (a) Displacement = 03(3 − 5) = 3 22 − 53 0 = 27 2 − 15 = − 3 2 m (b) Distance traveled = 03 |3 − 5| = 053(5 − 3) + 533(3 − 5) = 5 − 3 225 03 + 3 22 − 53 53 = 25 3 − 3 2 · 25 9 + 27 2 − 15 − 3 2 · 25 9 − 25 3 = 41 6 m 60. (a) Displacement = 24(2 − 2 − 3) = 1 33 − 2 − 34 2 = 64 3 − 16 − 12 − 8 3 − 4 − 6 = 2 3 m (b) () = 2 − 2 − 3 = ( + 1)( − 3), so () 0 for −1 3, but on the interval [24], () 0 for 2 ≤ 3. Distance traveled = 24 2 − 2 − 3 = 23 −(2 − 2 − 3) + 34(2 − 2 − 3) = − 1 33 + 2 + 33 2 + 1 33 − 2 − 34 3 = (−9 + 9 + 9) − − 8 3 + 4 + 6 + 64 3 − 16 − 12 − (9 − 9 − 9) = 4 m °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.4 INDEFINITE INTEGRALS AND THE NET CHANGE THEOREM ¤ 41 61. (a) 0() = () = + 4 ⇒ () = 1 2 2 + 4 + ⇒ (0) = = 5 ⇒ () = 1 2 2 + 4 + 5 ms (b) Distance traveled = 010 |()| = 010 1 2 2 + 4 + 5 = 010 1 2 2 + 4 + 5 = 1 6 3 + 22 + 510 0 = 500 3 + 200 + 50 = 416 2 3 m 62. (a) 0() = () = 2 + 3 ⇒ () = 2 + 3 + ⇒ (0) = = −4 ⇒ () = 2 + 3 − 4 (b) Distance traveled = 03 2 + 3 − 4 = 03 |( + 4)( − 1)| = 01−2 − 3 + 4 + 132 + 3 − 4 = − 1 3 3 − 3 2 2 + 41 0 + 1 3 3 + 3 2 2 − 43 1 = − 1 3 − 3 2 + 4 + 9 + 27 2 − 12 − 1 3 + 3 2 − 4 = 89 6 m 63. Since 0() = (), = 04 () = 049 + 2√ = 9 + 4 3 324 0 = 36 + 32 3 − 0 = 140 3 = 46 2 3 kg. 64. By the Net Change Theorem, the amount of water that flows from the tank during the first 10 minutes is 010 () = 010(200 − 4) = 200 − 2210 0 = (2000 − 200) − 0 = 1800 liters. 65. Let be the position of the car. We know from Equation 2 that (100) − (0) = 0100 (). We use the Midpoint Rule for 0 ≤ ≤ 100 with = 5. Note that the length of each of the five time intervals is 20 seconds = 3600 20 hour = 180 1 hour. So the distance traveled is 0100 () ≈ 180 1 [(10) + (30) + (50) + (70) + (90)] = 180 1 (38 + 58 + 51 + 53 + 47) = 247 180 ≈ 14 miles. 66. (a) By the Net Change Theorem, the total amount spewed into the atmosphere is (6) − (0) = 06 () = (6) since (0) = 0. The rate () is positive, so is an increasing function. Thus, an upper estimate for (6) is 6 and a lower estimate for (6) is 6. ∆ = − = 6 − 0 6 = 1. 6 = 6 =1 ()∆ = 10 + 24 + 36 + 46 + 54 + 60 = 230 tonnes. 6 = 6 =1 (−1)∆ = 6 + (0) − (6) = 230 + 2 − 60 = 172 tonnes. (b) ∆ = − = 6 − 0 3 = 2. (6) ≈ 3 = 2[(1) + (3) + (5)] = 2(10 + 36 + 54) = 2(100) = 200 tonnes. 67. From the Net Change Theorem, the increase in cost if the production level is raised from 2000 yards to 4000 yards is (4000) − (2000) = 2000 4000 0(). 2000 4000 0() = 2000 4000(3 − 001 + 00000062) = 3 − 00052 + 000000234000 2000 = 60,000 − 2,000 = $58,000 68. By the Net Change Theorem, the amount of water after four days is 25,000 + 04 () ≈ 25,000 + 4 = 25,000 + 4 −4 0 [(05) + (15) + (25) + (35)] ≈ 25,000 + [1500 + 1770 + 740 + (−690)] = 28,320 liters °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.42 ¤ CHAPTER 5 INTEGRALS 69. To use the Midpoint Rule, we’ll use the midpoint of each of three 2-second intervals. (6) − (0) = 06 () ≈ [(1) + (3) + (5)] 6 −3 0 ≈ (06 + 10 + 93)(2) = 398 fts 70. Use the midpoint of each of four 2-day intervals. Let = 0 correspond to July 18 and note that the inflow rate, (), is in ft3s. Amount of water = 08 () ≈ [(1) + (3) + (5) + (7)] 8 −4 0 ≈ [6401 + 4249 + 3821 + 2628](2) = 34,198. Now multiply by the number of seconds in a day, 24 · 602, to get 2,954,707,200 ft3. 71. Let () denote the bacteria population at time (in hours). By the Net Change Theorem, (1) − (0) = 01 0() = 01(1000 · 2) = 1000 ln 2 2 1 0 = 1000 ln 2 (21 − 20) = 1000 ln 2 ≈ 1443 Thus, the population after one hour is 4000 + 1443 = 5443. 72. Let () denote the number of megabits transmitted at time (in hours) [note that () is measured in megabits/second]. By the Net Change Theorem and the Midpoint Rule, (8) − (0) = 08 3600() ≈ 3600 · 8−4 0[(1) + (3) + (5) + (7)] ≈ 7200(032 + 050 + 056 + 083) = 7200(221) = 15,912 megabits 73. Power is the rate of change of energy with respect to time; that is, () = 0(). By the Net Change Theorem and the Midpoint Rule, (24) − (0) = 024 () ≈ 2412 − 0[(1) + (3) + (5) + · · · + (21) + (23)] ≈ 2(16,900 + 16,400 + 17,000 + 19,800 + 20,700 + 21,200 + 20,500 + 20,500 + 21,700 + 22,300 + 21,700 + 18,900) = 2(237,600) = 475,200 Thus, the energy used on that day was approximately 4.75 × 105 megawatt-hours. 74. (a) From Exercise 4.1.74(a), () = 0001463 − 0115532 + 2498169 − 2126872. (b) (125) − (0) = 0125 () = 00003654 − 0038513 + 124908452 − 2126872125 0 ≈ 206,407 ft 5.5 The Substitution Rule 1. Let = 2. Then = 2 and = 1 2 , so cos 2 = cos 1 2 = 1 2 sin + = 1 2 sin 2 + . 2. Let = −2. Then = −2 and = − 1 2 , so −2 = − 1 2 = − 1 2 + = − 1 2 −2 + . 3. Let = 3 + 1. Then = 32 and 2 = 1 3 , so 23 + 1 = √ 1 3 = 13 3322 + = 13 · 2332 + = 2 9(3 + 1)32 + . 4. Let = sin. Then = cos , so sin2 cos = 2 = 1 3 3 + = 1 3 sin3 + . °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.5 THE SUBSTITUTION RULE ¤ 43 5. Let = 4 − 5. Then = 43 and 3 = 1 4 , so 4−3 5 = 114 = 1 4 ln|| + = 1 4 ln 4 − 5 + . 6. Let = 2 + 1. Then = 2 and = 1 2 , so √2 + 1 = √ 1 2 = 1 2 · 2 332 + = 1 3(2 + 1)32 + . 7. Let = 1 − 2. Then = −2 and = − 1 2 , so √1 − 2 = √ − 1 2 = − 1 2 · 2 332 + = − 1 3(1 − 2)32 + . 8. Let = 3. Then = 32 and 2 = 1 3 , so 23 = 1 3 = 1 3 + = 1 33 + . 9. Let = 1 − 2. Then = −2 and = − 1 2 , so (1 − 2)9 = 9 − 1 2 = − 1 2 · 10 1 10 + = − 20 1 (1 − 2)10 + . 10. Let = 1 + cos. Then = −sin and sin = −, so sin √1 + cos = √(−) = − 2 332 + = − 2 3(1 + cos)32 + . 11. Let = 2 . Then = 2 and = 2 , so cos 2 = cos 2 = 2 sin + = 2 sin 2 + . 12. Let = 2. Then = 2 and = 1 2 , so sec2 2 = sec2 1 2 = 1 2 tan + = 1 2 tan 2 + . 13. Let = 5 − 3. Then = −3 and = − 1 3 , so 5 −3 = 1 − 1 3 = − 1 3 ln|| + = − 1 3 ln|5 − 3| + . 14. Let = 4 − 3. Then = −32 and 2 = − 1 3 , so 2(4 − 3)23 = 23 − 1 3 = − 1 3 · 3 553 + = − 1 5(4 − 3)53 + . 15. Let = cos. Then = −sin and sin = −, so cos3 sin = 3(−) = − 1 44 + = − 1 4 cos4 + . 16. Let = −5. Then = −5 and = − 1 5 , so −5 = − 1 5 = − 1 5 + = − 1 5−5 + . 17. Let = 1 − . Then = − and = −, so (1 −)2 = 12 (−) = − −2 = −(−−1) + = 1 + = 1 −1 + . 18. Let = √. Then = 1 2√ and 2 = √1 , so sin√√ = sin(2) = −2cos + = −2cos √ + . 19. Let = 3 + 3. Then = (3 + 32) = 3( + 2), so √3 ++ 2 3 = 1 3 1 2 = 13 −12 = 1 3 · 212 + = 2 33 + 3 + . °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.44 ¤ CHAPTER 5 INTEGRALS 20. Let = 3 + 1. Then = 32 and 1 3 = 2 + , so 3+ 1 2 = 1 1 3 = 1 3 ln|| + = 1 3 ln|3 + 1| + . 21. Let = ln. Then = , so (ln)2 = 2 = 1 33 + = 1 3(ln)3 + . 22. Let = cos. Then = −sin and − = sin , so sin sin(cos) = sin(−) = (−cos )(−1) + = cos(cos) + . 23. Let = tan. Then = sec2 , so sec2 tan3 = 3 = 1 44 + = 1 4 tan4 + . 24. Let = + 2. Then = and = − 2, so √ + 2 = ( − 2)√ = (32 − 212) = 2 552 − 2 · 2 332 + = 2 5( + 2)52 − 4 3( + 2)32 + . 25. Let = 1 + . Then = , so √1 + = √ = 2 332 + = 2 3(1 + )32 + . Or: Let = √1 + . Then 2 = 1 + and 2 = , so √1 + = · 2 = 2 33 + = 2 3(1 + )32 + . 26. Let = + . Then = and = (1), so + = (1) = 1 1 = 1 ln|| + = 1 ln| + | + . 27. Let = 3 + 3. Then = (32 + 3) and 1 3 = (2 + 1), so (2 + 1)(3 + 3)4 = 4 ( 1 3 ) = 1 3 · 1 55 + = 15 1 (3 + 3)5 + . 28. Let = cos. Then = −sin and sin = −, so cos sin = (−) = − + = −cos + . 29. Let = 5. Then = 5 ln5 and 5 = 1 ln5 , so 5 sin(5) = sin ln5 1 = −ln5 1 cos + = −ln5 1 cos(5) + . 30. Let = tan. Then = sec2 , so tan sec22 = 12 = −2 = −1−1 + = −tan 1 + = −cot + . Or: tan sec22 = cos12 · cos sin22 = csc2 = −cot + 31. Let = arctan. Then = 1 2 + 1 , so (arctan 2 + 1)2 = 2 = 1 33 + = 1 3(arctan)3 + . 32. Let = 2 + 4. Then = 2 and = 1 2 , so 2+ 4 = 1 12 = 1 2 ln|| + = 1 2 ln 2 + 4 + = 12 ln(2 + 4) + [since 2 + 4 0]. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.5 THE SUBSTITUTION RULE ¤ 45 33. Let = 1 + 5. Then = 5 and = 1 5 , so cos(1 + 5) = cos 1 5 = 1 5 sin + = 1 5 sin(1 + 5) + . 34. Let = . Then = − 2 and 12 = −1 , so cos( 2 ) = cos−1 = −1 sin + = −1 sin + 35. Let = cot. Then = −csc2 and csc2 = −, so √cot csc2 = √(−) = −3322 + = − 2 3(cot)32 + . 36. Let = 2 + 3. Then = 2 ln 2 and 2 = 1 ln 2 , so 22+ 3 = 1ln 2 1 = ln 2 1 ln|| + = ln 2 1 ln(2 + 3) + . 37. Let = sinh. Then = cosh , so sinh2 cosh = 2 = 1 33 + = 1 3 sinh3 + . 38. Let = 1 + tan. Then = sec2 , so cos2 √ 1 + tan = √sec 1 + tan 2 = √ = −12 = 1122 + = 2√1 + tan + . 39. 1 + cos sin 22 = 2 1 + cos sincos 2 = 2. Let = cos. Then = −sin , so 2 = −2 1 + 2 = −2 · 1 2 ln(1 + 2) + = −ln(1 + 2) + = −ln(1 + cos2 ) + . Or: Let = 1 + cos2 . 40. Let = cos. Then = −sin and sin = −, so 1 + cos sin2 = 1 + − 2 = −tan−1 + = −tan−1(cos) + . 41. cot = cos sin . Let = sin. Then = cos , so cot = 1 = ln|| + = ln|sin| + . 42. Let = ln. Then = 1 , so cos(ln ) = cos = sin + = sin(ln) + . 43. Let = sin−1 . Then = √1 1− 2 , so √1 − 2 sin−1 = 1 = ln|| + = ln sin−1 + . 44. Let = 2. Then = 2 , so 1 +4 = 1 + 1 2 2 = 1 2 tan−1 + = 1 2 tan−1(2) + . 45. Let = 1 + 2. Then = 2 , so 1 + 1 +2 = 1 +12 + 1 +2 = tan−1 + 1 2 = tan−1 + 1 2 ln|| + = tan−1 + 1 2 ln 1 + 2 + = tan−1 + 1 2 ln1 + 2 + [since 1 + 2 0]. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.46 ¤ CHAPTER 5 INTEGRALS 46. Let = 2 + . Then = , = − 2, and 2 = ( − 2)2, so 2√2 + = ( − 2)2√ = (2 − 4 + 4)12 = (52 − 432 + 412) = 2 7 72 − 8 552 + 8 332 + = 2 7(2 + )72 − 8 5(2 + )52 + 8 3(2 + )32 + 47. Let = 2 + 5. Then = 2 and = 1 2( − 5), so (2 + 5)8 = 1 2( − 5)8 ( 1 2 ) = 1 4 (9 − 58) = 1 4 ( 10 1 10 − 5 99) + = 40 1 (2 + 5)10 − 36 5 (2 + 5)9 + 48. Let = 2 + 1 [so 2 = − 1]. Then = 2 and = 1 2 , so 3 √2 + 1 = 2 √2 + 1 = ( − 1)√ 1 2 = 1 2 (32 − 12) = 1 2 2 552 − 2 332 + = 1 5(2 + 1)52 − 1 3(2 + 1)32 + . Or: Let = √2 + 1. Then 2 = 2 + 1 ⇒ 2 = 2 ⇒ = , so 3 √2 + 1 = 2 √2 + 1 = (2 − 1) · = (4 − 2) = 1 5 5 − 1 33 + = 1 5(2 + 1)52 − 1 3(2 + 1)32 + . Note: This answer can be written as 1 15 √2 + 1(34 + 2 − 2) + . 49. () = (2 − 1)3. = 2 − 1 ⇒ = 2 so (2 − 1)3 = 3 1 2 = 1 84 + = 1 8(2 − 1)4 + Where is positive (negative), is increasing (decreasing). Where changes from negative to positive (positive to negative), has a local minimum (maximum). 50. () = tan2 sec2 . = tan ⇒ = sec2 , so tan2 sec2 = 2 = 1 33 + = 1 3 tan3 + Note that is positive and is increasing. At = 0, = 0 and has a horizontal tangent. 51. () = cos sin. = cos ⇒ = −sin , so cos sin = (−) = − + = −cos + Note that at = , changes from positive to negative and has a local maximum. Also, both and are periodic with period 2, so at = 0 and at = 2, changes from negative to positive and has a local minimum. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.5 THE SUBSTITUTION RULE ¤ 47 52. () = sincos4. = cos ⇒ = −sin , so sincos4 = 4 (−) = − 1 5 5 + = − 1 5 cos5 + Note that at = , changes from positive to negative and has a local maximum. Also, both and are periodic with period 2, so at = 0 and at = 2, changes from negative to positive and has a local minimum. 53. Let = 2 , so = 2 . When = 0, = 0; when = 1, = 2 . Thus, 01 cos(2) = 02 cos 2 = 2 [sin] 0 2 = 2 sin 2 − sin 0 = 2 (1 − 0) = 2 54. Let = 3 − 1, so = 3. When = 0, = −1; when = 1, = 2. Thus, 01(3 − 1)50 = −21 50 1 3 = 1 3 51 1 512 −1 = 153 1 251 − (−1)51 = 153 1 (251 + 1) 55. Let = 1 + 7, so = 7. When = 0, = 1; when = 1, = 8. Thus, 01 √3 1 + 7 = 18 13( 1 7 ) = 1 7 3 4 438 1 = 28 3 (843 − 143) = 28 3 (16 − 1) = 45 28 56. Let = 5 + 1, so = 5. When = 0, = 1; when = 3, = 16. Thus, 03 5 + 1 = 116 115 = 1 5 ln|| 16 1 = 15(ln 16 − ln 1) = 1 5 ln 16. 57. Let = cos, so = −sin . When = 0, = 1; when = 6 , = √32. Thus, 06 cos sin2 = 1√32 12 (−) = 1 √ 1 32 = √23 − 1. 58. Let = 1 2 , so = 1 2 . When = 3 , = 6 ; when = 23 , = 3 . Thus, 2 3 3 csc2 1 2 = 63 csc2 (2) = 2−cot 3 6 = −2cot 3 − cot 6 = −2√13 − √3 = −2 1 3√3 − √3 = 4 3√3 59. Let = 1, so = −12 . When = 1, = 1; when = 2, = 1 2 . Thus, 12 1 2 = 112 (−) = − 1 12 = −(12 − ) = − √. 60. Let = −2, so = −2 . When = 0, = 0; when = 1, = −1. Thus, 01 −2 = 0−1 − 1 2 = − 1 2 − 0 1 = − 1 2 −1 − 0 = 1 2(1 − 1). 61. − 44(3 + 4 tan) = 0 by Theorem 7(b), since () = 3 + 4 tan is an odd function. 62. Let = sin, so = cos . When = 0, = 0; when = 2 , = 1. Thus, 02 cos sin(sin) = 01 sin = −cos1 0 = −(cos 1 − 1) = 1 − cos 1. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.48 ¤ CHAPTER 5 INTEGRALS 63. Let = 1 + 2, so = 2. When = 0, = 1; when = 13, = 27. Thus, 013 3 (1 + 2 )2 = 127 −23 1 2 = 1 2 · 31327 1 = 3 2(3 − 1) = 3. 64. Assume 0. Let = 2 − 2, so = −2 . When = 0, = 2; when = , = 0. Thus, 0 √2 − 2 = 02 12− 1 2 = 1 2 02 12 = 1 2 · 2 332 02 = 1 33. 65. Let = 2 + 2, so = 2 and = 1 2 . When = 0, = 2; when = , = 22. Thus, 0 2 + 2 = 222 12 1 2 = 1 2 2 3322 22 = 1 3322 22 = 1 3(22)32 − (2)32 = 1 32√2 − 13 66. − 33 4 sin = 0 by Theorem 7(b), since () = 4 sin is an odd function. 67. Let = − 1, so + 1 = and = . When = 1, = 0; when = 2, = 1. Thus, 12 √ − 1 = 01( + 1)√ = 01(32 + 12) = 2 552 + 2 3321 0 = 2 5 + 2 3 = 16 15. 68. Let = 1 + 2, so = 1 2( − 1) and = 2. When = 0, = 1; when = 4, = 9. Thus, 04 √1 + 2 = 19 1 2(√− 1) 2 = 1 4 19(12 − −12) = 1 4 2 332 − 2129 1 = 1 4 · 2 332 − 3129 1 = 1 6 [(27 − 9) − (1 − 3)] = 20 6 = 10 3 69. Let = ln, so = . When = , = 1; when = 4; = 4. Thus, 4 √ln = 14 −12 = 2124 1 = 2(2 − 1) = 2. 70. Let = ( − 1)2, so = 2( − 1). When = 0, = 1; when = 2, = 1. Thus, 02( − 1)(−1)2 = 11 1 2 = 0 since the limits are equal. 71. Let = + , so = ( + 1) . When = 0, = 1; when = 1, = + 1. Thus, 01 + 1 + = 1+1 1 = ln|| 1+1 = ln| + 1| − ln|1| = ln( + 1). 72. Let = 2 − , so = 2 . When = 0 = −; when = 2 , = − . Thus, 02 sin2 − = −− sin 2 = 2 −cos −− = −2[cos( − ) − cos(−)] = − 2 (−cos − cos) = − 2 (−2cos) = cos °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.5 THE SUBSTITUTION RULE ¤ 49 73. Let = 1 + √, so = 1 2√ ⇒ 2√ = ⇒ 2( − 1) = . When = 0, = 1; when = 1, = 2. Thus, 01 (1 + √)4 = 12 14 · [2( − 1)] = 212 13 − 14 = 2−212 + 313 2 1 = 2− 1 8 + 24 1 − − 1 2 + 1 3 = 2 12 1 = 1 6 74. If () = sin √3 , then (−) = sin √3 − = sin(−√3 ) = −sin √3 = −(), so is an odd function. Now = −32 sin √3 = −22 sin √3 + 23 sin √3 = 1 + 2. 1 = 0 by Theorem 7(b). To estimate 2, note that 2 ≤ ≤ 3 ⇒ √3 2 ≤ √3 ≤ √3 3 [≈ 144] ⇒ 0 ≤ √3 ≤ 2 [≈ 157] ⇒ sin 0 ≤ sin √3 ≤ sin 2 [since sine is increasing on this interval] ⇒ 0 ≤ sin √3 ≤ 1. By comparison property 8, 0(3 − 2) ≤ 2 ≤ 1(3 − 2) ⇒ 0 ≤ 2 ≤ 1 ⇒ 0 ≤ ≤ 1. 75. From the graph, it appears that the area under the curve is about 1 + a little more than 1 2 · 1 · 07, or about 14. The exact area is given by = 01 √2 + 1. Let = 2 + 1, so = 2. The limits change to 2 · 0 + 1 = 1 and 2 · 1 + 1 = 3, and = 13 √ 1 2 = 1 2 2 3323 1 = 1 33√3 − 1 = √3 − 1 3 ≈ 1399. 76. From the graph, it appears that the area under the curve is almost 1 2 · · 26, or about 4. The exact area is given by = 0(2 sin − sin 2) = −2cos 0 − 0 sin 2 = −2(−1 − 1) − 0 = 4 Note: 0 sin2 = 0 since it is clear from the graph of = sin2 that 2 sin2 = − 02 sin2 . 77. First write the integral as a sum of two integrals: = −22( + 3)√4 − 2 = 1 + 2 = −22 √4 − 2 + −22 3√4 − 2 . 1 = 0 by Theorem 7(b), since () = √4 − 2 is an odd function and we are integrating from = −2 to = 2. We interpret 2 as three times the area of a semicircle with radius 2, so = 0 + 3 · 1 2 · 22 = 6. 78. Let = 2. Then = 2 and the limits are unchanged (02 = 0 and 12 = 1), so = 01 √1 − 4 = 1 2 01 √1 − 2 . But this integral can be interpreted as the area of a quarter-circle with radius 1. So = 1 2 · 1 4 · 12 = 1 8. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.50 ¤ CHAPTER 5 INTEGRALS 79. First Figure Let = √, so = 2 and = 2 . When = 0, = 0; when = 1, = 1. Thus, 1 = 01 √ = 01 (2 ) = 201 . Second Figure 2 = 01 2 = 201 . Third Figure Let = sin, so = cos . When = 0, = 0; when = 2 , = 1. Thus, 3 = 02 sin sin 2 = 02 sin (2 sin cos) = 01 (2 ) = 201 . Since 1 = 2 = 3, all three areas are equal. 80. Let = 12 . Then = 12 and 024() = 02485 − 018 cos12 = 02(85 − 018 cos)12 = 12 85 − 018 sin2 0 = 12 [(85 · 2 − 0) − (0 − 0)] = 2040 kcal 81. The rate is measured in liters per minute. Integrating from = 0 minutes to = 60 minutes will give us the total amount of oil that leaks out (in liters) during the first hour. 060 () = 060 100−001 [ = −001, = −001] = 1000−06(−100) = −10,000− 0 06 = −10,000(−06 − 1) ≈ 45119 ≈ 4512 liters 82. Let () = with = 450268 and = 112567, and () = population after hours. Since () = 0(), 03 () = (3) − (0) is the total change in the population after three hours. Since we start with 400 bacteria, the population will be (3) = 400 + 03 () = 400 + 03 = 400 + 3 0 = 400 + 3 − 1 ≈ 400 + 11,313 = 11,713 bacteria 83. The volume of inhaled air in the lungs at time is () = 0 () = 0 1 2 sin25 = 025 1 2 sin 25 substitute = 25 , = 25 = 5 4 −cos2 05 = 45 −cos25 + 1 = 45 1 − cos25 liters 84. The rate is measured in kilograms per year. Integrating from = 0 years (2000) to = 20 years (2020) will give us the net change in biomass from 2000 to 2020. 020 (1 + 5 60,000−−0066)2 = 61+5−12 60,000 2 − 1 3 = 1 + 5 = −3−0−606 = 20,0001+5 6 −12 = 1 + 5 20,000 −12 − 20,6000 ≈ 16,666 Thus, the predicted biomass for the year 2020 is approximately 25,000 + 16,666 = 41,666 kg. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.SECTION 5.5 THE SUBSTITUTION RULE ¤ 51 85. 030 () = 030 0− = 0 1−30 (−) == −− − = 0− 1−30 = 0(−−30 + 1) The integral 030 () represents the total amount of urea removed from the blood in the first 30 minutes of dialysis. 86. Number of calculators = (4) − (2) = 24 50001 − 100( + 10)−2 = 5000 + 100( + 10)−14 2 = 50004 + 100 14 − 2 + 100 12 ≈ 4048 87. Let = 2. Then = 2, so 02 (2) = 04 () 1 2 = 1 2 04 () = 1 2(10) = 5. 88. Let = 2. Then = 2 , so 03 (2) = 09 () 1 2 = 1 2 09 () = 1 2(4) = 2. 89. Let = −. Then = −, so (−) = −− ()(−) = −− () = −− () From the diagram, we see that the equality follows from the fact that we are reflecting the graph of , and the limits of integration, about the -axis. 90. Let = + . Then = , so ( + ) = ++ () = ++ () From the diagram, we see that the equality follows from the fact that we are translating the graph of , and the limits of integration, by a distance . 91. Let = 1 − . Then = 1 − and = −, so 01 (1 − ) = 10 (1 − ) (−) = 01 (1 − ) = 01 (1 − ) . 92. Let = − . Then = −. When = , = 0 and when = 0, = . So 0 (sin) = − 0( − )(sin( − )) = 0( − )(sin) = 0 (sin) − 0 (sin) = 0 (sin) − 0 (sin) ⇒ 20 (sin) = 0 (sin) ⇒ 0 (sin) = 2 0 (sin). 93. sin 1 + cos2 = · sin 2 − sin2 = (sin), where () = 2 − 2 . By Exercise 92, 0 1 + cos sin2 = 0 (sin) = 2 0 (sin) = 2 0 1 + cos sin2 Let = cos. Then = −sin . When = , = −1 and when = 0, = 1. So 2 0 1 + cos sin2 = −2 1−1 1 +2 = 2 −11 1 +2 = 2 tan−1 1 −1 = 2 [tan−1 1 − tan−1(−1)] = 2 4 − −4 = 42 °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.52 ¤ CHAPTER 5 INTEGRALS 94. (a) 02 (cos) = 02 sin 2 − = 2 − , = − = 0 2 (sin)(−) = 02 (sin) = 02 (sin) Continuity of is needed in order to apply the substitution rule for definite integrals. (b) In part (a), take () = 2, so 02 cos2 = 02 sin2 . Now 02 cos2 + 02 sin2 = 02(cos2 + sin2 ) = 02 1 = 0 2 = 2 , so 202 cos2 = 2 ⇒ 02 cos2 = 4 = 02 sin2 . 5 Review 1. True by Property 2 of the Integral in Section 5.2. 2. False. Try = 0, = 2, () = () = 1 as a counterexample. 3. True by Property 3 of the Integral in Section 5.2. 4. False. You can’t take a variable outside the integral sign. For example, using () = 1 on [01], 01 () = 01 = 1 2 21 0 = 1 2 (a constant) while 01 1 = []1 0 = · 1 = (a variable). 5. False. For example, let () = 2. Then 01 √2 = 01 = 1 2, but 01 2 = 1 3 = √13. 6. True by the Net Change Theorem. 7. True by Comparison Property 7 of the Integral in Section 5.2. 8. False. For example, let = 0, = 1, () = 3, () = . () () for each in (0 1), but 0() = 0 1 = 0() for ∈ (01). 9. True. The integrand is an odd function that is continuous on [−1 1]. 10. True. −552 + + = −552 + + −55 = 2052 + + 0 [because 2 + is even and is odd] 11. False. For example, the function = || is continuous on R, but has no derivative at = 0. 12. True by FTC1. 13. True by Property 5 of Integrals. 14. False. For example, 01 − 1 2 = 1 2 2 − 1 2 1 0 = 1 2 − 1 2 − (0 − 0) = 0, but () = − 1 2 6= 0. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.CHAPTER 5 REVIEW ¤ 53 15. False. () is a constant, so () = 0, not () [unless () = 0]. Compare the given statement carefully with FTC1, in which the upper limit in the integral is . 16. False. See the paragraph before Note 4 and Figure 4 in Section 5.2, and notice that = − 3 0 for 1 ≤ 2. 17. False. The function () = 14 is not bounded on the interval [−21]. It has an infinite discontinuity at = 0, so it is not integrable on the interval. (If the integral were to exist, a positive value would be expected, by Comparison Property 6 of Integrals.) 18. False. For example, if () = 10 if if 0−≤1 ≤ ≤ 1 0 then has a jump discontinuity at 0, but −11 () exists and is equal to 1. 1. (a) 6 = 6 =1 (−1)∆ [∆ = 6 −6 0 = 1] = (0) · 1 + (1) · 1 + (2) · 1 + (3) · 1 + (4) · 1 + (5) · 1 ≈ 2 + 35 + 4 + 2 + (−1) + (−25) = 8 The Riemann sum represents the sum of the areas of the four rectangles above the -axis minus the sum of the areas of the two rectangles below the -axis. (b) 6 = 6 =1 ()∆ [∆ = 6 −6 0 = 1] = (1) · 1 + (2) · 1 + (3) · 1 + (4) · 1 + (5) · 1 + (6) · 1 = (05) + (15) + (25) + (35) + (45) + (55) ≈ 3 + 39 + 34 + 03 + (−2) + (−29) = 57 The Riemann sum represents the sum of the areas of the four rectangles above the -axis minus the sum of the areas of the two rectangles below the -axis. 2. (a) () = 2 − and ∆ = 2 −4 0 = 05 ⇒ 4 = 05(05) + 05(1) + 05(15) + 05(2) = 05(−025 + 0 + 075 + 2) = 125 The Riemann sum represents the sum of the areas of the two rectangles above the x-axis minus the area of the rectangle below the x-axis. (The second rectangle vanishes.) °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.54 ¤ CHAPTER 5 INTEGRALS (b) 022 − = lim →∞ =1 () ∆ [∆ = 2 and = 2] = lim →∞ =1422 − 22 = lim →∞ 2 42 =1 2 − 2 =1 = lim →∞83 · ( + 1)(2 6 + 1) − 42 · (2+ 1) = lim →∞4 3 · + 1 · 2+ 1 − 2 · + 1 = lim →∞4 31 + 1 2 + 1 − 21 + 1 = 4 3 · 1 · 2 − 2 · 1 = 2 3 (c) 022 − = 1 33 − 1 222 0 = 8 3 − 2 = 2 3 (d) 022 − = 1 − 2, where 1 and 2 are the areas shown in the diagram. 3. 01 + √1 − 2 = 01 + 01 √1 − 2 = 1 + 2. 1 can be interpreted as the area of the triangle shown in the figure and 2 can be interpreted as the area of the quarter-circle. Area = 1 2(1)(1) + 1 4()(1)2 = 1 2 + 4 . 4. On [0 ], lim →∞ =1 sin ∆ = 0 sin = [−cos] 0 = −(−1) − (−1) = 2. 5. 06 () = 04 () + 46 () ⇒ 10 = 7 + 46 () ⇒ 46 () = 10 − 7 = 3 6. (a) 15( + 25) = lim →∞ =1 ()∆ ∆ = 5 − 1 = 4 , = 1 + 4 = lim →∞ =1 1 + 4 + 21 + 45 · 4 CAS = lim →∞ 13054 + 312633+ 20802 − 256 · 4 = 5220 (b) 15( + 25) = 1 22 + 2 665 1 = 25 2 + 15,3625 − 1 2 + 1 3 = 12 + 5208 = 5220 7. First note that either or must be the graph of 0 (), since 00 () = 0, and (0) 6= 0. Now notice that 0 when is increasing, and that 0 when is increasing. It follows that is the graph of (), is the graph of 0(), and is the graph of 0 (). °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.CHAPTER 5 REVIEW ¤ 55 8. (a) By the Net Change Theorem (FTC2), 01 arctan = arctan 1 0 = 4 − 1 (b) 01 arctan = 0 since this is the derivative of a constant. (c) By FTC1, 0 arctan = arctan . 9. (4) = 04 () = 01 () + 12 () + 23 () + 34 () = − 1 2 · 1 · 2 area of triangle, below -axis + 1 2 · 1 · 2 + 1 · 2 + 1 2 · 1 · 2 = 3 By FTC1, 0() = (), so 0(4) = (4) = 0. 10. () = 0 () ⇒ 0() = () [by FTC1] ⇒ 00() = 0(), so 00(4) = 0(4) = −2, which is the slope of the line segment at = 4. 11. 1283 + 32 = 8 · 1 44 + 3 · 1 332 1 = 24 + 32 1 = 2 · 24 + 23 − (2 + 1) = 40 − 3 = 37 12. 0 4 − 8 + 7 = 1 55 − 42 + 7 0 = 1 5 5 − 4 2 + 7 − 0 = 1 5 5 − 4 2 + 7 13. 011 − 9 = − 10 1 101 0 = 1 − 10 1 − 0 = 10 9 14. Let = 1 − , so = − and = −. When = 0, = 1; when = 1, = 0. Thus, 01(1 − )9 = 10 9(−) = 01 9 = 10 1 101 0 = 10 1 (1 − 0) = 10 1 . 15. 19 √ − 22 = 19(−12 − 2) = 212 − 29 1 = (6 − 81) − (2 − 1) = −76 16. 01 (√4 + 1)2 = 01(12 + 214 + 1) = 2 332 + 8 554 + 1 0 = 2 3 + 8 5 + 1 − 0 = 49 15 17. Let = 2 + 1, so = 2 and = 1 2 . When = 0, = 1; when = 1, = 2. Thus, 01 (2 + 1)5 = 12 5 1 2 = 1 2 1 662 1 = 12 1 (64 − 1) = 63 12 = 21 4 . 18. Let = 1 + 3, so = 32 and 2 = 1 3 . When = 0, = 1; when = 2, = 9. Thus, 02 21 + 3 = 19 12 1 3 = 1 3 2 3329 1 = 2 9(27 − 1) = 52 9 . 19. 15 ( −4)2 does not exist because the function () = ( −14)2 has an infinite discontinuity at = 4; that is, is discontinuous on the interval [15]. 20. Let = 3, so = 3 . When = 0, = 1; when = 1, = 3. Thus, 01 sin(3) = 03 sin31 = 31 −cos3 0 = −31(−1 − 1) = 32 . °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.56 ¤ CHAPTER 5 INTEGRALS 21. Let = 3, so = 32 . When = 0, = 0; when = 1, = 1. Thus, 01 2 cos(3) = 01 cos 1 3 = 1 3 sin1 0 = 1 3(sin 1 − 0) = 1 3 sin 1. 22. −11 1 + sin2 = 0 by Theorem 5.5.7(b), since () = 1 + sin2 is an odd function. 23. − 44 2 + cos 4 tan = 0 by Theorem 5.5.7(b), since () = 2 + cos 4 tan is an odd function. 24. Let = , so = When = 0 = 1; when = 1 = . Thus, 01 1 +2 = 1 1 +12 = arctan 1 = arctan − arctan 1 = arctan − 4 . 25. 1 − 2 = 1 − 12 = 12 − 2 + 1 = −1 − 2ln|| + + 26. 110 2− 4 does not exist because the function () = 2− 4 has an infinite discontinuity at = 2; that is, is discontinuous on the interval [110]. 27. Let = 2 + 4. Then = (2 + 4) = 2( + 2), so √2+ 2 + 4 = −12 1 2 = 1 2 · 212 + = √ + = 2 + 4 + . 28. Let = 1 + cot. Then = −csc2 , so 1 + cot csc2 = 1 (−) = −ln|| + = −ln|1 + cot| + . 29. Let = sin. Then = cos , so sin cos = 1 = 1 · 1 22 + = 21(sin)2 + . 30. Let = cos. Then = −sin , so sin cos(cos) = − cos = −sin + = −sin(cos) + . 31. Let = √. Then = 2√, so √√ = 2 = 2 + = 2√ + . 32. Let = ln. Then = 1 , so sin(ln ) = sin = −cos + = −cos(ln) + . 33. Let = ln(cos). Then = −sin cos = −tan , so tan ln(cos) = − = − 1 22 + = − 1 2[ln(cos )]2 + . 34. Let = 2. Then = 2 , so √1− 4 = 12 √1 − 2 = 1 2 sin−1 + = 1 2 sin−12 + . 35. Let = 1 + 4. Then = 43 , so 1 +34 = 14 1 = 1 4 ln|| + = 1 4 ln1 + 4 + . 36. Let = 1 + 4. Then = 4, so sinh(1 + 4) = 1 4 sinh = 1 4 cosh + = 1 4 cosh(1 + 4) + . °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.CHAPTER 5 REVIEW ¤ 57 37. Let = 1 + sec. Then = sec tan , so sec 1 + sec tan = 1 + sec 1 (sec tan ) = 1 = ln|| + = ln|1 + sec| + . 38. Let = 1 + tan, so = sec2 . When = 0, = 1; when = 4 , = 2. Thus, 04(1 + tan)3 sec2 = 12 3 = 1 442 1 = 1 424 − 14 = 1 4(16 − 1) = 15 4 . 39. Since 2 − 4 0 for 0 ≤ 2 and 2 − 4 0 for 2 ≤ 3, we have 2 − 4 = −(2 − 4) = 4 − 2 for 0 ≤ 2 and 2 − 4 = 2 − 4 for 2 ≤ 3. Thus, 03 2 − 4 = 02(4 − 2) + 23(2 − 4) = 4 − 33 2 0 + 33 − 43 2 = 8 − 8 3 − 0 + (9 − 12) − 8 3 − 8 = 16 3 − 3 + 16 3 = 32 3 − 9 3 = 23 3 40. Since √ − 1 0 for 0 ≤ 1 and √ − 1 0 for 1 ≤ 4, we have √ − 1 = − √ − 1 = 1 − √ for 0 ≤ 1 and √ − 1 = √ − 1 for 1 ≤ 4. Thus, 04 √ − 1 = 01 1 − √ + 14 √ − 1 = − 2 3321 0 + 2 332 − 4 1 = 1 − 2 3 − 0 + 16 3 − 4 − 2 3 − 1 = 1 3 + 16 3 − 4 + 1 3 = 6 − 4 = 2 41. Let = 1 + sin. Then = cos , so √cos 1 + sin = −12 = 212 + = 2√1 + sin + . 42. Let = 2 + 1. Then 2 = − 1 and = 1 2 , so √23+ 1 = (√−1) 1 2 = 12 (12 − −12) = 1 2 2 332 − 212 + = 1 3(2 + 1)32 − (2 + 1)12 + = 1 3 (2 + 1)12 (2 + 1) − 3 + = 1 3√2 + 1 (2 − 2) + 43. From the graph, it appears that the area under the curve = √ between = 0 and = 4 is somewhat less than half the area of an 8 × 4 rectangle, so perhaps about 13 or 14. To find the exact value, we evaluate 04 √ = 04 32 = 2 5524 0 = 2 5(4)52 = 64 5 = 128. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.58 ¤ CHAPTER 5 INTEGRALS 44. From the graph, it seems as though 02 cos2 sin is equal to 0. To evaluate the integral, let = cos ⇒ = −sin . Thus, = 11 2 (−) = 0. 45. () = 0 1 +23 ⇒ 0() = 0 1 +23 = 1 +23 46. () = 1√ + sin = − 1 √ + sin ⇒ 0() = − 1√ + sin = −√ + sin 47. Let = 4. Then = 43. Also, = , so 0() = 04 cos(2) = 0 cos(2) · = cos(2) = 43 cos(8). 48. Let = sin Then = cos Also, = so 0() = 1sin 1 1 +− 2 4 = 1 1 1 +− 2 4 · = 11 + − 2 4 · = 11 + sin − sin2 4 · cos = 1 + sin cos3 4 49. = √ = √1 + 1 = − 1√ + 1 ⇒ = − 1√ + 1 . Let = √. Then 1√ = 1 = 1 = · 2√1 = √√ · 2√1 = 2√ , so = − √ 2 + = 2 − √ 2 . 50. = 23+1 sin4 = 20 sin4 + 03+1 sin4 = 03+1 sin4 − 02 sin4 ⇒ 0 = sin(3 + 1)4 · (3 + 1) − sin(2)4 · (2) = 3 sin(3 + 1)4 − 2sin(2)4 51. If 1 ≤ ≤ 3, then √12 + 3 ≤ √2 + 3 ≤ √32 + 3 ⇒ 2 ≤ √2 + 3 ≤ 2√3, so 2(3 − 1) ≤ 13 √2 + 3 ≤ 2√3(3 − 1); that is, 4 ≤ 13 √2 + 3 ≤ 4√3. 52. If 3 ≤ ≤ 5, then 4 ≤ + 1 ≤ 6 and 1 6 ≤ 1 + 1 ≤ 1 4, so 1 6 (5 − 3) ≤ 35 + 1 1 ≤ 14(5 − 3); that is, 1 3 ≤ 35 + 1 1 ≤ 12. 53. 0 ≤ ≤ 1 ⇒ 0 ≤ cos ≤ 1 ⇒ 2 cos ≤ 2 ⇒ 01 2 cos ≤ 01 2 = 1 3 31 0 = 1 3 [Property 7]. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.CHAPTER 5 REVIEW ¤ 59 54. On the interval 4 2 , is increasing and sin is decreasing, so sin is decreasing. Therefore, the largest value of sin on 4 2 is sin( 44) = √ 242 = 2√ 2. By Property 8 with = 2√ 2 we get 42 sin ≤ 2√ 2 2 − 4 = √22. 55. cos ≤ 1 ⇒ cos ≤ ⇒ 01 cos ≤ 01 = []1 0 = − 1 56. For 0 ≤ ≤ 1, 0 ≤ sin−1 ≤ 2 , so 01 sin−1 ≤ 01 2 = 4 21 0 = 4 . 57. ∆ = (3 − 0)6 = 1 2, so the endpoints are 0, 1 2, 1, 3 2, 2, 5 2, and 3, and the midpoints are 1 4, 3 4, 5 4, 7 4, 9 4, and 11 4 . The Midpoint Rule gives 03 sin(3) ≈ 6 =1 ()∆ = 1 2 sin 1 4 3 + sin 3 4 3 + sin 5 4 3 + sin 7 4 3 + sin 9 4 3 + sin 11 4 3 ≈ 0280981. 58. (a) Displacement = 052 − = 1 33 − 1 225 0 = 125 3 − 25 2 = 175 6 = 2916 meters (b) Distance traveled = 05 2 − = 05 |( − 1)| = 01 − 2 + 152 − = 1 22 − 1 331 0 + 1 33 − 1 225 1 = 1 2 − 1 3 − 0 + 125 3 − 25 2 − 1 3 − 1 2 = 177 6 = 295 meters 59. Note that () = 0(), where () = the number of barrels of oil consumed up to time . So, by the Net Change Theorem, 08 () = (8) − (0) represents the number of barrels of oil consumed from Jan. 1, 2000, through Jan. 1, 2008. 60. Distance covered = 050 () ≈ 5 = 505− 0[(05) + (15) + (25) + (35) + (45)] = 1(467 + 886 + 1022 + 1067 + 1081) = 4523 m 61. We use the Midpoint Rule with = 6 and ∆ = 246− 0 = 4. The increase in the bee population was 024 () ≈ 6 = 4[(2) + (6) + (10) + (14) + (18) + (22)] ≈ 4[50 + 1000 + 7000 + 8550 + 1350 + 150] = 4(18,100) = 72,400 62. 1 = 1 2 = 1 2(2)(2) = 2, 2 = 1 2 = 1 2(1)(1) = 1 2, and since = −√1 − 2 for 0 ≤ ≤ 1 represents a quarter-circle with radius 1, 3 = 1 42 = 1 4(1)2 = 4 . So −13 () = 1 − 2 − 3 = 2 − 1 2 − 4 = 1 4(6 − ) 63. Let = 2 sin. Then = 2 cos and when = 0, = 0; when = 2 , = 2. Thus, 02 (2 sin)cos = 02 () 1 2 = 1 2 02 () = 1 2 02 () = 1 2(6) = 3. 64. (a) is increasing on those intervals where 0 is positive. By the Fundamental Theorem of Calculus, 0() = 0 cos 2 2 = cos 2 2. This is positive when 2 2 is in the interval 2 − 1 2 2 + 1 2 , any integer. This implies that 2 − 1 2 2 2 2 + 1 2 ⇔ 0 ≤ || 1 or √4 − 1 || √4 + 1, any positive integer. So is increasing on the intervals (−11), √3 √5, −√5 −√3, √73, −3 −√7, . °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.60 ¤ CHAPTER 5 INTEGRALS (b) is concave upward on those intervals where 00 0. We differentiate 0 to find 00: 0() = cos 2 2 ⇒ 00() = −sin 2 2 2 · 2 = −sin 2 2. For 0, this is positive where (2 − 1) 2 2 2, any positive integer ⇔ 2(2 − 1) 2√, any positive integer. Since there is a factor of − in 00, the intervals of upward concavity for 0 are −2(2 + 1) −2√ , any nonnegative integer. That is, is concave upward on −√20, √22, −√6 −2, √62√2, . (c) From the graphs, we can determine that 0 cos 2 2 = 07 at ≈ 076 and ≈ 122. (d) The graphs of () and () have similar shapes, except that ’s flattens out near the origin, while ’s does not. Note that for 0, is increasing where is concave up, and is decreasing where is concave down. Similarly, is increasing where is concave down, and is decreasing where is concave up. For 0, these relationships are reversed; that is, is increasing where is concave down, and is increasing where is concave up. See Example 5.3.3 and Exercise 5.3.65 for a discussion of (). 65. Area under the curve = sinh between = 0 and = 1 is equal to 1 ⇒ 01 sinh = 1 ⇒ 1 cosh1 0 = 1 ⇒ 1(cosh − 1) = 1 ⇒ cosh − 1 = ⇒ cosh = + 1. From the graph, we get = 0 and ≈ 16161, but = 0 isn’t a solution for this problem since the curve = sinh becomes = 0 and the area under it is 0. Thus, ≈ 16161. 66. Both numerator and denominator approach 0 as → 0, so we use l’Hospital’s Rule. (Note that we are differentiating with respect to , since that is the quantity which is changing.) We also use FTC1: lim →0 ( ) = lim →0 0 −(−)2(4) √4 H = lim →0 −(−)2(4) √4 = −2(4) √4 67. Using FTC1, we differentiate both sides of the given equation, 1 () = ( − 1)2 + 1 −(), and get () = 2 + 2( − 1)2 + −() ⇒ ()(1 − −) = 2 + 2( − 1)2 ⇒ () = 2(2 − 1) 1 − − . 68. The second derivative is the derivative of the first derivative, so we’ll apply the Net Change Theorem with = 0 1200() = 12(0)0() = 0(2) − 0(1) = 5 − 2 = 3. The other information is unnecessary. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.CHAPTER 5 REVIEW ¤ 61 69. Let = () and = 0(). So 2 () 0() = 2(()) = 2 ( ( )) = [()]2 − [()]2. 70. Let () = 2 1 + 3 . Then 0(2) = lim →0 (2 + ) − (2) = lim →0 1 22+ 1 + 3 , and 0() = √1 + 3, so lim →0 1 22+ 1 + 3 = 0(2) = 1 + 23 = √9 = 3. 71. Let = 1 − . Then = −, so 01 (1 − ) = 10 ()(−) = 01 () = 01 (). 72. lim →∞ 1 1 9 + 2 9 + 3 9 + · · · + 9 = lim →∞ 1 − 0 =1 9 = 01 9 = 10 10 1 0 = 10 1 The limit is based on Riemann sums using right endpoints and subintervals of equal length. 73. The shaded region has area 01 () = 1 3. The integral 01 −1() gives the area of the unshaded region, which we know to be 1 − 1 3 = 2 3. So 01 −1() = 2 3. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.62 ¤ CHAPTER 5 INTEGRALS °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.PROBLEMS PLUS 1. Differentiating both sides of the equation sin = 02 () (using FTC1 and the Chain Rule for the right side) gives sin + cos = 2(2). Letting = 2 so that (2) = (4), we obtain sin 2 + 2 cos 2 = 4(4), so (4) = 1 4(0 + 2 · 1) = 2 . 2. The area under the curve = + 1 from = to = + 15 is given by () = +15 + 1 To find the minimum value of we’ll differentiate using FTC1 and set the derivative equal to 0. 0() = +15 + 1 = 1 + 1 + 1+15 + 1 = − 1 + 1 + 1+15 + 1 = − + 1 + + 15 + + 1 1 5 = 15 + + 1 1 5 − 1 0() = 0 ⇔ 15 + 1 + 15 − 1 = 0 ⇔ 15( + 15) + − ( + 15) = 0 ⇔ 152 + 225 − 15 = 0 multiply by 4 3 ⇔ 22 + 3 − 2 = 0 ⇔ (2 − 1)( + 2) = 0 ⇔ = 1 2 or = −2 Since 0, = 1 2 00() = − 1 ( + 15)2 + 1 2 0, so 1 2 = 122 + 1 = 1 22 + ln|| 2 12 = (2 + ln 2) − 1 8 − ln 2 = 15 8 + 2 ln 2 is the minimum value of . 3. For = 04 (−2)4 , let = − 2 so that = + 2 and = . Then = −22( + 2)4 = −22 4 + −22 24 = 0 [by 5.5.7(b)] +204 (−2)4 = 2. 4. (a) From the graph of () = 2 − 2 3 , it appears that the areas are equal; that is, the area enclosed is independent of . (b) We first find the x-intercepts of the curve, to determine the limits of integration: = 0 ⇔ 2 − 2 = 0 ⇔ = 0 or = 2. Now we integrate the function between these limits to find the enclosed area: = 02 2−3 2 = 13 2 − 1 332 0 = 13 (2)2 − 1 3(2)3 = 13 43 − 8 33 = 4 3, a constant. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. 65 NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.66 ¤ CHAPTER 5 PROBLEMS PLUS (c) The vertices of the family of parabolas seem to determine a branch of a hyperbola. (d) For a particular , the vertex is the point where the maximum occurs. We have seen that the x-intercepts are 0 and 2, so by symmetry, the maximum occurs at = , and its value is 2() − 2 3 = 1 . So we are interested in the curve consisting of all points of the form 1 , 0. This is the part of the hyperbola = 1 lying in the first quadrant. 5. () = 0() √1 + 1 3 , where () = 0cos [1 + sin(2)]. Using FTC1 and the Chain Rule (twice) we have 0() = 1 + [ 1()]3 0() = 1 + [ 1()]3 [1 + sin(cos2 )](−sin). Now 2 = 00 [1 + sin(2)] = 0, so 0 2 = √1 + 0 1 (1 + sin 0)(−1) = 1 · 1 · (−1) = −1. 6. If () = 0 2 sin(2) = 2 0 sin(2), then 0() = 2 sin(2) + 2 0 sin(2), by the Product Rule and FTC1. 7. By l’Hospital’s Rule and the Fundamental Theorem, using the notation exp() = , lim →0 0(1 − tan 2)1 H = lim →0 (1 − tan 2)1 1 = explim →0 ln(1 −tan 2) H = explim →0 −1 2sec − tan 2 2 2 = exp−12−· 102 = −2 8. The area () = 0 sin(2), and the area () = 1 2 sin(2). Since lim →0+ () = 0 = lim →0+ (), we can use l’Hospital’s Rule: lim →0+ () () H = lim →0+ sin(2) 1 2 sin(2) + 1 2 [2cos(2)] [by FTC1 and the Product Rule] H = lim →0+ 2cos(2) cos(2) − 23 sin(2) + 2cos(2) = lim →0+ 3cos(2cos( 2) − 222)sin(2) = 3 −2 0 = 23 9. () = 2 + − 2 = (− + 2)( + 1) = 0 ⇔ = 2 or = −1. () ≥ 0 for ∈ [−12] and () 0 everywhere else. The integral (2 + − 2) has a maximum on the interval where the integrand is positive, which is [−12]. So = −1, = 2. (Any larger interval gives a smaller integral since () 0 outside [−12]. Any smaller interval also gives a smaller integral since () ≥ 0 in [−1 2].) 10. This sum can be interpreted as a Riemann sum, with the right endpoints of the subintervals as sample points and with = 0, = 10,000, and () = √. So we approximate 10,000 =1 √ ≈ lim →∞ 10,000 =1 10,000 = 010000 √ = 2 3 3210 0 ,000 = 2 3(1,000,000) ≈ 666,667. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.CHAPTER 5 PROBLEMS PLUS ¤ 67 Alternate method: We can use graphical methods as follows: From the figure we see that −1 √ √ +1 √ , so 010,000 √ 10000 =1 √ 110001 √ . Since √ = 2 332 + , we get 010,000 √ = 666,6666 and 110001 √ = 2 3[(10,001)32 − 1] ≈ 666,766. Hence, 666,6666 10000 =1 √ 666,766. We can estimate the sum by averaging these bounds: 10,000 =1 ≈ 666,6666 + 666,766 2 ≈ 666,716. The actual value is about 666,71646. 11. (a) We can split the integral 0 [[]] into the sum =1 −1 [[]] . But on each of the intervals [ − 1 ) of integration, [[]] is a constant function, namely − 1. So the ith integral in the sum is equal to ( − 1)[ − ( − 1)] = ( − 1). So the original integral is equal to =1 ( − 1) = −1 =1 = ( − 1) 2 . (b) We can write [[]] = 0 [[]] − 0 [[]] . Now 0 [[]] = 0[[]] [[]] + [[]] [[]]. The first of these integrals is equal to 1 2([[]] − 1)[[]], by part (a), and since [[]] = [[]] on [[[]] ], the second integral is just [[]]( − [[]]). So 0 [[]] = 1 2([[]] − 1)[[]] + [[]]( − [[]]) = 1 2 [[]](2 − [[]] − 1) and similarly 0 [[]] = 1 2 [[]] (2 − [[]] − 1). Therefore, [[]] = 1 2 [[]] (2 − [[]] − 1) − 1 2 [[]] (2 − [[]] − 1). 12. By FTC1, 01sin 1 + 4 = 1sin 1 + 4 . Again using FTC1, 2 2 01sin 1 + 4 = 1sin 1 + 4 = 1 + sin4 cos. 13. Let () = 0 () = + 2 2 + 3 3 + 44 0 = + 2 2 + 3 3 + 44. Then (0) = 0, and (1) = 0 by the given condition, + 2 + 3 + 4 = 0. Also, 0() = () = + + 2 + 3 by FTC1. By Rolle’s Theorem, applied to on [01], there is a number in (01) such that 0() = 0, that is, such that () = 0. Thus, the equation () = 0 has a root between 0 and 1. More generally, if () = 0 + 1 + 22 + · · · + and if 0 + 1 2 + 2 3 + · · · + + 1 = 0 then the equation () = 0 has a root between 0 and 1 The proof is the same as before: Let () = 0 () = 0 + 21 2 + 32 3 + · · · + + 1 . Then (0) = (1) = 0 and 0() = (). By Rolle’s Theorem applied to on [0 1], there is a number in (01) such that 0() = 0, that is, such that () = 0. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.68 ¤ CHAPTER 5 PROBLEMS PLUS 14. Let be the distance between the center of the disk and the surface of the liquid. The wetted circular region has area 2 − 2 while the unexposed wetted region (shaded in the diagram) has area 2 √2 − 2 , so the exposed wetted region has area () = 2 − 2 − 2 √2 − 2 , 0 ≤ ≤ . By FTC1, we have 0() = −2 + 2√2 − 2. Now 0() 0 ⇒ −2 + 2√2 − 2 0 ⇒ √2 − 2 ⇒ 2 − 2 22 ⇒ 2 22 + 2 ⇒ 2 2(2 + 1) ⇒ 2 2 2 + 1 ⇒ √2 + 1, and we’ll call this value *. Since 0() 0 for 0 * and 0() 0 for * , we have an absolute maximum when = *. 15. Note that 0 0 () = 0 () by FTC1, while 0 ()( − ) = 0 () − 0 () = 0 () + () − () = 0 () Hence, 0 ()( − ) = 0 0 () + . Setting = 0 gives = 0. 16. The parabola = 4 − 2 and the line = + 2 intersect when 4 − 2 = + 2 ⇔ 2 + − 2 = 0 ⇔ ( + 2)( − 1) = 0 ⇔ = −2 or 1. So the point A is (−20) and B is (13). The slope of the line = + 2 is 1 and the slope of the parabola = 4 − 2 at -coordinate is −2. These slopes are equal when = − 1 2, so the point C is − 1 2 15 4 . The area 1 of the parabolic segment is the area under the parabola from = −2 to = 1, minus the area under the line = + 2 from −2 to 1. Thus, 1 = −12(4 − 2) − −12( + 2) = 4 − 1 331 −2 − 1 22 + 21 −2 = 4 − 1 3 − −8 + 8 3 − 1 2 + 2 − (2 − 4) = 9 − 9 2 = 9 2 The area 2 of the inscribed triangle is the area under the line segment AC plus the area under the line segment CB minus the area under the line segment AB. The line through A and C has slope 154 − 0 −12 + 2 = 5 2 and equation − 0 = 5 2( + 2), or = 5 2 + 5. The line through C and B has slope 3 − 154 1 + 12 = − 1 2 and equation − 3 = − 1 2( − 1), or = − 1 2 + 7 2. Thus, 2 = −−212 5 2 + 5 + −112 − 1 2 + 7 2 − −12( + 2) = 5 42 + 5− −1 22 + − 1 42 + 7 21 −12 − 9 2 = 16 5 − 5 2 − (5 − 10) + − 1 4 + 7 2 − − 16 1 − 7 4 − 9 2 = 45 16 + 81 16 − 72 16 = 54 16 = 27 8 Archimedes’ result states that 1 = 4 32 which is verified in this case since 4 3 · 27 8 = 9 2. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.CHAPTER 5 PROBLEMS PLUS ¤ 69 17. Let be the nonzero -intercept so that the parabola has equation () = ( − ), or = 2 − , where 0. The area under the parabola is = 0 ( − ) = 0 (2 − ) = 1 3 3 − 1 2 2 0 = 1 3 3 − 1 2 3 = − 1 6 3 The point ( ) is on the parabola, so () = ⇒ = ( − ) ⇒ = ( − ). Substituting for in gives () = −6 · −3 ⇒ 0() = − 6 · ( − )(32) − 3(−1) ( − )2 = − 6 · 2[3( − ) + ] ( − )2 = − 2(3 − 2) 6( − )2 Now 0 = 0 ⇒ = 3 2 . Since 0() 0 for 3 2 and 0() 0 for 3 2 , so has an absolute minimum when = 3 2 . Substituting for in gives us = − 3 2 = − 2 2 , so () = −22 − 3 2 , or () = −2 2 2 + 3 . Note that the vertex of the parabola is 3 4 9 8 and the minimal area under the parabola is 3 2 = 9 8 . 18. We restrict our attention to the triangle shown. A point in this triangle is closer to the side shown than to any other side, so if we find the area of the region consisting of all points in the triangle that are closer to the center than to that side, we can multiply this area by 4 to find the total area. We find the equation of the set of points which are equidistant from the center and the side: the distance of the point ( ) from the side is 1 − , and its distance from the center is 2 + 2. So the distances are equal if 2 + 2 = 1 − ⇔ 2 + 2 = 1 − 2 + 2 ⇔ = 1 2(1 − 2). Note that the area we are interested in is equal to the area of a triangle plus a crescent-shaped area. To find these areas, we have to find the -coordinate of the horizontal line separating them. From the diagram, 1 − = √2 ⇔ = 1 1 + √2 = √2 − 1. We calculate the areas in terms of , and substitute afterward. The area of the triangle is 1 2(2)() = 2, and the area of the crescent-shaped section is − 1 2(1 − 2) − = 20 1 2 − − 1 2 2 = 2 1 2 − − 1 6 3 0 = − 22 − 1 3 3. So the area of the whole region is 4 − 22 − 1 3 3 + 2 = 41 − − 1 3 2 = 4√2 − 11 − √2 − 1 − 1 3 √2 − 12 = 4√2 − 11 − 1 3 √2 = 4 3 4√2 − 5 °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.70 ¤ CHAPTER 5 PROBLEMS PLUS 19. lim →∞√ √1 + 1 + √ √1 + 2 + · · · + √ √1 + = lim →∞ 1 + 1 + + 2 + · · · + + = lim →∞ 1 1 + 1 1 + 1 + 2 1 + · · · + √1 + 1 1 = lim →∞ 1 =1 where () = √1 + 1 = 01 √1 + 1 = 2√1 + 1 0 = 2√2 − 1 20. Note that the graphs of ( − )2 and [( − ) − 2]2 intersect when | − | = | − − 2| ⇔ − = − − 2 ⇔ = + 1. The integration will proceed differently depending on the value of . Case 1: −2 ≤ −1 In this case, () = ( − − 2)2 for ∈ [01], so () = 01( − − 2)2 = 1 3( − − 2)31 0 = 1 3(− − 1)3 − (− − 2)3 = 1 3 (32 + 9 + 7) = 2 + 3 + 7 3 = + 3 22 + 12 1 This is a parabola; its maximum value for −2 ≤ −1 is (−2) = 1 3, and its minimum value is − 3 2 = 12 1 . Case 2: −1 ≤ 0 In this case, () = ( − )2 if 0 ≤ ≤ + 1 ( − − 2)2 if + 1 ≤ 1 Therefore, () = 01 () = 0+1( − )2 + 1+1( − − 2)2 = 1 3 ( − )3 0+1 + 1 3( − − 2)31 +1 = 1 31 + 3 + (− − 1)3 − (−1) = −2 − + 1 3 = − + 1 22 + 12 7 Again, this is a parabola, whose maximum value for −1 ≤ 0 is − 1 2 = 12 7 , and whose minimum value on this -interval is (−1) = 1 3. °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.CHAPTER 5 PROBLEMS PLUS ¤ 71 Case 3: 0 ≤ ≤ 2 In this case, () = ( − )2 for ∈ [01], so () = 01( − )2 = 1 3 ( − )31 0 = 1 3 (1 − )3 − (−)3 = 2 − + 1 3 = − 1 2 2 + 12 1 This parabola has a maximum value of (2) = 7 3 and a minimum value of 1 2 = 12 1 . We conclude that () has an absolute maximum value of (2) = 7 3, and absolute minimum values of − 3 2 = 1 2 = 12 1 . °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved.72 ¤ CHAPTER 5 PROBLEMS PLUS °c 2016 Cengage Learning. All Rights Reserved. May not be scanned, copied, or duplicated, or posted to a publicly accessible website, in whole or in part. NOT FOR SALE INSTRUCTOR USE ONLY © Cengage Learning. All Rights Reserved. [Show More]
Last updated: 1 year ago
Preview 1 out of 1 pages
Instant download

Buy this document to get the full access instantly
Instant Download Access after purchase
Add to cartInstant download
Reviews( 0 )
Document information
Connected school, study & course
About the document
Uploaded On
Jan 06, 2020
Number of pages
1
Written in
Additional information
This document has been written for:
Uploaded
Jan 06, 2020
Downloads
0
Views
52