College Algebra Milestone 5 - All Answers ACCURATE QUESTIONS AND ANSWERS. GRADED A. 100% PASS RATE

Document Content and Description Below

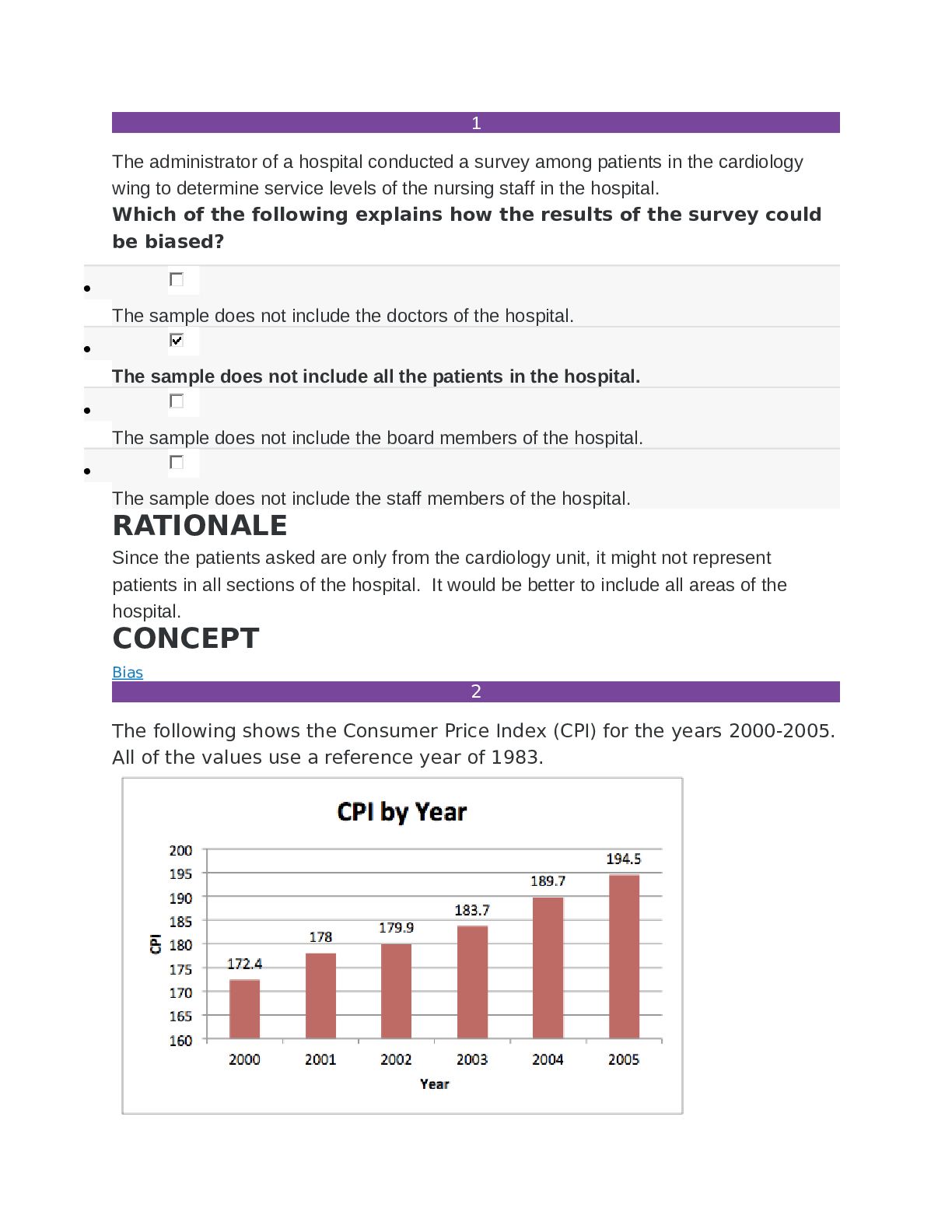
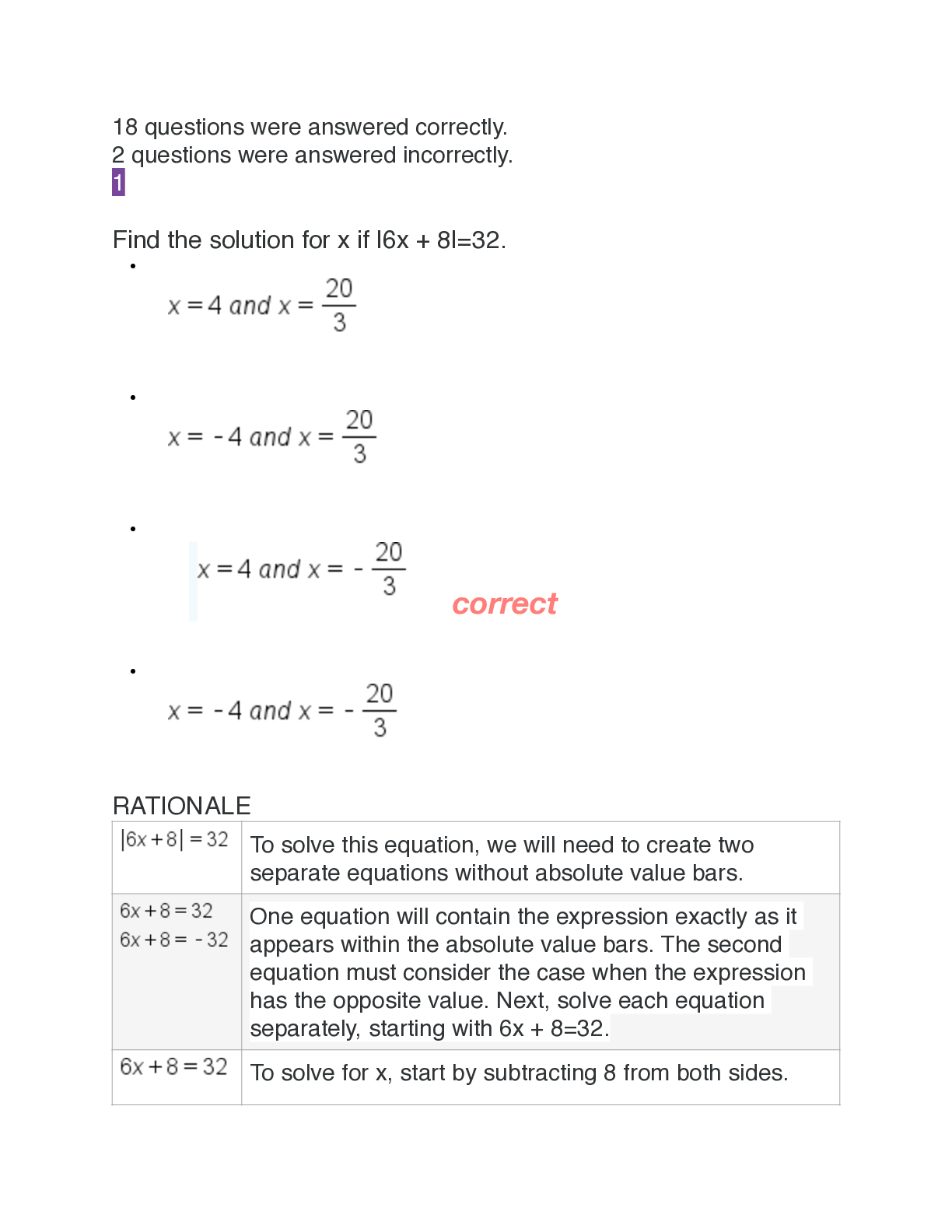
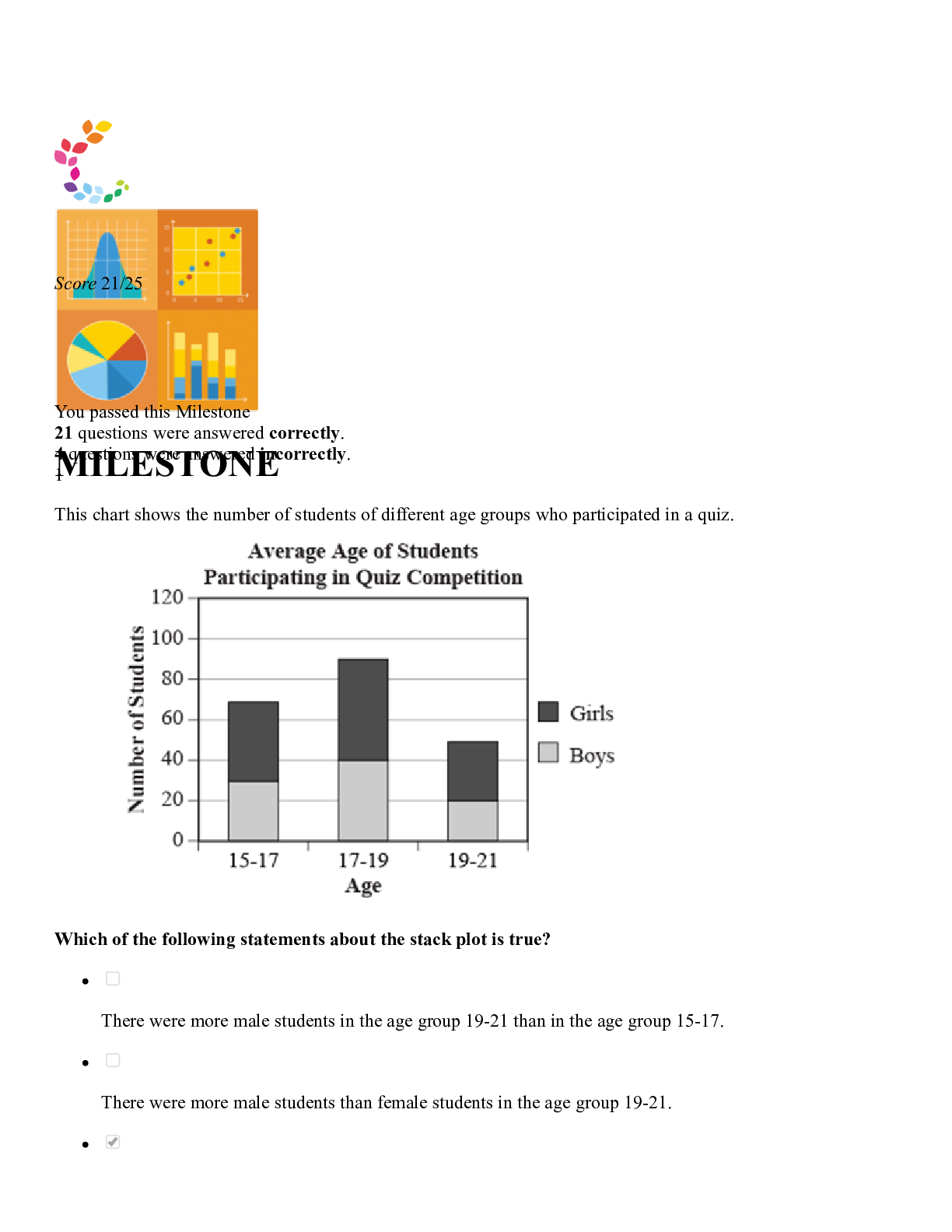
You passed this Milestone 21 questions were answered correctly. 1 question was answered incorrectly. 1 Consider the function . What are the domain and range of this function? • • correct ... • • RATIONALE A Square root function has the domain restriction that the radicand (the value underneath the radical) cannot be negative. To find the specific domain, construct an inequality showing that the radicand must be greater than or equal to zero. CONCEPT Finding the Domain and Range of Functions 2 Kevin examines the following data, which shows the balance in an investment account. This tell us that must be greater than or equal to . In other words, must be less than or equal to . We can write this inequality in the other direction. This is the domain of the function, which means all values must be less than or equal to . To find the range, consider the fact that it is not possible for the input of the function to be a negative number. For all x-values less than or equal to , the function will have non-negative values for y that only get bigger and bigger as x increases. The range is all values greater than or equal to zero. The expression under the radical, , must be greater than or equal to zero. To solve this inequality, add to both sides to undo the subtraction of . Year Balance 1 $5,000.00 2 $5,100.00 3 $5,202.00 4 $5,306.04 5 $5,412.16 What is the formula for the geometric sequence represented by the data above? • • • • correct RATIONALE The first term, which is , so will be replaced by in the formula. Next, let's find , the common ratio. This is the general formula for a geometric sequence. We will use information in the table to find values for and . Let's start with finding , the value of the first term. CONCEPT Introduction to Geometric Sequences 3 Find the solution for in the equation . • • • • correct RATIONALE This is the formula for the geometric sequence. To find , take the value of any term, and divide it by the value of the previous term to find the common ratio. For example, so . Finally, plug in values for and into the geometric sequence formula. divided by is equal to . To undo the variable exponent, apply a logarithm to both sides. To solve this equation, begin by dividing both sides by to cancel the coefficient in front of the exponential. CONCEPT Solving Exponential Equations using Logarithms 4 Select the graph of . If you apply a log to one side, you must apply a log to other side. Next, apply the Power Property of Logs which states that exponents inside a logarithm can be expressed as outside scalar multiples of the logarithm. This is the solution to the equation . [Show More]

Last updated: 1 year ago

Preview 1 out of 41 pages